K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên

9 tháng 5 2017
f(x)=sin3x , f '(x) = 3cos3x .... f ''(x) =-3.3.sin(3x)
suy ra f ''(x) = -9sin(3x) ....
f ''(\(\dfrac{\pi}{2}\)) = -9.sin(3.\(\dfrac{-\pi}{2}\)) =-9
f ''(0\(\)) = -9.sin(3.0\(\)) =0
f ''(\(\dfrac{\pi}{18}\)) = -9.sin(3.\(\dfrac{\pi}{18}\))=\(\dfrac{-9}{2}\)..ok nha

QD
4 tháng 4 2017
a) Ta có f'(x) = 6(x + 10)'.(x + 10)5
\(=6.\left(x+10\right)^5\)
f"(x) = 6.5(x + 10)'.(x + 10)4 = 30.(x + 10)4.
=> f''(2) = 30.(2 + 10)4 = 622 080.
b) Ta có f'(x) = (3x)'.cos3x = 3cos3x,
f"(x) = 3.[-(3x)'.sin3x] = -9sin3x.
Suy ra f"\(\dfrac{-\pi}{2}\) = -9sin\(\dfrac{-3\pi}{2}\) = -9;
f"(0) = -9sin0 = 0;
f"\(\dfrac{\pi}{18}\) = -9sin\(\dfrac{\pi}{6}\) = \(\dfrac{-9}{2}\).
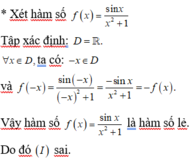
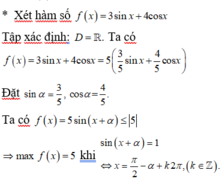

f''(-π/2) = -9, f''(0) = 0, f''(π/18) = -9/2