Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Câu 1: Xét trên miền [1;4]
Do \(f\left(x\right)\) đồng biến \(\Rightarrow f'\left(x\right)\ge0\)
\(x\left(1+2f\left(x\right)\right)=\left[f'\left(x\right)\right]^2\Leftrightarrow x=\frac{\left[f'\left(x\right)\right]^2}{1+2f\left(x\right)}\Leftrightarrow\frac{f'\left(x\right)}{\sqrt{1+2f\left(x\right)}}=\sqrt{x}\)
Lấy nguyên hàm 2 vế:
\(\int\frac{f'\left(x\right)dx}{\sqrt{1+2f\left(x\right)}}=\int\sqrt{x}dx\Leftrightarrow\int\left(1+2f\left(x\right)\right)^{-\frac{1}{2}}d\left(f\left(x\right)\right)=\int x^{\frac{1}{2}}dx\)
\(\Leftrightarrow\sqrt{1+2f\left(x\right)}=\frac{2}{3}x\sqrt{x}+C\)
Do \(f\left(1\right)=\frac{3}{2}\Rightarrow\sqrt{1+2.\frac{3}{2}}=\frac{2}{3}.1\sqrt{1}+C\Rightarrow C=\frac{4}{3}\)
\(\Rightarrow\sqrt{1+2f\left(x\right)}=\frac{2}{3}x\sqrt{x}+\frac{4}{3}\)
Đến đây có thể bình phương chuyển vế tìm hàm \(f\left(x\right)\) chính xác, nhưng dài, thay luôn \(x=4\) vào ta được:
\(\sqrt{1+2f\left(4\right)}=\frac{2}{3}4.\sqrt{4}+\frac{4}{3}=\frac{20}{3}\Rightarrow f\left(4\right)=\frac{\left(\frac{20}{3}\right)^2-1}{2}=\frac{391}{18}\)
Câu 2:
Diện tích hình phẳng cần tìm là hai miền đối xứng qua Oy nên ta chỉ cần tính trên miền \(x\ge0\)
Hoành độ giao điểm: \(sinx=x-\pi\Rightarrow x=\pi\)
\(S=2\int\limits^{\pi}_0\left(sinx-x+\pi\right)dx=4+\pi^2\Rightarrow\left\{{}\begin{matrix}a=4\\b=1\end{matrix}\right.\)
\(\Rightarrow2a+b^3=9\)

a) f(x) = 2x3 – 3x2 – 12x + 1 ⇒ f’(x) = 6x2 – 6x – 12
f’(x) = 0 ⇔ x ∈ {-1, 2}
So sánh các giá trị:
f(x) = -3; f(-1) = 8;
f(2) = -19, f(52)=−332f(52)=−332
Suy ra:
maxx∈[−2,52]f(x)=f(−1)=8minx∈[−2,52]f(x)=f(2)=−19maxx∈[−2,52]f(x)=f(−1)=8minx∈[−2,52]f(x)=f(2)=−19
b) f(x) = x2 lnx ⇒ f’(x)= 2xlnx + x > 0, ∀ x ∈ [1, e] nên f(x) đồng biến.
Do đó:
maxx∈[1,e]f(x)=f(e)=e2minx∈[1,e]f(x)=f(1)=0maxx∈[1,e]f(x)=f(e)=e2minx∈[1,e]f(x)=f(1)=0
c) f(x) = f(x) = xe-x ⇒ f’(x)= e-x – xe-x = (1 – x)e-x nên:
f’(x) = 0 ⇔ x = 1, f’(x) > 0, ∀x ∈ (0, 1) và f’(x) < 0, ∀x ∈ (1, +∞)
nên:
maxx∈[0,+∞)f(x)=f(1)=1emaxx∈[0,+∞)f(x)=f(1)=1e
Ngoài ra f(x) = xe-x > 0, ∀ x ∈ (0, +∞) và f(0) = 0 suy ra
maxx∈[0,+∞)f(x)=f(0)=0maxx∈[0,+∞)f(x)=f(0)=0
d) f(x) = 2sinx + sin2x ⇒ f’(x)= 2cosx + 2cos2x
f’(x) = 0 ⇔ cos 2x = -cosx ⇔ 2x = ± (π – x) + k2π
⇔ x∈{−π+k2π;π3+k2π3}x∈{−π+k2π;π3+k2π3}
Trong khoảng [0,3π2][0,3π2] , phương trình f’(x) = 0 chỉ có hai nghiệm là x1=π3;x2=πx1=π3;x2=π
So sánh bốn giá trị : f(0) = 0; f(π3)=3√32;f(π)=0;f(3π2)=−2f(π3)=332;f(π)=0;f(3π2)=−2
Suy ra:
maxx∈[0,3π2]f(x)=f(π3)=3√32minx∈[0,3π2]f(x)=f(3π2)=−2

Nhìn 2 vế của hàm số thì có vẻ ta cần phân tích biểu thức vế trái về dạng \(\left[f\left(x\right).u\left(x\right)\right]'=f\left(x\right).u'\left(x\right)+u\left(x\right).f'\left(x\right)\), ta cần tìm thằng \(u\left(x\right)\) này
Biến đổi 1 chút xíu: \(\frac{\left[f\left(x\right).u\left(x\right)\right]'}{u\left(x\right)}=\frac{u'\left(x\right)}{u\left(x\right)}f\left(x\right)+f'\left(x\right)\) (1) hay vào bài toán:
\(\left(\frac{x+2}{x+1}\right)f\left(x\right)+f'\left(x\right)=\frac{e^x}{x+1}\) (2)
Nhìn (1) và (2) thì rõ ràng ta thấy \(\frac{u'\left(x\right)}{u\left(x\right)}=\frac{x+2}{x+1}=1+\frac{1}{x+1}\)
Lấy nguyên hàm 2 vế:
\(ln\left(u\left(x\right)\right)=\int\left(1+\frac{1}{x+1}\right)dx=x+ln\left(x+1\right)\)
\(\Rightarrow u\left(x\right)=e^{x+ln\left(x+1\right)}=e^x.e^{ln\left(x+1\right)}=e^x.\left(x+1\right)\)
Vậy ta đã tìm xong hàm \(u\left(x\right)\)
Vế trái bây giờ cần biến đổi về dạng:
\(\left[f\left(x\right).e^x\left(x+1\right)\right]'=e^x\left(x+2\right).f\left(x\right)+f'\left(x\right).e^x\left(x+1\right).f'\left(x\right)\)
Để tạo thành điều này, ta cần nhân \(e^x\) vào 2 vế của biểu thức ban đầu:
\(e^x\left(x+2\right)f\left(x\right)+e^x\left(x+1\right)f'\left(x\right)=e^{2x}\)
\(\Leftrightarrow\left[f\left(x\right).e^x.\left(x+1\right)\right]'=e^{2x}\)
Lấy nguyên hàm 2 vế:
\(f\left(x\right).e^x\left(x+1\right)=\int e^{2x}dx=\frac{1}{2}e^{2x}+C\)
Do \(f\left(0\right)=\frac{1}{2}\Rightarrow f\left(0\right).e^0=\frac{1}{2}e^0+C\Rightarrow C=0\)
Vậy \(f\left(x\right).e^x\left(x+1\right)=\frac{1}{2}e^{2x}\Rightarrow f\left(x\right)=\frac{1}{2}\frac{e^{2x}}{e^x\left(x+1\right)}=\frac{e^x}{2\left(x+1\right)}\)
\(\Rightarrow f\left(2\right)=\frac{e^2}{2\left(2+1\right)}=\frac{e^2}{6}\)

Ta có \(f'\left(x\right)=3ax^2+2bx+c;f"\left(x\right)=6ax+2b\)
Hàm số \(f\left(x\right)\) đạt cực tiểu tại \(x=0\) khi và chỉ khi
\(\begin{cases}f'\left(0\right)=0\\f"\left(0\right)>0\end{cases}\)\(\Leftrightarrow\begin{cases}c=0\\2b>0\end{cases}\)\(\Leftrightarrow\begin{cases}c=0\\b>0\end{cases}\left(1\right)\)
Hàm số \(f\left(x\right)\) đạt cực đại tại \(x=1\) khi và chỉ khi \(\begin{cases}f'\left(1\right)=0\\f"\left(1\right)< 0\end{cases}\)\(\Leftrightarrow\begin{cases}3a+2b+c=0\\6a+2b< 0\end{cases}\)
\(\begin{cases}f\left(0\right)=0\\f\left(1\right)=1\end{cases}\)\(\Leftrightarrow\begin{cases}d=0\\a+b+c+d=1\end{cases}\) \(\Leftrightarrow\begin{cases}d=0\\a+b+c+d=1\end{cases}\) (3)
Từ (1), (2), (3) suy ra \(a=-2;b=3;c=0;d=0\)
Kiểm tra lại \(f\left(x\right)=-2x^3+3x^2\)
Ta có \(f'\left(x\right)=-6x^2+6x;f"\left(x\right)=-12x+6\)
\(f"\left(0\right)=6>0\), hàm số đạt cực tiểu tại \(x=0\)
\(f"\left(1\right)=-6< 0\), hàm số đạt cực đại tại \(x=1\)
Vậy \(a=-2;b=3;c=0;d=0\)

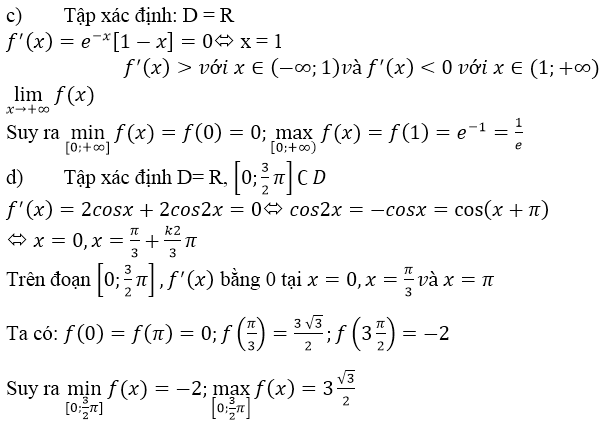
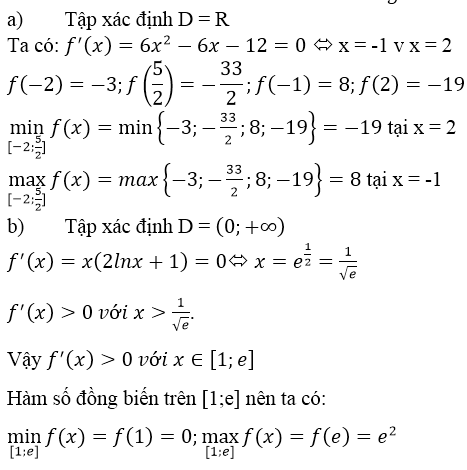
Chọn D.
Đặt t = 2x => dt = 2dx, Đổi cận x = 0 <=> t = 0, x = 1 <=> t = 2
I = 1 4 ∫ 0 2 t f ' ( t ) d t
Đặt u = t ⇒ d u = d t d v = f ' ( t ) d t ⇒ v = f ( t )
I = 1 4 t f ( t ) 2 0 - ∫ 0 2 f ( t ) d t = 1 4 ( 2 f ( 2 ) - 0 f ( 0 ) - 4 ) = 7