Cho biểu thức: A = a 2

DV

Dung Vu

10 tháng 11 2021

2 a. rút gọn biểu C = $\dfrac{2x^{\text{2}}-x}{\text{x }-1}+\dfrac{x+1}{1-x}+\dfrac{2-x^2}{x-1}$ b. Rút gọn biểu thức D = $\left(\dfrac{1}{a-\sqrt{a}}+\dfrac{1}{\sqrt{\text{a}}-1}\right):\dfrac{\sqrt{\text{a}}+1}{a-2\sqrt{a}+1}$Vậy khi rút gọn một biểu thức hửu tỉ và một biểu thức chứa căn có tìm điều kiện xác định...

Đọc tiếp

#Toán lớp 9

1

NH

Nguyễn Hoàng Minh

10 tháng 11 2021

$a,C=\dfrac{2x^2-x-x-1+2-x^2}{x-1}\left(x\ne1\right)\\ C=\dfrac{x^2-2x+1}{x-1}=\dfrac{\left(x-1\right)^2}{x-1}=x-1\\ b,D=\dfrac{1+\sqrt{a}}{\sqrt{a}\left(\sqrt{a}-1\right)}\cdot\dfrac{\left(\sqrt{a}-1\right)^2}{\sqrt{a}+1}\left(a>0;a\ne1\right)\\ D=\dfrac{\sqrt{a}-1}{\sqrt{a}}$

Có

Đúng(1)

NP

Nguyễn Phương Diệp Thy

27 tháng 10 2021

1. Cho biểu thức : Q = ( √x + 2 / x +2 √x + 1 - √x - / x -1) ( x+ √x)a) Rút gọn biểu thức Qb) Tìm các gtri nguyên x dể Q nhận gtri nguyên 2. Cho biểu thức : A= ( 1/ √x +2 + 1/ √x +2 + 1/ √x -2 ) ( √x -2 /x a) Tìm đk xác định và rút gọn Ab) Tìm tất cả các gtri của x để A > 1/2MÌNH CẦN GẤP TRONG TỐI NI...

Đọc tiếp

#Toán lớp 9

1

NL

Nguyễn Lê Phước Thịnh

27 tháng 10 2021

Bài 1:

a: $Q=\left(\dfrac{\sqrt{x}+2}{x+2\sqrt{x}+1}-\dfrac{\sqrt{x}-2}{x-1}\right)\left(x+\sqrt{x}\right)$

$=\dfrac{x+\sqrt{x}-2-x+\sqrt{x}+2}{\left(\sqrt{x}+1\right)^2\cdot\left(\sqrt{x}-1\right)}\cdot\sqrt{x}\left(\sqrt{x}+1\right)$

$=\dfrac{2x}{x-1}$

Đúng(0)

NC

Nguyễn Châu Mỹ Linh

5 tháng 5 2021

Câu 1: Rút gọn biểu thức: $A=\left(\dfrac{1-a\sqrt{a}}{1-\sqrt{a}}+\sqrt{a}\right)\left(\dfrac{1-\sqrt{a}}{1-a}\right)^2$(với a $\ge$ 0;a $\ne$1)Câu 2: Rút gọn biểu thức: $M=\left(\dfrac{a+\sqrt{a}}{\sqrt{a}+1}+1\right)\left(1+\dfrac{a-\sqrt{a}}{1-\sqrt{a}}\right)$(với a$\ge$0;...

Đọc tiếp

#Toán lớp 9

2

NL

Nguyễn Lê Phước Thịnh

5 tháng 5 2021

Câu 2:

Ta có: $M=\left(\dfrac{a+\sqrt{a}}{\sqrt{a}+1}+1\right)\left(1+\dfrac{a-\sqrt{a}}{1-\sqrt{a}}\right)$

$=\left(\dfrac{\sqrt{a}\left(\sqrt{a}+1\right)}{\sqrt{a}+1}+1\right)\left(1-\dfrac{\sqrt{a}\left(\sqrt{a}-1\right)}{\sqrt{a}-1}\right)$

$=\left(1+\sqrt{a}\right)\left(1-\sqrt{a}\right)$

$=1-a$

Đúng(1)

NL

Nguyễn Lê Phước Thịnh

5 tháng 5 2021

Câu 1:

Ta có: $A=\left(\dfrac{1-a\sqrt{a}}{1-\sqrt{a}}+\sqrt{a}\right)\left(\dfrac{1-\sqrt{a}}{1-a}\right)^2$

$=\left(\dfrac{\left(1-\sqrt{a}\right)\left(1+\sqrt{a}+a\right)}{1-\sqrt{a}}+\sqrt{a}\right)\left(\dfrac{1}{\sqrt{a}+1}\right)^2$

$=\left(\sqrt{a}+1\right)^2\cdot\dfrac{1}{\left(\sqrt{a}+1\right)^2}$

$=1$

Đúng(1)

TK

trương khoa

21 tháng 5 2021

Cho biểu thức A=$\dfrac{2a^2+4}{1-a^2}-\dfrac{1}{1+\sqrt{a}}-\dfrac{1}{1-\sqrt{a}}$( với a≥0;a≠1)

Rút gọn biểu thức A

NHờ mọi người giúp ạ

#Toán lớp 9

1

AH

Akai Haruma

Giáo viên

21 tháng 5 2021

Lời giải:

$A=\frac{2a^2+4}{(1-a)(1+a)}-\frac{1-\sqrt{a}+1+\sqrt{a}}{(1+\sqrt{a})(1-\sqrt{a})}=\frac{2a^2+4}{(1-a)(1+a)}-\frac{2}{1-a}$

$=\frac{2a^2+4}{(1-a)(1+a)}-\frac{2(1+a)}{(1-a)(1+a)}=\frac{2a^2-2a+2}{(1-a)(1+a)}=\frac{2(a^2-a+1)}{1-a^2}$

Đúng(1)

TK

trương khoa

22 tháng 5 2021

hình như sai rồi thầy ơi

Đúng(0)

N

NinhTuấnMinh

23 tháng 2 2022

với a > 1, cho biểu thức $P=\left(\dfrac{2}{\sqrt{a+1}}+\sqrt{a-1}\right):\left(\dfrac{2}{\sqrt{a^2-1}}+1\right)$
rút gọn biểu thức P và tim giá trị của a để P=2

#Toán lớp 9

1

TC

Trên con đường thành công không có....

23 tháng 2 2022

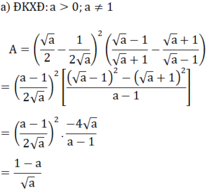
$P=\left(\dfrac{2+\sqrt{a+1}\sqrt{a-1}}{\sqrt{a+1}}\right):\left(\dfrac{2+\sqrt{a+1}\sqrt{a-1}}{\sqrt{a+1}\sqrt{a-1}}\right)$

$=\dfrac{2+\sqrt{a+1}\sqrt{a-1}}{\sqrt{a+1}}.\dfrac{\sqrt{a+1}\sqrt{a-1}}{2+\sqrt{a+1}\sqrt{a-1}}$

$=\sqrt{a-1}$

P=2$\Rightarrow\sqrt{a-1}=2\Leftrightarrow a-1=4\Leftrightarrow a=5\left(tm\right)$

Đúng(2)

LB

Linh Bùi

21 tháng 2 2021

Câu 2: CHo biểu thức:

$B=\left(\dfrac{1}{a-\sqrt{a}}+\dfrac{1}{\sqrt{a}-1}\right):\dfrac{a-1}{a-2\sqrt{a}+1}$ ( với a> 0, a ≠ 1)

a) Rút gọn biểu thức B

b) Tính GT của B khi A = 3-2$\sqrt{2}$

(mink đag cần gấp)

#Toán lớp 9

1

NL

Nguyễn Lê Phước Thịnh

21 tháng 2 2021

a) Ta có: $B=\left(\dfrac{1}{a-\sqrt{a}}+\dfrac{1}{\sqrt{a}-1}\right):\dfrac{a-1}{a-2\sqrt{a}+1}$

$=\left(\dfrac{1}{\sqrt{a}\left(\sqrt{a}-1\right)}+\dfrac{\sqrt{a}}{\sqrt{a}\left(\sqrt{a}-1\right)}\right):\dfrac{\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}{\left(\sqrt{a}-1\right)^2}$

$=\dfrac{\sqrt{a}+1}{\sqrt{a}\left(\sqrt{a}-1\right)}\cdot\dfrac{\sqrt{a}-1}{\sqrt{a}+1}$

$=\dfrac{1}{\sqrt{a}}$

b) Thay $a=3-2\sqrt{2}$ vào biểu thức $B=\dfrac{1}{\sqrt{a}}$, ta được:

$B=\dfrac{1}{\sqrt{3-2\sqrt{2}}}=\dfrac{1}{\sqrt{2}-1}=\sqrt{2}+1$

Vậy: Khi $a=3-2\sqrt{2}$ thì $B=\sqrt{2}+1$

Đúng(1)

KG

KYAN Gaming

26 tháng 7 2021

1. Cho biểu thức: A=$\left[\dfrac{a+3\sqrt{a}+2}{\left(\sqrt{a}+2\right)\left(\sqrt{a}-1\right)}-\dfrac{a+\sqrt{a}}{a-1}\right]:\left(\dfrac{1}{\sqrt{a}+1}+\dfrac{1}{\sqrt{a}-1}\right)$

Rút gọn biểu thức trên

#Toán lớp 9

2

LD

Lê Đình Hiếu

26 tháng 7 2021

A=$\left[\dfrac{\left(\sqrt{a}+2\right)\left(\sqrt{a}+1\right)}{\left(a-1\right)\left(\sqrt{a}+2\right)}-\dfrac{\left(a+\sqrt{a}\right)}{\left(a-1\right)}\right]$::::::::$\left(\dfrac{\left(\sqrt{a}-1+\sqrt{a}+1\right)}{a-1}\right)$

=$\left[\dfrac{1}{\sqrt{a}-1}\right]:\left(\dfrac{2\sqrt{a}}{a-1}\right)$=$\dfrac{\sqrt{a}-1}{2\sqrt{a}}$

=$\dfrac{a^2+a\sqrt{a}+11a+6}{2\sqrt{a}\left(\sqrt{a}+2\right)}$

Đúng(1)

NL

Nguyễn Lê Phước Thịnh

26 tháng 7 2021

Ta có: $A=\left(\dfrac{a+3\sqrt{a}+2}{\left(\sqrt{a}+2\right)\left(\sqrt{a}-1\right)}-\dfrac{a+\sqrt{a}}{a-1}\right):\left(\dfrac{1}{\sqrt{a}+1}+\dfrac{1}{\sqrt{a}-1}\right)$

$=\dfrac{\sqrt{a}+1-\sqrt{a}}{\sqrt{a}-1}:\dfrac{\sqrt{a}-1+\sqrt{a}+1}{\left(\sqrt{a}+1\right)\left(\sqrt{a}-1\right)}$

$=\dfrac{1}{\sqrt{a}-1}\cdot\dfrac{\left(\sqrt{a}+1\right)\left(\sqrt{a}-1\right)}{2\sqrt{a}}$

$=\dfrac{\sqrt{a}+1}{2\sqrt{a}}$

Đúng(1)

TT

Tôm Tôm

27 tháng 10 2021

Cho biểu thức K= ( √a/√a-1 - 1/a-√a) : ( 1/√a+1 + 2/a-1)

a) rút gọn K

b) tính giá trị của K khi a=3+2√2

c) tìm a sao cho K<0

#Toán lớp 9

1

NL

Nguyễn Lê Phước Thịnh

27 tháng 10 2021

a: $K=\left(\dfrac{\sqrt{a}}{\sqrt{a}-1}-\dfrac{1}{a-\sqrt{a}}\right):\left(\dfrac{1}{\sqrt{a}+1}+\dfrac{2}{a-1}\right)$

$=\dfrac{a-1}{\sqrt{a}\left(\sqrt{a}-1\right)}:\dfrac{\sqrt{a}-1+2}{a-1}$

$=\dfrac{a-1}{\sqrt{a}}$

Đúng(0)

HT

Hoài Thu Vũ

5 tháng 8 2023

Cho biểu thức A= $(\frac{1}{\sqrt{x-1}}$+$\frac{1}{\sqrt{x-1}})^{2}$. $\frac{x^{2}-1}{2}$- $\sqrt{x^2-1}$
Rút gọn biểu thức A

#Toán lớp 9

1

H9

HT.Phong (9A5)

CTVHS

5 tháng 8 2023

$A=\left(\dfrac{1}{\sqrt{x-1}}+\dfrac{1}{\sqrt{x-1}}\right)^2\cdot\dfrac{x^2-1}{2}-\sqrt{x^2-1}$ (ĐK: $x>1$)

$A=\left(\dfrac{2}{\sqrt{x-1}}\right)^2\cdot\dfrac{x^2-1}{2}-\sqrt{x^2-1}$

$A=\dfrac{4}{x-1}\cdot\dfrac{\left(x+1\right)\left(x-1\right)}{2}-\sqrt{x^2-1}$

$A=2\left(x+1\right)-\sqrt{\left(x+1\right)\left(x-1\right)}$

$A=\sqrt{x+1}\left(2\sqrt{x+1}-\sqrt{x-1}\right)$

Đúng(1)

HT

Hoài Thu Vũ

5 tháng 8 2023

Cho biểu thức A= $(\frac{1}{\sqrt{x-1}}$+$\frac{1}{\sqrt{x+1}})^{2}$. $\frac{x^{2}-1}{2}$- $\sqrt{x^2-1}$
Rút gọn biểu thức A

#Toán lớp 9

1

HQ

Hà Quang Minh

Giáo viên

5 tháng 8 2023

$A=\left(\dfrac{1}{\sqrt{x-1}}+\dfrac{1}{\sqrt{x+1}}\right)^2\cdot\dfrac{x^2-1}{2}-\sqrt{x^2-1}\\ \Rightarrow A=\left(\dfrac{\sqrt{x+1}+\sqrt{x-1}}{\sqrt{x^2-1}}\right)^2\cdot\dfrac{x^2-1}{2}-\sqrt{x^2-1}\\ \Rightarrow A=\dfrac{\left(\sqrt{x+1}+\sqrt{x-1}\right)^2}{2}-\sqrt{x^2-1}\\ \Rightarrow A=\dfrac{2x+2\sqrt{x^2-1}-2\sqrt{x^2-1}}{2}\\ \Rightarrow A=x$

Đúng(1)

Chúng tôi đề xuất

Tài nguyên

Ứng dụng mobile

Bảng xếp hạng

Các khóa học có thể bạn quan tâm

Yêu cầu VIP