
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a) A chia hết cho 2 vì tất cả các số hạng của tổng đều chia hết cho 2.
b) Ta tách ghép các số hạng của A thành các nhóm sao cho mỗi nhóm xuất hiện thừa số chia hết cho 3. Khi đó:

![]()
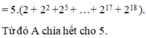

7)a) abcabc : abc = 1001
abcabc = 1001 x abc . Mà 1001 chia hết cho 7; 11; 13 nên 1001 x abc chia hết cho 7; 11; 13 . Vậy abcabc chia hết cho 7; 11; 13 ( đpcm)
b .Vì abc = 2 . deg nên abcdeg : deg = 2001
abcdeg = 2001 x deg. Do 2001 chia hết cho 23 và 29 nên 2001 x deg chia hết cho 23 và 29 . Vậy abcdeg chia hết cho 23 và 29 ( đpcm)
Ta có :
abcabc = 1000abc + abc
= 1001 . abc
= 7 . 11 . 13 . abc chia hết cho 7 ; 11 ; 13

+, Ta có:
\(B=23!+19!-15!\)
\(B=\left(1\times2\times...\times11\times...\times23\right)+\left(1\times2\times...\times11\times...\times19\right)-\left(1\times2\times...\times11\times...\times15\right)\)
\(B=11\times\left[\left(1\times2\times...\times10\times12\times...\times23\right)+\left(1\times2\times...\times10\times12\times...\times19\right)-\left(1\times2\times...\times10\times12\times...\times15\right)\right]\)
\(\Rightarrow B⋮11\)
+, Ta có:
\(B=23!+19!-15!\)
\(B=\left(1\times2\times...\times10\times11\times...\times23\right)+\left(1\times2\times...\times10\times11\times...\times19\right)-\left(1\times2\times...\times10\times11\times...\times15\right)\)
\(B=11\times10\times\left[\left(1\times2\times...\times9\times12\times...\times23\right)+\left(1\times2\times...\times9\times12\times...\times19\right)-\left(1\times2\times...\times9\times12\times...\times15\right)\right]\)
\(B=110\times\left[\left(1\times2\times...\times9\times12\times...\times23\right)+\left(1\times2\times...\times9\times12\times...\times19\right)-\left(1\times2\times...\times9\times12\times...\times15\right)\right]\)
\(\Rightarrow B⋮110\)
+,Ta có:
\(B=23!+19!-15!\)
\(B=\left(1\times2\times...\times5\times...\times23\right)+\left(1\times2\times...\times5\times...\times19\right)-\left(1\times2\times...\times5\times...\times15\right)\)
\(B=5\times\left[\left(1\times2\times...\times4\times6\times...\times23\right)+\left(1\times2\times...\times4\times6\times...\times19\right)-\left(1\times2\times...\times4\times6\times...\times15\right)\right]\)
\(\Rightarrow B⋮5\)
~ Chúc bạn học tốt ~!

Ta có: 7a+3b⋮23⇒6(7a+3b)⋮237a+3b⋮23⇒6(7a+3b)⋮23
⇒6(7a+3b)+(4a+5b)⋮23⇒6(7a+3b)+(4a+5b)⋮23
⇒46a+23b⋮23⇒23(2a+b)⋮23⇒46a+23b⋮23⇒23(2a+b)⋮23(Đúng)
Vậy 4a+5b⋮23

`#3107.101107`
a,
\(C=2+2^3+2^5+...+2^{23}\)
\(=\left(2+2^3+2^5\right)+\left(2^5+2^7+2^9\right)+...+\left(2^{19}+2^{21}+2^{23}\right)\)
\(=2\left(1+2^2+2^4\right)+2^5\cdot\left(1+2^2+2^4\right)+...+2^{19}\cdot\left(1+2^2+2^4\right)\)
\(=\left(1+2^2+2^4\right)\cdot\left(2+2^5+...+2^{19}\right)\)
\(=21\cdot\left(2+2^5+...+2^{19}\right)\)
Vì \(21\text{ }⋮\text{ }21\)
\(\Rightarrow21\left(2+2^5+...+2^{19}\right)\text{ }⋮\text{ }21\)
Vậy, \(C\text{ }⋮\text{ }21\)
b,
\(C=2+2^3+2^5+...+2^{23}\)
\(=\left(2+2^3\right)+\left(2^5+2^7\right)+...+\left(2^{21}+2^{23}\right)\)
\(=\left(2+2^3\right)+2^4\cdot\left(2+2^3\right)+...+2^{20}\cdot\left(2+2^3\right)\)
\(=\left(2+2^3\right)\cdot\left(1+2^4+...+2^{20}\right)\)
\(=10\cdot\left(1+2^4+...+2^{20}\right)\)
Vì \(10\text{ }⋮\text{ }10\)
\(\Rightarrow10\cdot\left(1+2^4+...+2^{20}\right)\text{ }⋮\text{ }10\)
Vậy, \(C\text{ }⋮\text{ }10.\)
a) c = 2 + 2³ + 2⁵ + ... + 2¹⁹ + 2²¹ + 2²³
= (2 + 2³ + 2⁵) + (2⁷ + 2⁹ + 2¹¹) + ... + (2¹⁹ + 2²¹ + 2²³)
= 2.(1 + 2² + 2⁴) + 2⁷.(1 + 2² + 2⁴) + ... + 2¹⁹.(1 + 2² + 2⁴)
= 2.21 + 2⁷.21 + ... + 2¹⁹.21
= 21.(2 + 2⁷ + ... + 2¹⁹) ⋮ 21
Vậy c ⋮ 21
b) c = 2 + 2³ + 2⁵ + 2⁷ + ... + 2²¹ + 2²³
= (2 + 2³) + (2⁵ + 2⁷) + ... + (2²¹ + 2²³)
= 10 + 2⁴.(2 + 2³) + ... + 2²⁰.(2 + 2³)
= 10 + 2⁴.10 + ... + 2²⁰.10
= 10.(1 + 2⁴ + ... + 2²⁰) ⋮ 10
Vậy c ⋮ 10

a) \(A=2+2^2+2^3+...+2^{20}\)
\(A=\left(2+2^2\right)+\left(2^3+2^4\right)+...+\left(2^{19}+2^{20}\right)\)
\(A=2\cdot\left(1+3\right)+2^3\cdot\left(1+3\right)+...+2^{59}\cdot\left(1+3\right)\)
\(A=3\cdot\left(2+2^3+...+2^{59}\right)\)
Vậy A chia hết cho 3
________
\(A=2+2^2+2^3+...+2^{20}\)
\(A=\left(2+2^3\right)+\left(2^2+2^4\right)+...+\left(2^{58}+2^{60}\right)\)
\(A=2\cdot\left(1+4\right)+2^2\cdot\left(1+4\right)+...+2^{58}\cdot\left(1+4\right)\)
\(A=5\cdot\left(2+2^2+...+2^{58}\right)\)
Vậy A chia hết cho 5
Ta gọi 4m+5 là a;7m+3 là b,ta có:
a chia hết cho 23 thì 4a cũng chia hết cho 23 nên 4a=(4m+5)=16m+20
Ta đặt c=4a+b=(16m+20)+(7m+3)=(16+7)m+(20+3)=23m+23
Vì 23m+23 luôn chia hết cho 23 mà 16m+20 cũng chia hết nên 7m+3 phải chia hết cho 23