Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a. Làm gọn 1 chút xíu:
\(y=\left(x^{11}+2x^7-3x^5-6x\right)\left(3x^7+6x^2-2\right)\)
\(y'=\left(11x^{10}+14x^6-15x^4-6\right)\left(3x^7+6x^2-2\right)+\left(21x^6+12x\right)\left(x^{11}+2x^7-3x^5-6x\right)\)
b.
\(y'=5\left(x^4-\dfrac{2}{3x}\right)^4\left(4x^3+\dfrac{2}{3x^2}\right)\Rightarrow y'\left(10\right)=5\left(10^4-\dfrac{2}{30}\right)^4\left(4.10^3+\dfrac{2}{300}\right)=?\)
c.
\(y'=\dfrac{7}{\left(x+1\right)^2}\Rightarrow y'\left(4\right)=\dfrac{7}{25}\)

a, \(y'=-2x+4\\ y'\left(-1\right)=6\\ y\left(-1\right)=-1\)
Phương trình tiếp tuyến tại điểm có hoành độ bằng -1 là: \(y=6\left(x+1\right)-1=6x+5\)
b, \(y'=-12x^2+6x\\ y'\left(2\right)=-36\\y\left(2\right)=-16 \)
Phương trình tiếp tuyến tại điểm có hoành độ bằng 2 là: \(y=-36\left(x-2\right)-16=-36x+56\)
c, \(y'=-4x^3+4x\\ y'\left(\sqrt{2}\right)=4\sqrt{2}\\ y\left(\sqrt{2}\right)=4\)
Phương trình tiếp tuyến tại điểm có hoành độ bằng \(\sqrt{2}\) là: \(y=4\sqrt{2}\left(x-\sqrt{2}\right)+4=4\sqrt{2}x-4\)

1. \(y'=\sqrt{x-2}+\dfrac{x+1}{2\sqrt{x-2}}\)
2. \(y'=-\dfrac{\dfrac{1}{2\sqrt{x^2+4x+5}}\cdot\left(x^2+4x+5\right)'}{x^2+4x+5}=-\dfrac{x+2}{\sqrt{\left(x^2+4x+5\right)^3}}\)
3. \(y'=\dfrac{\dfrac{x-1}{2\sqrt{x+1}}-\sqrt{x+1}}{\left(x-1\right)^2}=\dfrac{-x-3}{\left(x-1\right)^2\sqrt{x+1}}\)
4. \(y'=\dfrac{\sqrt{x^2+1}-\dfrac{x+1}{2\sqrt{x^2+1}}\cdot\left(x^2+1\right)'}{x^2+1}=\dfrac{\dfrac{2\left(x^2+1\right)-\left(x+1\right)\cdot2x}{2\sqrt{x^2+1}}}{x^2+1}=\dfrac{1-x}{\sqrt{\left(x^2+1\right)^3}}\)
5. \(y'=-\dfrac{\dfrac{\left(4-3x^2\right)'}{2\sqrt{4-3x^2}}}{4-3x^2}=\dfrac{3x}{\sqrt{\left(4-3x^2\right)^3}}\)
1. \(y'=\sqrt{x-2}+\dfrac{x+1}{2\sqrt{x-2}}=\dfrac{3x-3}{2\sqrt{x-2}}\)
2. \(y'=-\dfrac{\left(\sqrt{x^2+4x+5}\right)'}{x^2+4x+5}=-\dfrac{x+2}{\left(x^2+4x+5\right)\sqrt{x^2+4x+5}}\)
3. \(y'=\dfrac{\dfrac{\left(x-1\right)}{2\sqrt{x+1}}-\sqrt{x+1}}{\left(x-1\right)^2}=\dfrac{-x-3}{2\left(x-1\right)^2\sqrt{x+1}}\)
4. \(y'=\dfrac{\sqrt{x^2+1}-\dfrac{x\left(x+1\right)}{\sqrt{x^2+1}}}{x^2+1}=\dfrac{1-x}{\left(x^2+1\right)\sqrt{x^2+1}}\)
5. \(y'=\dfrac{\left(\sqrt{4-3x^2}\right)'}{3x^2-4}=\dfrac{-3x}{\left(3x^2-4\right)\sqrt{4-3x^2}}\)

a, \(y'=8x^3-6x\\ y'\left(-\sqrt{2}\right)=-10\sqrt{2}\\ y\left(-\sqrt{2}\right)=-1\)
Phương trình tiếp tuyến tại điểm có hoành độ bằng \(-\sqrt{2}\) là \(y=-10\sqrt{2}\left(x+\sqrt{2}\right)-1=-10\sqrt{2}x-21\)
b, \(y'=-\dfrac{13}{\left(2x-3\right)^2}\\ y'\left(1\right)=-13\\ y\left(1\right)=-6\)
Phương trình tiếp tuyến tại điểm có hoành độ bằng 1 là \(-13\left(x-1\right)-6=-13x+7\)

a: \(y'=\left(x^2\right)'+\left(3x\right)'-\left(6x^6\right)'+\left(\dfrac{2x-3}{x-1}\right)'\)
\(=2x+3-6\cdot6x^5+\dfrac{\left(2x-3\right)'\left(x-1\right)-\left(2x-3\right)\left(x-1\right)'}{\left(x-1\right)^2}\)
\(=-36x^5+2x+3+\dfrac{2\left(x-1\right)-2x+3}{\left(x-1\right)^2}\)
\(=-36x^5+2x+3+\dfrac{1}{\left(x-1\right)^2}\)
b: \(\left(\sqrt{2x^2-3x+1}\right)'=\dfrac{\left(2x^2-3x+1\right)'}{2\sqrt{2x^2-3x+1}}\)
\(=\dfrac{4x-3}{2\sqrt{2x^2-3x+1}}\)
\(y'=3\cdot2x-4+\dfrac{4x-3}{2\sqrt{2x^2-3x+1}}\)
\(=6x-4+\dfrac{4x-3}{2\sqrt{2x^2-3x+1}}\)
c: \(\left(\sqrt{4x^2-3x+1}\right)'=\dfrac{\left(4x^2-3x+1\right)'}{2\sqrt{4x^2-3x+1}}\)
\(=\dfrac{8x-3}{2\sqrt{4x^2-3x+1}}\)
\(y'=\left(\sqrt{4x^2-3x+1}\right)'-4'=\dfrac{8x-3}{2\sqrt{4x^2-3x+1}}\)
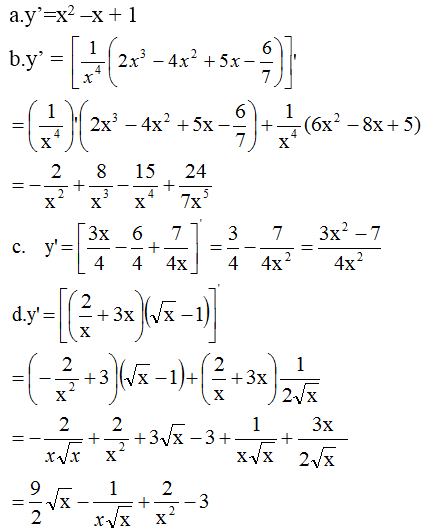
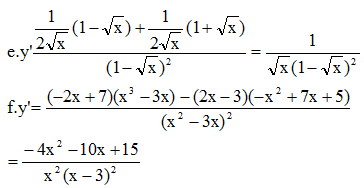
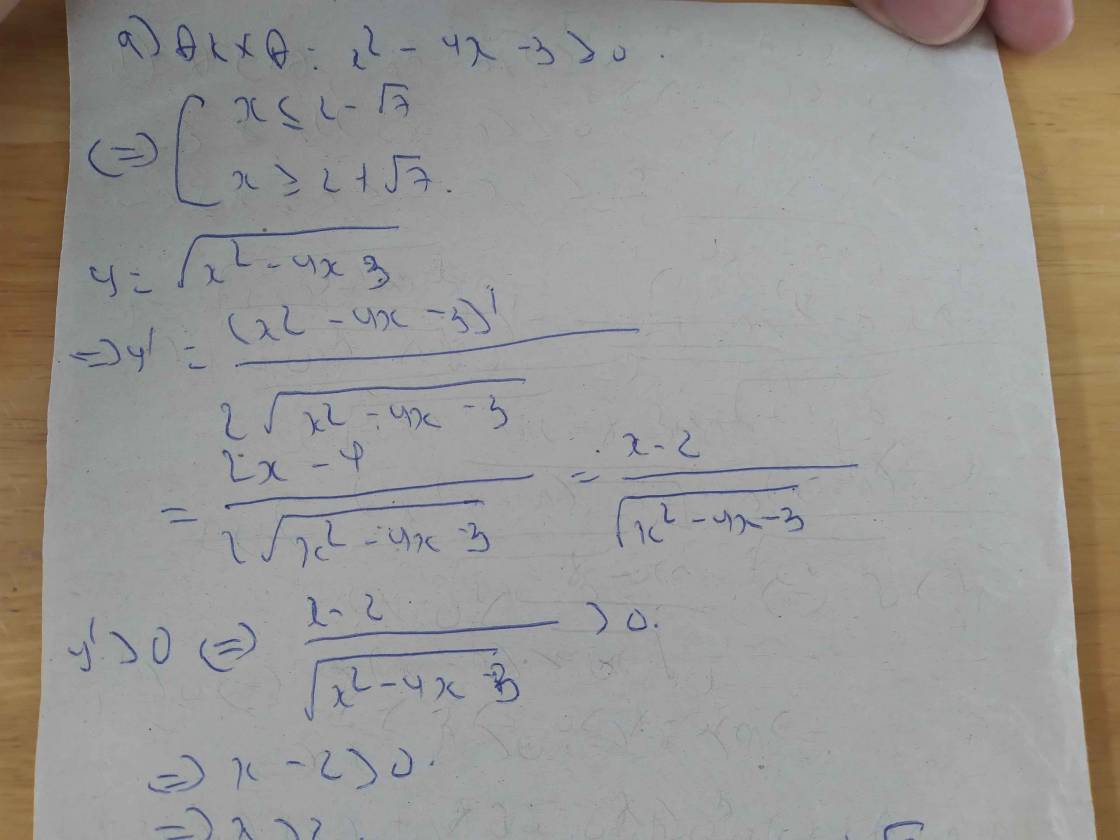

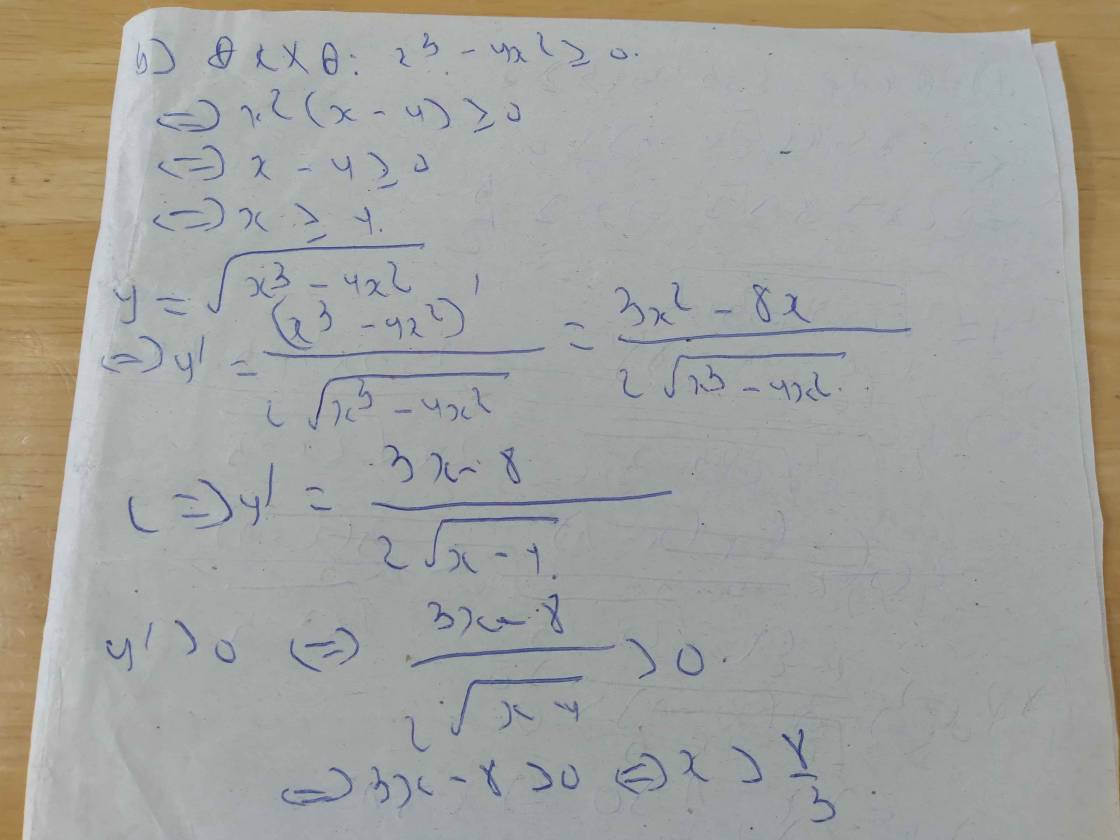

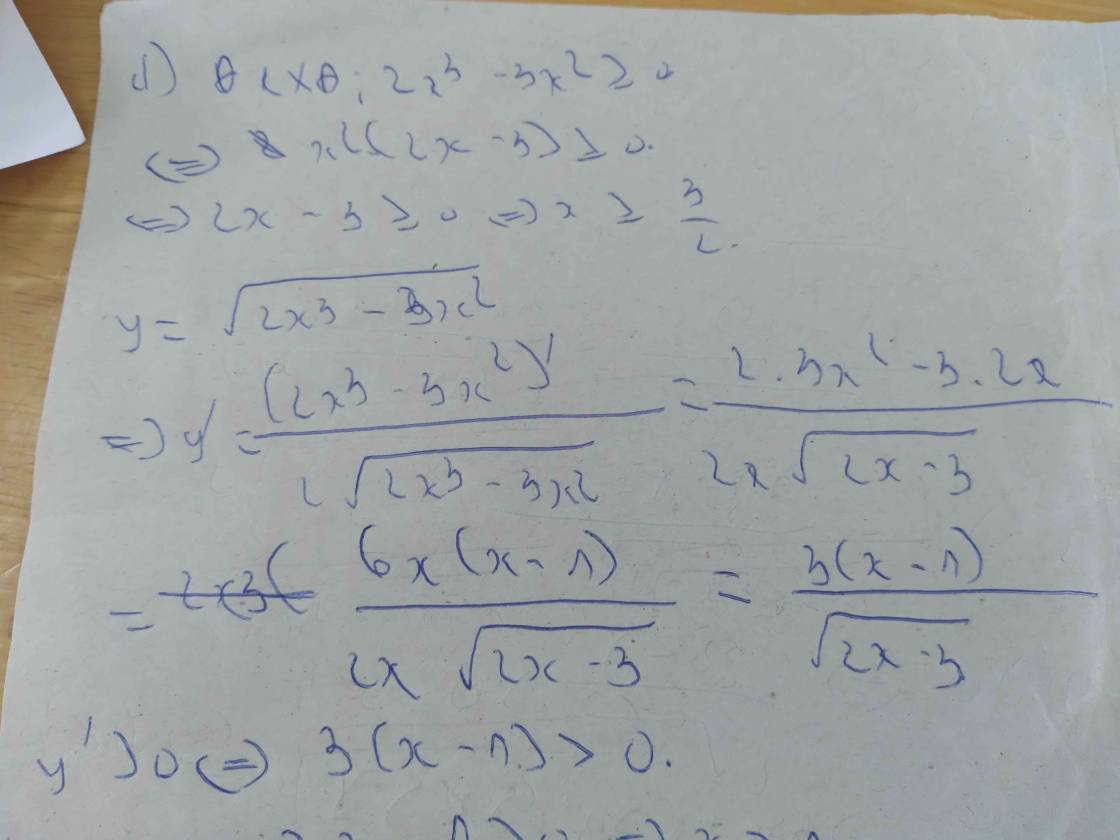

Câu 5
Thay x = -2 vào pt y = -3x^2 + 7x - 5
y = -12 - 14 - 5 = -26 - 5 = - 31
Câu 6 Thay x = 3 vào y = ( 3x - 1)^2
y = ( 9 - 1 )^2 = 64
Câu 7 : Thay x = 1/2 y = \(\sqrt{x^2+4x}\)
= \(\sqrt{\dfrac{1}{4}+2}=\sqrt{\dfrac{9}{4}}=\dfrac{3}{2}\)