Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Đồ thị này cắt trục Ox tại rất nhiều điểm chứ không phải chỉ có 1 điểm
=>Chọn C

Gọi M là trung điểm AB \(\Rightarrow M\left(-1;1\right)\)
\(\overrightarrow{BA}=\left(6;6\right)=6\left(1;1\right)\)
d cách đều 2 điểm AB khi d đi qua M hoặc d song song AB
TH1: d đi qua M
\(y'=\dfrac{1}{\left(x+1\right)^2}\) , gọi tiếp điểm có hoành độ \(x_0\Rightarrow\) phương trình tiếp tuyến:
\(y=\dfrac{1}{\left(x_0+1\right)^2}\left(x-x_0\right)+\dfrac{2x_0+1}{x_0+1}\)
Do tiếp tuyến qua M nên: \(1=\dfrac{1}{\left(x_0+1\right)^2}\left(-1-x_0\right)+\dfrac{2x_0+1}{x_0+1}\)
\(\Leftrightarrow x_0=1\Rightarrow\)tiếp tuyến: \(y=\dfrac{1}{4}\left(x-1\right)+\dfrac{3}{2}\)
TH2: tiếp tuyến song song AB \(\Rightarrow\) có hệ số góc \(k=\dfrac{1}{1}=1\)
\(\Rightarrow\dfrac{1}{\left(x+1\right)^2}=1\Rightarrow\left[{}\begin{matrix}x=0\Rightarrow y=1\\x=-2\Rightarrow y=3\end{matrix}\right.\)
Có 2 tiếp tuyến thỏa mãn: \(\left[{}\begin{matrix}y=1\left(x-0\right)+1\\y=1\left(x+2\right)+3\end{matrix}\right.\)

\(y=\dfrac{2x+2}{x-1}\Rightarrow y'=\dfrac{-4}{\left(x-1\right)^2}\)
a. \(y'\left(2\right)=-4\)
Phương trình tiếp tuyến: \(y=-4\left(x-2\right)+4\Leftrightarrow y=-4x+12\)
b. Pt hoành độ giao điểm:
\(\dfrac{2x+2}{x-1}=2x-1\Leftrightarrow2x^2-5x-1=0\Rightarrow\left[{}\begin{matrix}x=\dfrac{5-\sqrt{33}}{4}\\x=\dfrac{5+\sqrt{33}}{4}\end{matrix}\right.\)
\(y'\left(\dfrac{5-\sqrt{33}}{4}\right)=-\dfrac{17+\sqrt{33}}{8}\) ; \(y'\left(\dfrac{5+\sqrt{33}}{4}\right)=\dfrac{-17+\sqrt{33}}{8}\)
\(y\left(\dfrac{5-\sqrt{33}}{4}\right)=\dfrac{3-\sqrt{33}}{2}\) ; \(y\left(\dfrac{5+\sqrt{33}}{4}\right)=\dfrac{3+\sqrt{33}}{2}\)
Có 2 tiếp tuyến thỏa mãn:
\(\left[{}\begin{matrix}y=\dfrac{-17-\sqrt{33}}{8}\left(x-\dfrac{5-\sqrt{33}}{4}\right)+\dfrac{3-\sqrt{33}}{2}\\y=\dfrac{-17+\sqrt{33}}{8}\left(x-\dfrac{5+\sqrt{33}}{4}\right)+\dfrac{3+\sqrt{33}}{2}\end{matrix}\right.\)
Đề bài cho số liệu thật kì quặc

1.
ĐKXĐ: \(\left\{{}\begin{matrix}cosx\ne0\\tanx-sinx\ne0\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}cosx\ne0\\\dfrac{sinx}{cosx}-sinx\ne0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}cosx\ne0\\sinx\ne0\\cosx\ne1\end{matrix}\right.\) \(\Leftrightarrow sin2x\ne0\Leftrightarrow x\ne\dfrac{k\pi}{2}\)
2.
ĐKXĐ: \(sin2x\ne0\Leftrightarrow x\ne\dfrac{k\pi}{2}\)
3.
ĐKXĐ: \(\left\{{}\begin{matrix}sin\left(x-\dfrac{\pi}{4}\right)\ne0\\cos\left(x-\dfrac{\pi}{4}\right)\ne0\end{matrix}\right.\)
\(\Leftrightarrow sin\left(2x-\dfrac{\pi}{2}\right)\ne0\Leftrightarrow cos2x\ne0\)
\(\Leftrightarrow x\ne\dfrac{\pi}{4}+\dfrac{k\pi}{2}\)

1: ĐKXĐ: 3-cosx>0
=>cosx<3(luôn đúng)
2: ĐKXĐ: 1-sin 3x>=0
=>sin 3x<=1(luôn đúng)
3: ĐKXĐ: sin x<>0 và 2x<>pi/2+kpi
=>x<>kpi và x<>pi/4+kpi/2
4: ĐKXĐ: 2x-1>=0
=>x>=1/2

Ta có hàm số: \(y=\dfrac{1-cosx}{tanx}\) hàm số được xác định khi:
\(\left\{{}\begin{matrix}cosx\ne0\\tanx\ne0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ne\dfrac{\pi}{2}+k\pi\\x\ne k\pi\end{matrix}\right.\left(k\in Z\right)\)
\(\Leftrightarrow x\ne\dfrac{k\pi}{2}\)
Tập xác định của y là:
\(D=R\backslash\left(\dfrac{k\pi}{2};k\in Z\right)\)
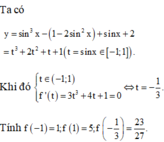

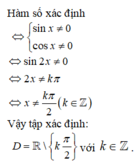
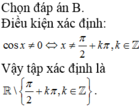
Câu 1. Hàm số xác định \(\Leftrightarrow\cos x\ne0\Leftrightarrow x\ne\dfrac{\pi}{2}+k2\pi\)
Câu 2. có \(-1\le\sin3x\le1\Leftrightarrow2\le\sin3x+3\le4\)
tập giá trị của hàm số : [2;4]