Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Bài 3.
Tính số học sinh của lớp 6A.
lớp của 6A trường câụ là bao nhiêu rồi ghi vó là được
chúc bạn học tốt

a) \(x\in\left\{-1;0;1;2\right\}\)
b) \(x\in\left\{0;1;2;3;4;5\right\}\)


3/ Chu vi hình chữ nhật:
\(\left(\dfrac{1}{4}+\dfrac{3}{10}\right)\cdot2=\dfrac{11}{10}\) (chưa biết đơn vị)
Diện tích hình chữ nhật:
\(\dfrac{1}{4}\cdot\dfrac{3}{10}=\dfrac{11}{20}\) (chưa biết đơn vị)

\(\left(x-2\right)\left(x-4\right)< 0\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x-2< 0\\x-4>0\end{matrix}\right.=>4< x< 2\left(1\right)\\\left\{{}\begin{matrix}x-2>0\\x-4< 0\end{matrix}\right.=>2< x< 4\left(2\right)}\end{matrix}\right.\)(1 ) vô lý=> loại
=> (x-2).(x-4)<0 <=> 2<x<4
b. ta có\(x^2+1>0\forall x\)
=>(x2 -1).(x2+1)<0 <=> (x2 -1)<0 <=> x2<1
<=> -1<x<1
câu c bạn làm tương tự

a) \(100:\left\{250:\left[450-\left(4.5^3-2^2.25\right)\right]\right\}\)
\(=100:\left\{250:\left[450-\left(4.125-4.25\right)\right]\right\}\)
\(=100:\left\{250:\left[450-\left(500-100\right)\right]\right\}\)
\(=100:\left[250:\left(450-400\right)\right]\)
\(=100:\left(250:50\right)\)
\(=100:5\)
\(=20\)
b) \(109.5^2-3^2.25\)
\(=109.25-9.25\)
\(=25\left(109-9\right)\)
\(=25.100\)
\(=2500\)
c) \(\left[5^2.6-20.\left(37-2^5\right)\right]:10-20\)
\(=\left[5^2.6-20.\left(37-32\right)\right]:10-20\)
\(=\left(5^2.6-20.5\right):10-20\)
\(=\left(25.6-20.5\right):10-20\)
\(=\left(150-100\right):10-20\)
\(=50:10-20\)
\(=5-20\)
\(=-15\)

31 + 32 + ..... + 3100
Đặt A = 31 + 32 + .... + 3100
Số hạng của A là :
(100 - 1) : 1 + 1 = 100 ( số hạng )
Vì 100 \(⋮\) 2 , ta nhóm A như sau :
A = 31 + 32 + .... + 3100
A = (31 + 32) + (33 + 34) + .... + (399 + 3100)
A = 3(1 + 3) + 33(1 + 3) + .... + 399(1 + 3)
A = 3.4 + 33.4 + .... + 399.4
A = 4(3 + 33 + .... + 399)
Vì 4 \(⋮\) 4 \(\Rightarrow\) 4(3 + 33 + .... + 399) \(⋮\) 4
Hay A \(⋮\) 4
Vậy A chia hết cho 4.

Nếu là z+x thì mik biết làm nè:
Đặt x-y=2011(1)
y-z=-2012(2)
z+x=2013(3)
Cộng (1);(2);(3) lại với nhau ta được :
2x=2012=>x=1006
Từ (1) => y=-1005
Từ (3) => z=1007
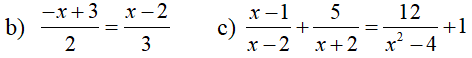


 tìm x: nhanh nhanh lên nhé mình đang cần gấp
tìm x: nhanh nhanh lên nhé mình đang cần gấp
\(a,\left(3x-7\right)\left(x+5\right)=\left(5+x\right)\left(3-2x\right)\)
\(\Leftrightarrow\left(3x-7\right)\left(x+5\right)-\left(x+5\right)\left(3-2x\right)=0\)
\(\Leftrightarrow\left(x+5\right)\left(3x-7-3+2x\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+5=0\\5x-10=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-5\\x=2\end{matrix}\right.\)
\(b,\dfrac{-x+3}{2}=\dfrac{x-2}{3}\left(MSC=6\right)\)
Suy ra :
\(3\left(-x+3\right)=2\left(x-2\right)\)
\(\Leftrightarrow-3x+9-2x+4=0\)
\(\Leftrightarrow-5x+13=0\)
\(\Leftrightarrow x=\dfrac{13}{5}\)
\(c,\dfrac{x-1}{x-2}+\dfrac{5}{x+2}=\dfrac{12}{x^2-4}+1\)\(\left(dkxd:x\ne\pm2\right)\)
\(\Leftrightarrow\dfrac{\left(x-1\right)\left(x+2\right)+5\left(x-2\right)-12-x^2+4}{x^2-4}=0\)
\(\Leftrightarrow x^2+2x-x-2+5x-10-12-x^2+4=0\)
\(\Leftrightarrow6x-20=0\)
\(\Leftrightarrow x=\dfrac{10}{3}\)\(\left(n\right)\)
Vậy \(S=\left\{\dfrac{10}{3}\right\}\)
a
b
phải ko bn