D
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
29 tháng 11 2022
a: \(B=\left(\dfrac{4x}{x+2}-\dfrac{\left(x-2\right)\left(x^2+2x+4\right)}{\left(x+2\right)\left(x^2-2x+4\right)}\cdot\dfrac{4\left(x^2-2x+4\right)}{\left(x-2\right)\left(x+2\right)}\right)\cdot\dfrac{x+2}{16}\cdot\dfrac{\left(x+2\right)\left(x+1\right)}{x^2+x+1}\)
\(=\left(\dfrac{4x}{x+2}-\dfrac{4\left(x^2+2x+4\right)}{\left(x+2\right)^2}\right)\cdot\dfrac{x+2}{16}\cdot\dfrac{\left(x+2\right)\left(x+1\right)}{x^2+x+1}\)
\(=\dfrac{4x^2+8x-4x^2-8x-16}{\left(x+2\right)^2}\cdot\dfrac{\left(x+2\right)^2\cdot\left(x+1\right)}{16\left(x^2+x+1\right)}\)
\(=\dfrac{-16}{16\left(x^2+x+1\right)}\cdot\left(x+1\right)=-\dfrac{x+1}{x^2+x+1}\)
b: \(B=\dfrac{\left(x+2\right)\left(x-1\right)}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{x+2}{x^2+x+1}\)
\(P=A+B=\dfrac{-x-1+x+2}{x^2+x+1}=\dfrac{1}{x^2+x+1}=\dfrac{1}{\left(x+\dfrac{1}{2}\right)^2+\dfrac{3}{4}}< =1:\dfrac{3}{4}=\dfrac{4}{3}\)
Dấu = xảy ra khi x=-1/2
PN
17 tháng 5 2017
3x.|x+1|−2x|x+2|=12
Với x < -2 ta có: 3x.(-x-1)-2x(-x-2)-12=0
<=> -3x2 - 3x + 2x2 + 4x -12 =0
<=> -x2 - x - 12=0
$\Leftrightarrow $ -(x2 +x+12)=0 ( vô lý)
Làm tương tự với 2 trường hợp còn lại:
begin{align}
\begin{cases}
-2 bé hơn hoặc bằng x bé hơn -1 \\
x lớn hơn hoặc bằng -1 \\
\end{cases}
\end{align}
12 tháng 7 2017
a, \(\dfrac{4x^2-8xy}{10y-5x}=\dfrac{4x\left(x-2y\right)}{5\left(2y-x\right)}=\dfrac{-4x}{5}\)
b, \(\dfrac{\left(x-2\right)^2-1}{x^2-6x+9}=\dfrac{\left(x-2-1\right)\left(x-2+1\right)}{\left(x-3\right)^2}\)
\(=\dfrac{\left(x-3\right)\left(x-1\right)}{\left(x-3\right)^2}=\dfrac{x-1}{x-3}\)
c, \(\dfrac{x^2+8x+16}{x^2-16}=\dfrac{\left(x+4\right)^2}{\left(x-4\right)\left(x+4\right)}=\dfrac{x+4}{x-4}\)
4 tháng 12 2022
a: \(=\dfrac{4x^3+8x^2-11x+3-\left(x^2-5\right)\left(2x-1\right)-2x^3-5x^2+x+1}{\left(2x-1\right)^3}\)
\(=\dfrac{2x^3+3x^2-10x+4-2x^3+x^2+10x-5}{\left(2x-1\right)^3}\)
\(=\dfrac{4x^2-1}{\left(2x-1\right)^3}=\dfrac{2x+1}{\left(2x-1\right)^2}\)
b: \(=\dfrac{1+x+1-x}{1-x^2}+\dfrac{2}{1+x^2}+\dfrac{4}{1+x^4}+\dfrac{8}{1+x^8}+\dfrac{16}{1+x^{16}}\)
\(=\dfrac{2+2x^2+2-2x^2}{1-x^4}+\dfrac{4}{1+x^4}+\dfrac{8}{1+x^8}+\dfrac{16}{1+x^{16}}\)
\(=\dfrac{4+4x^4+4-4x^4}{1-x^8}+\dfrac{8}{1+x^8}+\dfrac{16}{1+x^{16}}\)
\(=\dfrac{8+8x^8+8-8x^8}{1-x^{16}}+\dfrac{16}{1+x^{16}}\)
\(=\dfrac{32}{1+x^{32}}\)
24 tháng 12 2018
a) \(\dfrac{x}{x-4}+\dfrac{4-x}{16-x^2}=\dfrac{x\left(x+4\right)}{\left(x-4\right)\left(x+4\right)}-\dfrac{4-x}{\left(x-4\right)\left(x+4\right)}\)
\(=\dfrac{x^2+4x-4+x}{\left(x-4\right)\left(x+4\right)}=\dfrac{x^2+5x-4}{\left(x-4\right)\left(x+4\right)}\)
b) \(\dfrac{4x^2+8x+4}{x^2-1}:\dfrac{1+x}{1-x}=\dfrac{4\left(x^2+2x+1\right)}{\left(x-1\right)\left(x+1\right)}\cdot\dfrac{1-x}{1+x}\)
\(=\dfrac{4\left(x+1\right)^2\left(1-x\right)}{-\left(1-x\right)\left(x+1\right)\left(x+1\right)}=-4\)
GH
20 tháng 2 2018
a)\(\dfrac{3x+2}{3x-2}-\dfrac{6}{2+3x}=\dfrac{9x^2}{9x^2-4}\left(ĐKXĐ:x\ne\pm\dfrac{2}{3}\right)\)
\(\Leftrightarrow\dfrac{3x+2}{3x-2}-\dfrac{6}{3x+2}=\dfrac{9x^2}{\left(3x-2\right)\left(3x+2\right)}\)
\(\Leftrightarrow\dfrac{\left(3x+2\right)^2-6\left(3x-2\right)}{\left(3x-2\right)\left(3x+2\right)}=\dfrac{9x^2}{\left(3x-2\right)\left(3x+2\right)}\)
\(\Rightarrow9x^2+12x+4-18x+12=9x^2\)
\(\Leftrightarrow9x^2-6x+16-9x^2=0\)
\(\Leftrightarrow-6x=-16\)
\(\Leftrightarrow x=\dfrac{8}{3}\) (thỏa mãn ĐKXĐ)
Vậy .................
GH
20 tháng 2 2018
b) \(\dfrac{5-x}{4x^2-8x}+\dfrac{7}{8x}=\dfrac{x-1}{2x\left(x-2\right)}+\dfrac{1}{8x-16}\left(ĐKXĐ:x\ne0;x\ne2\right)\)
\(\Leftrightarrow\dfrac{5-x}{4x\left(x-2\right)}+\dfrac{7}{8x}=\dfrac{x-1}{2x\left(x-2\right)}+\dfrac{1}{8\left(x-2\right)}\)
\(\Leftrightarrow\dfrac{2\left(5-x\right)+7\left(x-2\right)}{8x\left(x-2\right)}=\dfrac{4\left(x-1\right)+x}{8x\left(x-2\right)}\)
\(\Rightarrow10-2x+7x-14=4x-4+x\)
\(\Leftrightarrow5x-4=5x-4\)
\(\Leftrightarrow0x=0\) (vô số nghiệm)
Vậy \(S=R\backslash\left\{0;2\right\}\)
VM
30 tháng 4 2017
\(\Leftrightarrow\) \(\dfrac{7}{8x}\)+\(\dfrac{5-x}{4x\left(x-2\right)}\)= \(\dfrac{x-1}{2x\left(x-2\right)}\)+ \(\dfrac{1}{8\left(x-2\right)}\)
\(\Rightarrow\) 7(x-2) + 2(5-x) = 4(x-1) +x
\(\Leftrightarrow\) 7x-2x+10-2x= 4x-4+x
\(\Leftrightarrow\)7x-2x-2x-4x-x = -4-10
\(\Leftrightarrow\) -2x = -14
\(\Leftrightarrow\) x = 7
Vậy phương trình có nghiệm x=7
11 tháng 2 2019
⇔ 78x78x+5−x4x(x−2)5−x4x(x−2)= x−12x(x−2)x−12x(x−2)+ 18(x−2)18(x−2)
⇔ 7(x-2)8x(x-2)78x+2(5−x)8x(x−2)5−x4x(x−2)= 4(x−1)28x(x−2)x−12x(x−2)+ x8x(x−2)
18(x−2)
⇒7(x-2)+2(5-x)=4(x-1)+x
⇔ 7x-2x+10-2x= 4x-4+x
⇔7x-2x-2x-4x-x = -4-10
⇔ -2x = -14
⇔ x = 7
vậy tập của phương trình là: S=7}
13 tháng 6 2022
1: \(B=\left(\dfrac{4x}{x+2}-\dfrac{\left(x-2\right)\left(x^2+2x+4\right)}{\left(x+2\right)\left(x^2-2x+4\right)}\cdot\dfrac{4\left(x^2-2x+4\right)}{\left(x-2\right)\left(x+2\right)}\right):\dfrac{16}{x+2}\cdot\dfrac{\left(x+2\right)\left(x+1\right)}{x^2+x+1}\)
\(=\left(\dfrac{4x}{x+2}-\dfrac{4\left(x^2+2x+4\right)}{\left(x+2\right)^2}\right)\cdot\dfrac{x+2}{16}\cdot\dfrac{\left(x+2\right)\left(x+1\right)}{x^2+x+1}\)
\(=\dfrac{4x^2+8x-4x^2-8x-16}{\left(x+2\right)^2}\cdot\dfrac{\left(x+2\right)^2\cdot\left(x+1\right)}{16\left(x^2+x+1\right)}\)
\(=\dfrac{-\left(x+1\right)}{x^2+x+1}\)
2: Để B=0 thì -x-1=0
hay x=-1(nhận)
14 tháng 11 2017
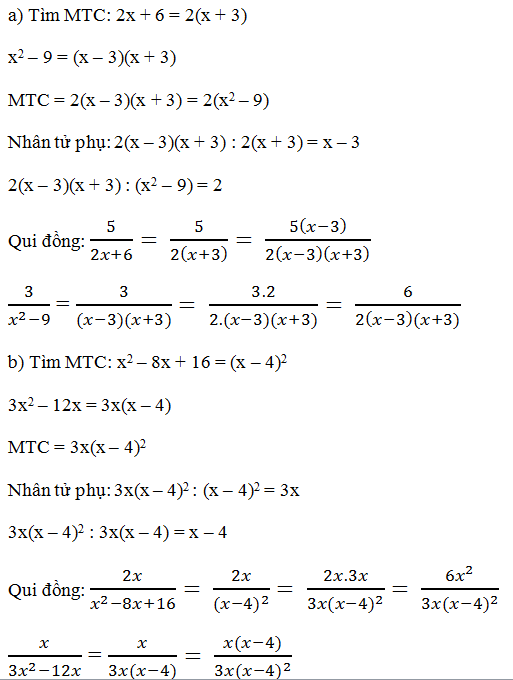
a) Tìm MTC:
2x + 6 = 2(x + 3)
x2 – 9 = (x – 3)(x + 3)
MTC = 2(x – 3)(x + 3) = 2(x2 – 9)
Nhân tử phụ:
2(x – 3)(x + 3) : 2(x + 3) = x – 3
2(x – 3)(x + 3) : (x2 – 9) = 2
Qui đồng:
b) Tìm MTC:
x2 – 8x + 16 = (x – 4)2
3x2 – 12x = 3x(x – 4)
MTC = 3x(x – 4)2
Nhân tử phụ:
3x(x – 4)2 : (x – 4)2 = 3x
3x(x – 4)2 : 3x(x – 4) = x – 4
Qui đồng:
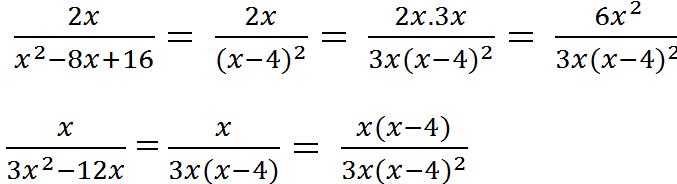
\(A=\dfrac{\left(x-4\right)\left(x+4\right)}{x}\cdot\dfrac{x}{\left(x-4\right)^2}=\dfrac{x+4}{x-4}\)
Để A=2 thì 2x-8=x+4
=>x=12