a 1 phần x + 2 phần x+1 cộng 3 phần x+2

Khách

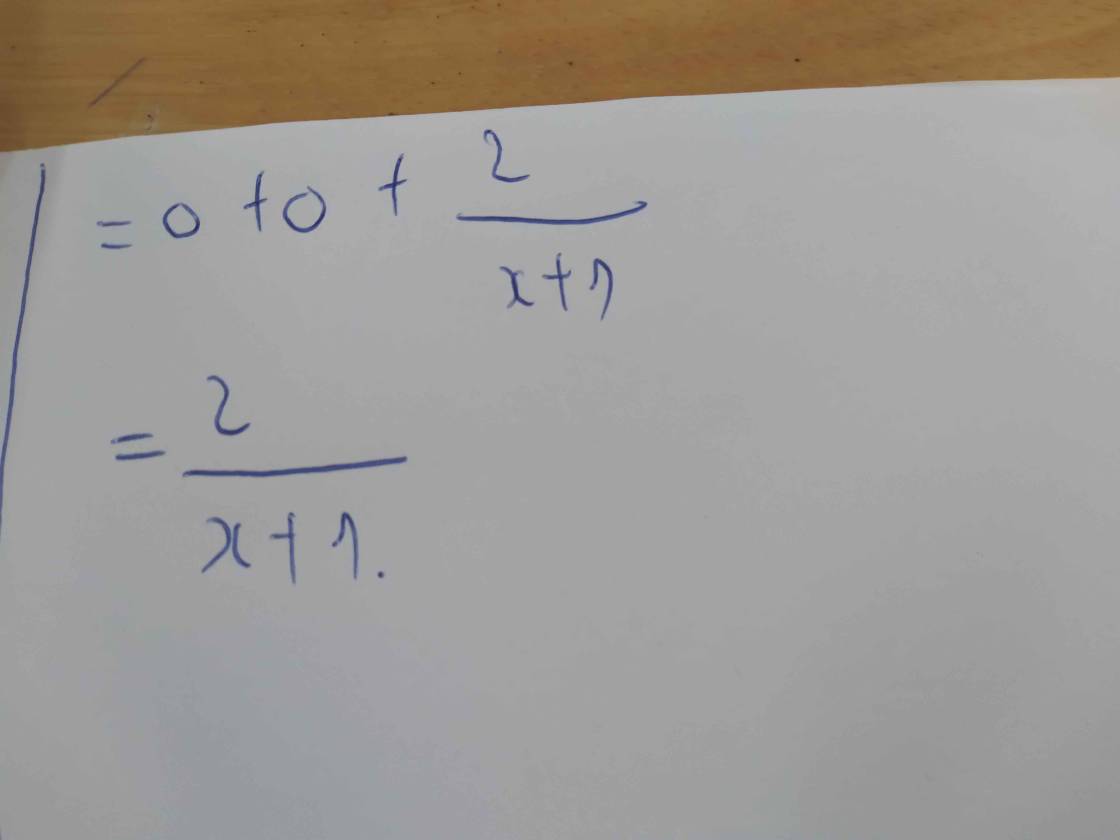
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Chọn lớp

Chọn môn

Mua vip

thảo phương

28 tháng 2 - olm

a 1 phần x + 2 phần x+1 cộng 3 phần x+2 _ 1 phần x trừ 3 phần x+2

#Toán lớp 8

Nguyễn Lê Phước Thịnh

1 tháng 3

Đúng(0)

Các câu hỏi dưới đây có thể giống với câu hỏi trên

Hoàng Gia Bảo

16 tháng 8 2023 - olm

âm x mũ 3 phần 8 cộng 3 phần 4 và x mũ 2 trừ 3 phần 2 và x cộng 1

#Toán lớp 8

Dii Quèngg

29 tháng 6 2021

Mn giúp mik bài này ạ

Giải phương trình

x + 1 phần x - 1 trừ x -1 phần x + 1 cộng x^2 + 3 phần 1 - x^2 bằng 0

#Toán lớp 8

Nguyễn Ngọc Lộc

29 tháng 6 2021

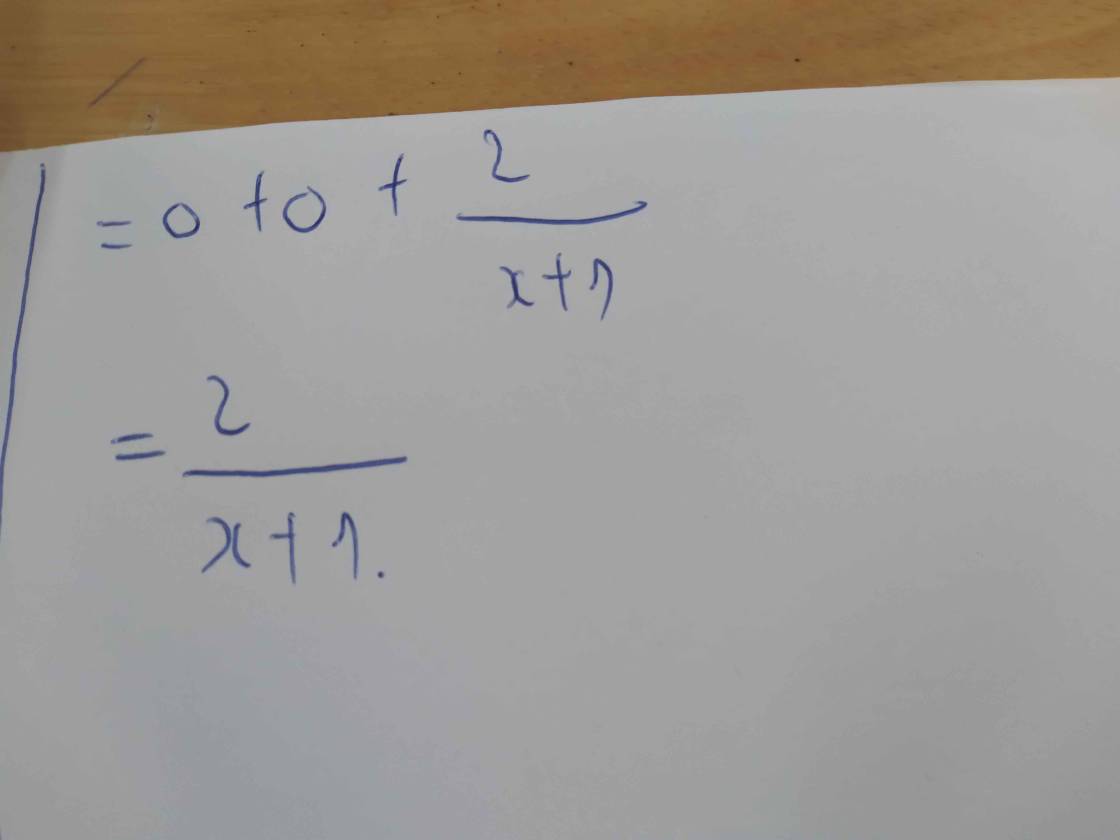
ĐKXĐ ; \(x\ne\pm1\)

Ta có : \(\dfrac{x+1}{x-1}-\dfrac{x-1}{x+1}+\dfrac{x^2+3}{1-x^2}=0\)

\(\Leftrightarrow\dfrac{\left(x+1\right)^2}{x^2-1}-\dfrac{\left(x-1\right)^2}{x^2-1}+\dfrac{-x^2-3}{x^2-1}=0\)

\(\Leftrightarrow\left(x+1\right)^2-\left(x-1\right)^2-x^2-3=0\)

\(\Leftrightarrow x^2+2x+1-x^2+2x-1-x^2-3=0\)

\(\Leftrightarrow-x^2+4x-3=0\)

\(\Leftrightarrow-x^2+3x+x-3=0\)

\(\Leftrightarrow-x\left(x-3\right)+\left(x-3\right)=0\)

\(\Leftrightarrow\left(x-3\right)\left(x-1\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=3\left(TM\right)\\x=1\left(L\right)\end{matrix}\right.\)

=> X = 3

Vậy ..

Đúng(3)

minhhien jo

28 tháng 12 2022

cho biểu thức 2 phần x trừ 2 trừ 4 x cộng 4 trừ x mũ 2 cộng x phần x cộng 2 tất cả chia x phần x trừ 2 tìm x nguyên âmđể A nguyên

#Toán lớp 8

xuan anh Phung

18 tháng 7 2016 - olm

*) tính tổng

A= 1/x-1 - 1/x+1 - 2/x^2+1 - 4/x^4+1 - 8/x^8+1 - 16/x^16+1

-) 1 phần x-1 trừ đi 1 phần x mũ 2 +1 trừ đi 4 phần x mũ 4 +1 trừ đi 8 phần x mũ 8 + 1 trừ đi 16 phần x mũ 16 +1 ( giải thích cho các ban hiểu ấy mà)

#Toán lớp 8

gfffffffh

1 tháng 3 2022

gfvfvfvfvfvfvfv555

Đúng(0)

Huỳnh Ngân

26 tháng 4 2018

Giải các phương trình sau

a) x phần 3+2x-1 phần 6=1 phần 2

b)3x+1 phần 2 + x-1 phần 3= x-9 phần 6

c)x+1 phần x-2=x+3 phần x+2

d)3x-1 phần 3x+1 + x-3 phần x+3=2

e)x phần x-1 - x-1 phần x =0

#Toán lớp 8

Aki Tsuki

26 tháng 4 2018

heoheo lần sau bạn đánh = kí hiệu đi :(((

a/ \(\dfrac{x}{3}+\dfrac{2x-1}{6}=\dfrac{1}{2}\)

\(\Leftrightarrow2x+2x-1=3\)

<=> 4x = 4 <=> x = 1

Vậy x = 1

b/ \(\dfrac{3x+1}{2}+\dfrac{x-1}{3}=\dfrac{x-9}{6}\)

\(\Leftrightarrow3\left(3x+1\right)+2\left(x-1\right)=x-9\)

\(\Leftrightarrow9x+3+2x-2=x-9\)

\(\Leftrightarrow10x=-10\Leftrightarrow x=-1\)

Vậy pt có nghiệm x = -1

c/ \(\dfrac{x-1}{x-2}=\dfrac{x+3}{x+2}\) ĐKXĐ: \(x\ne\pm2\)

<=> \(\left(x-1\right)\left(x+2\right)=\left(x+3\right)\left(x-2\right)\)

\(\Leftrightarrow x^2+2x-x-2=x^2-2x+3x-6\)

\(\Leftrightarrow0x=-4\left(voly\right)\)

Vậy pt vô nghiệm

d/ \(\dfrac{3x-1}{3x+1}+\dfrac{x-3}{x+3}=2\) ĐKXĐ: \(\left\{{}\begin{matrix}x\ne-3\\x\ne-\dfrac{1}{3}\end{matrix}\right.\)

pt <=> \(\dfrac{\left(3x-1\right)\left(x+3\right)}{\left(3x+1\right)\left(x+3\right)}+\dfrac{\left(x-3\right)\left(3x+1\right)}{\left(3x+1\right)\left(x+3\right)}=\dfrac{2\left(3x+1\right)\left(x+3\right)}{\left(3x+1\right)\left(x+3\right)}\)

=> (3x-1)(x+3) + (x-3)(3x+1) = 2(3x+1)(x+3)

\(\Leftrightarrow3x^2+8x-3+3x^2-8x-3=6x^2+20x+6\)

\(\Leftrightarrow-20x=12\Leftrightarrow x=-\dfrac{3}{5}\left(tm\right)\)

Vậy pt có nghiệm x=....

e/ như ý d

Đúng(0)

Huỳnh Ngân

26 tháng 4 2018

Mơn bn nhe ^^ tại mjk chưa bt ạk

Đúng(0)

Lê Ngọc Diệp

11 tháng 3 2018 - olm

x - 1 phần -1 + 1 phần x + 1 = 2 x trừ 1 phần x bình cộng x

Can nhanh cac ban nhe thank you

#Toán lớp 8

Thúy Ngân

11 tháng 3 2018

Ta có : \(\frac{x-1}{-1}+\frac{1}{x+1}=\frac{2x-1}{x^2+x}\)

\(\Rightarrow\) \(-x+1=\frac{2x-1}{x\left(x+1\right)}-\frac{1}{x+1}\) \(=\frac{1}{x+1}.\left(\frac{2x-1-x}{x}\right)=\frac{1}{x+1}.\frac{x-1}{x}\)

\(\Rightarrow\frac{1}{x+1}.\frac{x-1}{x}+x-1=0\)

\(\Rightarrow\frac{x-1}{x\left(x+1\right)}+\left(x-1\right)=0\)

\(\Rightarrow\left(x-1\right)\left(\frac{1}{x\left(x+1\right)}+1\right)=0\)

\(\Rightarrow x-1=0\)hoặc \(\frac{1}{x\left(x+1\right)}+1=0\)

\(\Rightarrow x=1\) hoặc \(\frac{1}{x\left(x+1\right)}=-1\)

\(\Rightarrow x=1\)hoặc \(x^2+x=-1\)

\(\Rightarrow x=1\)hoặc \(x^2+1+x=0\)

\(\Rightarrow x=1\)hoặc \(\left(x+\frac{1}{2}\right)^2+\frac{3}{4}=0\)

\(\Rightarrow x=1\)hoặc \(\left(x+\frac{1}{2}\right)^2=-\frac{3}{4}\)(vô lí)

Vậy : x = 1 thì thỏa mãn điều kiện bài toán.

Đúng(0)

Phạm Thị Thu Thủy

30 tháng 1 2022

×^2+8/×^2-4-×-1/×+2=×+1/×+2

Đúng(0)

Hắc Miu

30 tháng 6 2020

Giúp mik vs ạ>>

Giải bất phương trình sau:

+ 3x +5 < 14

+ 1 phần x trừ 1 trừ 3 phần x trừ 2 bằng âm 1 phần ( x-1)(x-2)

#Toán lớp 8

Tuyết Ngân

30 tháng 6 2020

3x+5<14

<=>3x<9

<=>x<3

vậy S=(x\(\in\)R/x<3)

Đúng(0)

Nguyễn Quỳnh Diệp

20 tháng 2 2018 - olm

Giải phương trình, xin lỗi vì mình không biết cách viết phân số nên mình sẽ ghi là phần nhaaa !1) 2 - x phần 2008, trừ 1 = 1 - x phần 2009, trừ x phần 20102) 4 ( x - 3 ) 2 = 9 ( 2 - 3x )2 3) x + 1 phần x - 1, cộng x2 + 3x - 2 phần 1 - x2 , = x - 1 phần x + 14) x + 97 phần 125, trừ x - 7 phần 21 = x - 77 phần 49, trừ x - 63 phần 355) Một số tự nhiên có 2 chữ số. Chữ số hàng chục gấp đôi chữ số hàng đơn vị....

Đọc tiếp

#Toán lớp 8

Mai Nhật Huy

24 tháng 2 2022

Giúp mình với ạ ;-;

a) (x-1)(2x^2-8)=0

b)3x^2-8x+5=0

c)(7x-1).2x-7x+1=0

d)(4x+2)(x-1)=1phần2x(x-1)

e)5x-2 phần 3 = 5-3x phần 2

f) 2x-1 phần x-1 + 1= 1 phần x-1

g) 1 phần x-2 + 3= x-3 phần 2-x

#Toán lớp 8

Đỗ Tuệ Lâm

24 tháng 2 2022

(x-1)(2x^2-8)=0

\(\Leftrightarrow\left(x-1\right)\left(2x^2-8\right)=0\\ \left(2x^3-8x-2x^2+8\right)=0\)

\(\Leftrightarrow2x\left(x-1\right)-8\left(x-1\right)=0\)

\(\Leftrightarrow x=1;x=\dfrac{8}{2}\)

3x^2-8x+5=0

áp dụng công thức bậc 2 ta có:

\(x=\dfrac{-\left(-8\right)\pm\sqrt{\left(-8\right)^2-4.3.5}}{2.3}\)

\(\Rightarrow x=\dfrac{5}{3};x=1\)

Đúng(4)

Đỗ Tuệ Lâm

24 tháng 2 2022

(7x-1).2x-7x+1=0

\(\Leftrightarrow\left(7x-1\right)\left(2x-1\right)=0\)

\(\Leftrightarrow x=\dfrac{1}{7};x=\dfrac{1}{2}\)

Đúng(2)

Hank Pham

8 tháng 6 2020

2(x-3)+5x(x-1)=5x mũ 2 (2x+1)(x -1)=0 3x-15=2x(x-5) 10× +3 phần 12=1 6+8x phần 9 (2x mũ 2+1)(4x-3)=(2x mũ 2+1)(x-12) (x+7)(3x-1)=49-x mũ 2 2x(x+2)mũ 2 -8x mũ 2=2(x-2)(x mũ 2+2x+4) (2x+5)mũ 2=(x+2)mũ 2 2(3x+1)+1 phần 4-5=2(3x-1) phần 5 3x+2 phần 10 3-7x phần 1+x=1 phần 2 X+7 phần x+4- 7 phần x-4=-56 phần x mũ 2 -16 x-3 phần x-2+x -2 phần x-4 =-1 1 phần x-1+2x mũ 2 -5 phần x mũ 3-1=4 phần x mũ 2+x+1 x-1 phần x+2-x phần x-2=5x -2 phần...

Đọc tiếp

#Toán lớp 8

Chúng tôi đề xuất

Tài nguyên

Ứng dụng mobile

Bảng xếp hạng

Các khóa học có thể bạn quan tâm

Yêu cầu VIP