Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: Ta có: \(\left(4\sqrt{2}-\dfrac{11}{2}\sqrt{8}-\dfrac{1}{3}\sqrt{288}+\sqrt{50}\right)\cdot\left(\dfrac{1}{2}\sqrt{2}\right)\)
\(=\dfrac{1}{2}\sqrt{2}\cdot\left(4\sqrt{2}-11\sqrt{2}-4\sqrt{2}+5\sqrt{2}\right)\)
\(=\dfrac{1}{2}\sqrt{2}\cdot6\sqrt{2}=3\)

1) \(A=2\sqrt{5}-6\sqrt{2}+3\sqrt{5}=5\sqrt{5}-6\sqrt{2}\)
2) \(B=\dfrac{30\left(\sqrt{7}+1\right)}{7-1}+\dfrac{15\left(\sqrt{7}-2\right)}{7-4}=5\sqrt{7}+5+5\sqrt{7}-10=-5+10\sqrt{7}\)
3) \(C=\left(3-\dfrac{\sqrt{5}\left(\sqrt{5}-1\right)}{\sqrt{5}-1}\right)\left(3+\dfrac{\sqrt{5}\left(\sqrt{5}+1\right)}{\sqrt{5}+1}\right)=\left(3-\sqrt{5}\right)\left(3+\sqrt{5}\right)=9-5=4\)
4) \(D=3-\sqrt{2}+1-\sqrt{2}=4-2\sqrt{2}\)

\(2,\\ a,PT\Leftrightarrow\sqrt{\left(5x-1\right)^2}=\sqrt{4\left(x+1\right)^2}\\ \Leftrightarrow\left|5x-1\right|=2\left|x+1\right|\\ \Leftrightarrow\left[{}\begin{matrix}5x-1=2\left(x+1\right)\\1-5x=2\left(x+1\right)\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}3x=3\\7x=-1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-\dfrac{1}{7}\end{matrix}\right.\)
\(b,ĐK:x^2-3\ge0\\ PT\Leftrightarrow\sqrt{x^2-3}=x-1\\ \Leftrightarrow x^2-3=x^2-2x+1\\ \Leftrightarrow2x=4\Leftrightarrow x=2\left(tm\right)\\ c,ĐK:x\le\dfrac{7}{2}\\ PT\Leftrightarrow7-2x=x^2+7\\ \Leftrightarrow x^2+2x=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\left(tm\right)\\x=2\left(tm\right)\end{matrix}\right.\\ d,ĐK:x\ge3\\ PT\Leftrightarrow3\sqrt{x-3}+\dfrac{1}{2}\cdot2\sqrt{x-3}-9\cdot\dfrac{1}{3}\sqrt{x-3}=2\\ \Leftrightarrow\sqrt{x-3}=2\\ \Leftrightarrow x-3=4\Leftrightarrow x=7\left(tm\right)\)

`(5sqrt{1/5}+1/2sqrt{20}-5/4sqrt{4/5}+sqrt{5}):2/5
`=(sqrt5+1/2*2sqrt5-sqrt{5/4}+sqrt5):2/5`
`=(sqrt5+sqrt5+sqrt5-sqrt5/2):2/5`
`=(5/2*sqrt5):2/5`
`=25/4sqrt5`
`1/3sqrt{48}+3sqrt{75}-sqrt{27}-10sqrt{1 1/3}`
`=1/3*4sqrt3+3*5sqrt3-3sqrt3-10sqrt{4/3}`
`=4/sqrt3+15sqrt3-3sqrt3-20/sqrt3`
`=12sqrt3-16/sqrt3`

Bài 1:
a) Ta có: \(\left(5\sqrt{\dfrac{1}{5}}+\dfrac{1}{2}\sqrt{20}-\dfrac{5}{4}\sqrt{\dfrac{4}{5}}+\sqrt{5}\right)\)
\(=\left(\sqrt{5}+\sqrt{5}-\dfrac{5}{4}\cdot\dfrac{2}{\sqrt{5}}+\sqrt{5}\right)\)
\(=3\sqrt{5}-\dfrac{1}{2}\sqrt{5}\)
\(=\dfrac{5}{2}\sqrt{5}\)
c) Ta có: \(\dfrac{5\sqrt{7}-7\sqrt{5}+2\sqrt{70}}{\sqrt{35}}\)
\(=\dfrac{\sqrt{35}\left(\sqrt{5}-\sqrt{7}+2\sqrt{2}\right)}{\sqrt{35}}\)
\(=2\sqrt{2}+\sqrt{5}-\sqrt{7}\)
Bài 2:
e) ĐKXĐ: \(\dfrac{4}{3}\le x\le6\)
Ta có: \(\sqrt{6-x}=3x-4\)
\(\Leftrightarrow6-x=\left(3x-4\right)^2\)
\(\Leftrightarrow9x^2-24x+16+6-x=0\)
\(\Leftrightarrow9x^2-25x+22=0\)
\(\Delta=\left(-25\right)^2-4\cdot9\cdot22=625-792< 0\)
Vậy: Phương trình vô nghiệm

đề là rút gọn các biểu thức sau
nhờ mọi người giải giúp mình. cảm ơn mn nhìu
a: \(=\dfrac{\sqrt{6-2\sqrt{5}}\left(3+\sqrt{5}\right)}{2\left(\sqrt{5}+1\right)}\)
\(=\dfrac{\left(\sqrt{5}-1\right)\left(3+\sqrt{5}\right)}{2\left(\sqrt{5}+1\right)}=\dfrac{3\sqrt{5}+5-3-\sqrt{5}}{2\sqrt{5}+2}\)
\(=\dfrac{2\sqrt{5}+2}{2\sqrt{5}+2}=1\)
b: \(=\dfrac{2\sqrt{5}\left(\sqrt{5}+\sqrt{2}\right)}{\sqrt{5}+\sqrt{2}}-2-2\sqrt{5}\)
=2căn 5-2-2căn 5
=-2
d: \(=\dfrac{\sqrt{2}}{2+\sqrt{3}+1}+\dfrac{\sqrt{2}}{2-\sqrt{3}+1}\)
\(=\dfrac{\sqrt{2}}{3+\sqrt{3}}+\dfrac{\sqrt{2}}{3-\sqrt{3}}\)
\(=\dfrac{3\sqrt{2}-\sqrt{6}+3\sqrt{2}+\sqrt{6}}{6}=\sqrt{2}\)

\(1,=2\sqrt{3}-3\sqrt{3}+4\sqrt{3}=3\sqrt{3}\\ 2,=\left(2\sqrt{6}+2\sqrt{5}-4\sqrt{5}\right):5=\dfrac{2\sqrt{6}}{5}-\dfrac{2\sqrt{5}}{5}\\ 3,=6\sqrt{3}-\dfrac{4\sqrt{3}}{3}-4\sqrt{3}-\dfrac{5\sqrt{3}}{3}=2\sqrt{3}-\dfrac{9\sqrt{3}}{3}=2\sqrt{3}-3\sqrt{3}=-\sqrt{3}\\ 4,Sửa:\dfrac{1}{\sqrt{5}-\sqrt{3}}-\dfrac{1}{\sqrt{5}+\sqrt{3}}\\ =\dfrac{\sqrt{5}+\sqrt{3}-\sqrt{5}+\sqrt{3}}{\left(\sqrt{5}-\sqrt{3}\right)\left(\sqrt{5}+\sqrt{3}\right)}=\dfrac{2\sqrt{3}}{2}=\sqrt{3}\)
1) \(=2\sqrt{3}-3\sqrt{3}+4\sqrt{3}=3\sqrt{3}\)
2) \(=\left(2\sqrt{6}+2\sqrt{5}-4\sqrt{5}\right)=\dfrac{2\sqrt{6}}{5}+\dfrac{2\sqrt{5}}{5}-\dfrac{4\sqrt{5}}{5}\)
3) \(=6\sqrt{3}-\dfrac{4\sqrt{3}}{3}-4\sqrt{3}-\dfrac{5\sqrt{3}}{3}=2\sqrt{3}-3\sqrt{3}=-\sqrt{3}\)
4) \(=\dfrac{\sqrt{5}+\sqrt{3}-\sqrt{5}+\sqrt{3}}{5-3}=\dfrac{2\sqrt{3}}{2}=\sqrt{3}\)
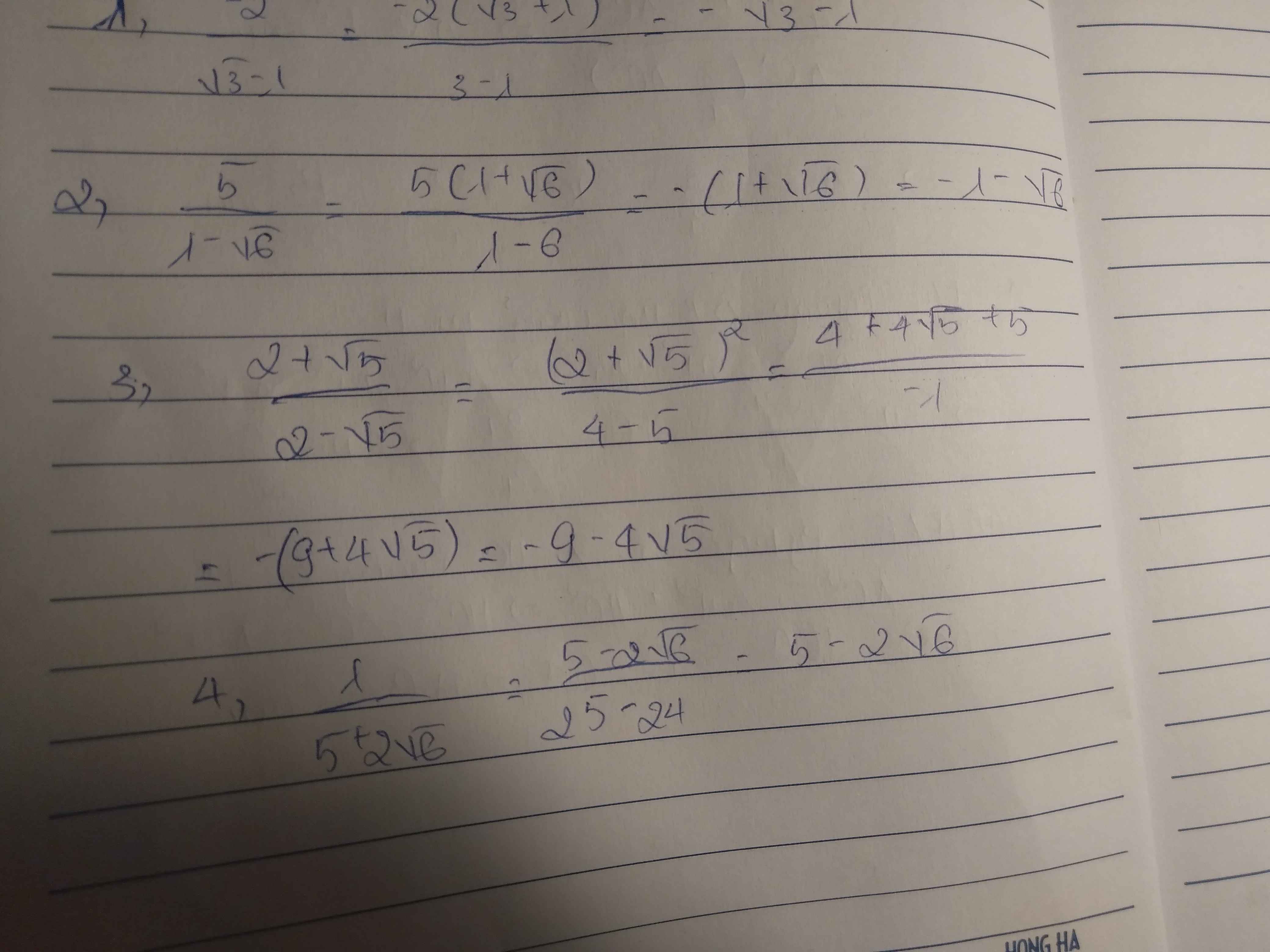
\(5\sqrt{\dfrac{1}{5}}-\dfrac{8}{1+\sqrt{5}}-\dfrac{\sqrt{20}-5}{2-\sqrt{5}}=\dfrac{5\sqrt{5}}{5}-\dfrac{8\left(\sqrt{5}-1\right)}{\left(\sqrt{5}-1\right)\left(\sqrt{5}+1\right)}-\dfrac{\sqrt{5}\left(2-\sqrt{5}\right)}{2-\sqrt{5}}=\sqrt{5}-\dfrac{8\left(\sqrt{5}-1\right)}{4}-\sqrt{5}=-\dfrac{8\left(\sqrt{5}-1\right)}{4}=-2\left(\sqrt{5}-1\right)=2-2\sqrt{5}\)