1:Giải phương trình( giải chi tiết giúp mình với...
Đọc tiếp
1:Giải phương trình( giải chi tiết giúp mình với ạ)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
LB
1
Những câu hỏi liên quan
NV
Nguyễn Việt Lâm
Giáo viên
23 tháng 1 2024
ĐKXĐ: \(0\le x\le9\)
Bình phương 2 vế ta được:
\(x+9-x+2\sqrt{x\left(9-x\right)}=-x^2+9x+9\)
\(\Leftrightarrow-x^2+9x-2\sqrt{-x^2+9x}=0\)
\(\Leftrightarrow\sqrt{-x^2+9x}\left(\sqrt{-x^2+9x}-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{-x^2+9x}=0\\\sqrt{-x^2+9x}=2\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}-x^2+9x=0\\-x^2+9x-4=0\end{matrix}\right.\)
Tới đây em tự hoàn thành nốt
19 tháng 8 2021
Bài 1:
ĐKXĐ: \(x\ge\dfrac{1}{2}\)
Ta có: \(\sqrt{5x^2}=2x-1\)
\(\Leftrightarrow5x^2=\left(2x-1\right)^2\)
\(\Leftrightarrow5x^2-4x^2+4x-1=0\)
\(\Leftrightarrow x^2+4x-1=0\)
\(\text{Δ}=4^2-4\cdot1\cdot\left(-1\right)=20\)
Vì Δ>0 nên phương trình có hai nghiệm phân biệt là:
\(\left\{{}\begin{matrix}x_1=\dfrac{-4-2\sqrt{5}}{2}=-2-\sqrt{5}\left(loại\right)\\x_2=\dfrac{-4+2\sqrt{5}}{2}=-2+\sqrt{5}\left(loại\right)\end{matrix}\right.\)
AH
Akai Haruma
Giáo viên
19 tháng 8 2021
Bài 1: Bình phương hai vế lên có giải ra được kết quả. Nhưng phải kèm thêm điều kiện $2x-1\geq 0$ do $\sqrt{5x^2}\geq 0$
PT \(\Leftrightarrow \left\{\begin{matrix} 2x-1\geq 0\\ 5x^2=(2x-1)^2\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\geq \frac{1}{2}\\ x^2+4x-1=0\end{matrix}\right.\)
\(\Leftrightarrow \left\{\begin{matrix} x\geq \frac{1}{2}\\ (x+2)^2-5=0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\geq \frac{1}{2}\\ (x+2-\sqrt{5})(x+2+\sqrt{5})=0\end{matrix}\right.\)
\(\Leftrightarrow \left\{\begin{matrix} x\geq \frac{1}{2}\\ x=-2\pm \sqrt{5}\end{matrix}\right.\) (vô lý)
Vậy pt vô nghiệm.
NN
1
HP
19 tháng 3 2021
ĐK: \(x\ge0\)
Dễ thấy \(1-\sqrt{2\left(x^2-x+1\right)}\le1-\sqrt{2}< 0\)
Khi đó bất phương trình tương đương:
\(x-\sqrt{x}\le1-\sqrt{2\left(x^2-x+1\right)}\)
\(\Leftrightarrow\sqrt{x}-\dfrac{1}{\sqrt{x}}-1+\sqrt{2\left(x+\dfrac{1}{x}-1\right)}\le0\)
\(\Leftrightarrow\sqrt{x}-\dfrac{1}{\sqrt{x}}-1+\sqrt{2\left(\sqrt{x}-\dfrac{1}{\sqrt{x}}\right)^2+2}\le0\)
\(\Leftrightarrow t-1+\sqrt{2t^2+2}\le0\)
T
1
NV
Nguyễn Việt Lâm
Giáo viên
23 tháng 1 2024
ĐKXĐ: \(x\ge1\)
Đặt \(\left\{{}\begin{matrix}\sqrt[]{x-1}=a\ge0\\\sqrt[3]{2-x}=b\end{matrix}\right.\) \(\Rightarrow a^2+b^3=1\)
Ta được hệ:
\(\left\{{}\begin{matrix}a+b=1\\a^2+b^3=1\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}b=1-a\\a^2+b^3=1\end{matrix}\right.\)
\(\Rightarrow a^2+\left(1-a\right)^3=1\)
\(\Leftrightarrow a^3-4a^2+3a=0\)
\(\Leftrightarrow a\left(a-1\right)\left(a-3\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}a=0\\a=1\\a=3\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}\sqrt[]{x-1}=0\\\sqrt[]{x-1}=1\\\sqrt[]{x-1}=3\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=1\\x=2\\x=10\end{matrix}\right.\)
8 tháng 5 2022
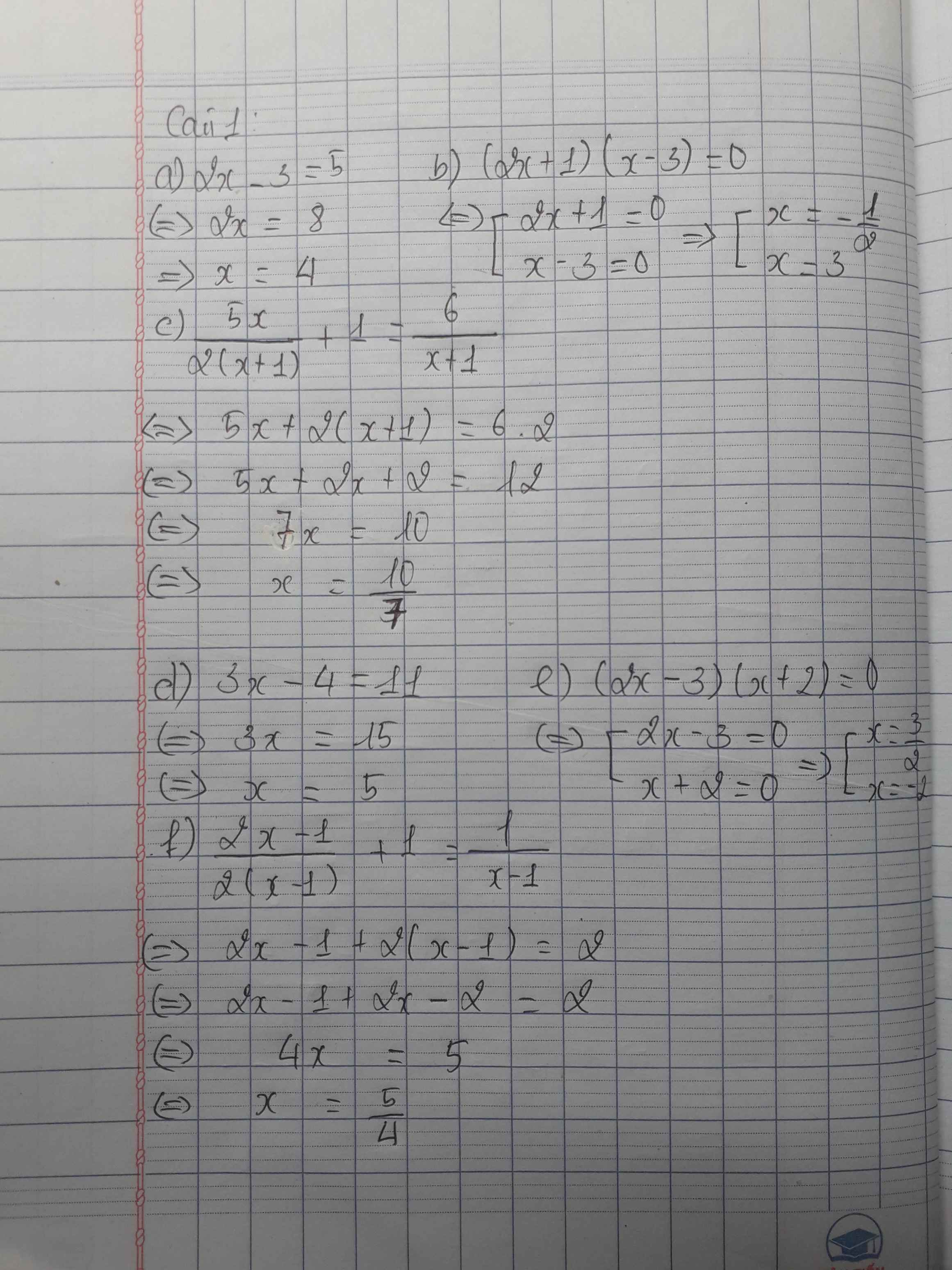
Câu 1:
a)2x-3=5
\(\leftrightarrow\)2x=5+3
\(\leftrightarrow\)2x=8
\(\leftrightarrow\)x=4
Vậy pt có tập nghiệm S={4}
b)(2x+1)(x-3)=0
\(\leftrightarrow\) 2x+1=0
Hoặc x-3=0
\(\leftrightarrow\)x=-1/2
x=3
Vậy pt có tập nghiệm S={-1/2;3}
d)3x-4=11
\(\leftrightarrow\)3x=11+4
\(\leftrightarrow\)3x=15
\(\leftrightarrow\)x=5
Vậy pt có tập nghiệm S={5}
e)(2x-3)(x+2)=0
\(\leftrightarrow\)2x-3=0
Hoặc x+2=0
\(\leftrightarrow\)x=3/2
hoặc x=-2
Vậy pt có tập nghiệm S={3/2;-2}
Câu 2:
a)2x-3<15
\(\leftrightarrow\)2x<15+3
\(\leftrightarrow\)2x<18
\(\leftrightarrow\)x<9
Vật bpt có tập nghiệm S={x|x<9}
c)5x-2<18
\(\leftrightarrow\)5x<20
\(\leftrightarrow\)x<4
Vậy bpt có tập nghiệm S={x|x<4}
Mấy bài phân số nhác gõ quá~
14 tháng 3 2022
Ta có:
(2 - 3x)(x + 8) = (3x - 2)(3 - 5x)
⇔ (2 - 3x)(x + 8) - (3x - 2)(3 - 5x) = 0
⇔ (2 - 3x)(x + 8) + (2 - 3x)(3 - 5x) = 0
⇔ (2 - 3x)(x + 8 + 3 - 5x) = 0
⇔ (2 - 3x)(11 - 4x) = 0
⇔ 2 - 3x = 0 hay 11 - 4x = 0
⇔ 2 = 3x hay 11 = 4x
⇔ x = \(\dfrac{2}{3}\) hay x = \(\dfrac{11}{4}\)
Vậy tập nghiệm của pt S = \(\left\{\dfrac{2}{3};\dfrac{11}{4}\right\}\)
14 tháng 3 2022
<=> (2-3x ) (x+8) + (2-3x ) (3-5x)=0
<=> (2-3x ) ( x+8 + 3-5x ) =0
<=> (2-3x ) ( 11 - 4x ) = 0
=> 2-3x =0 hoặc 11-4x =0
3x = 2 4x =11
x = 2/3 x = 11/4
HL
0
LL
4 tháng 6 2021
gọi x là vận tốc của ô tô
y là vận tốc của xe máy (km/h) (x>y>0)
sau 4h 2 xe gặp nhau nên tổng quãng đường AB bằng:
AB= 4.x+4.y = 4.(x+y) (km)
nên thgian ô tô và xe máy đi hết AB lần lượt là:
\(\dfrac{4\left(x+y\right)}{y}\)(h); \(\dfrac{4\left(x+y\right)}{x}\) (h)
vì ô tô đến sớm hơn xe máy 6h nên ta có pt:
\(\dfrac{4\left(x+y\right)}{y}\)-\(\dfrac{4\left(x+y\right)}{x}\)=6
⇔\(\dfrac{4x+4y}{y}\)-\(\dfrac{4x+4y}{x}\)=6
⇔4.\(\dfrac{x}{y}\) +4-4-\(\dfrac{4y}{x}\)=6
⇔\(\dfrac{x}{y}\)-\(\dfrac{y}{x}\)=\(\dfrac{6}{4}\)=\(\dfrac{3}{2}\)
đặt: t=\(\dfrac{x}{y}\) (t>0)
⇒t-\(\dfrac{1}{t}\)=\(\dfrac{3}{2}\)
⇔t2-\(\dfrac{3}{2}\)t-1=0
⇔(t -2)(t +\(\dfrac{1}{2}\))=0
⇔t=2
⇒\(\dfrac{x}{y}\)=2 ⇒x=2y
⇒AB=4.(x+y)=6x=12y
nên thgian ô tô và xe máy đi hết AB lần lượt là:
\(\dfrac{6x}{x}=6\) (h)\(\dfrac{12y}{y}=12\) (h)
5 tháng 6 2021
Gọi thời gian xe máy đi hết quãng đường AB là x (h) (x>4)
thời gian xe máy đi hết quãng đường AB là y (h) (y>4)
Trong 1 giờ xe máy đi được \(\dfrac{1}{x}\) (quãng đường)
Trong 1 giờ ô tô đi được \(\dfrac{1}{y}\) (quãng đường)
Trong 1 giờ hai xe đi được \(\dfrac{1}{x}+\dfrac{1}{y}=\dfrac{1}{4}\left(1\right)\)
Mà thời gian ô tô về đến A sớm hơn xe máy về đến B là 6 giờ nên: \(x-y=6\left(2\right)\)
Từ (1) và (2) ta có hệ phương trình:
\(\left\{{}\begin{matrix}\dfrac{1}{x}+\dfrac{1}{y}=\dfrac{1}{4}\\x-y=6\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{1}{x}+\dfrac{1}{x-6}=\dfrac{1}{4}\\y=x-6\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x^2-14x+24=0\\y=2-6\end{matrix}\right.\)(ĐK:\(x\ne6\)) \(\Leftrightarrow\left\{{}\begin{matrix}x=12\\y=6\end{matrix}\right.\)(TM)
Vậy thời gian xe máy đi hết quãng đường AB là 12 giờ
thời gian ô tô đi hết quãng đường AB là 6giờ
-Chúc bạn học tốt-
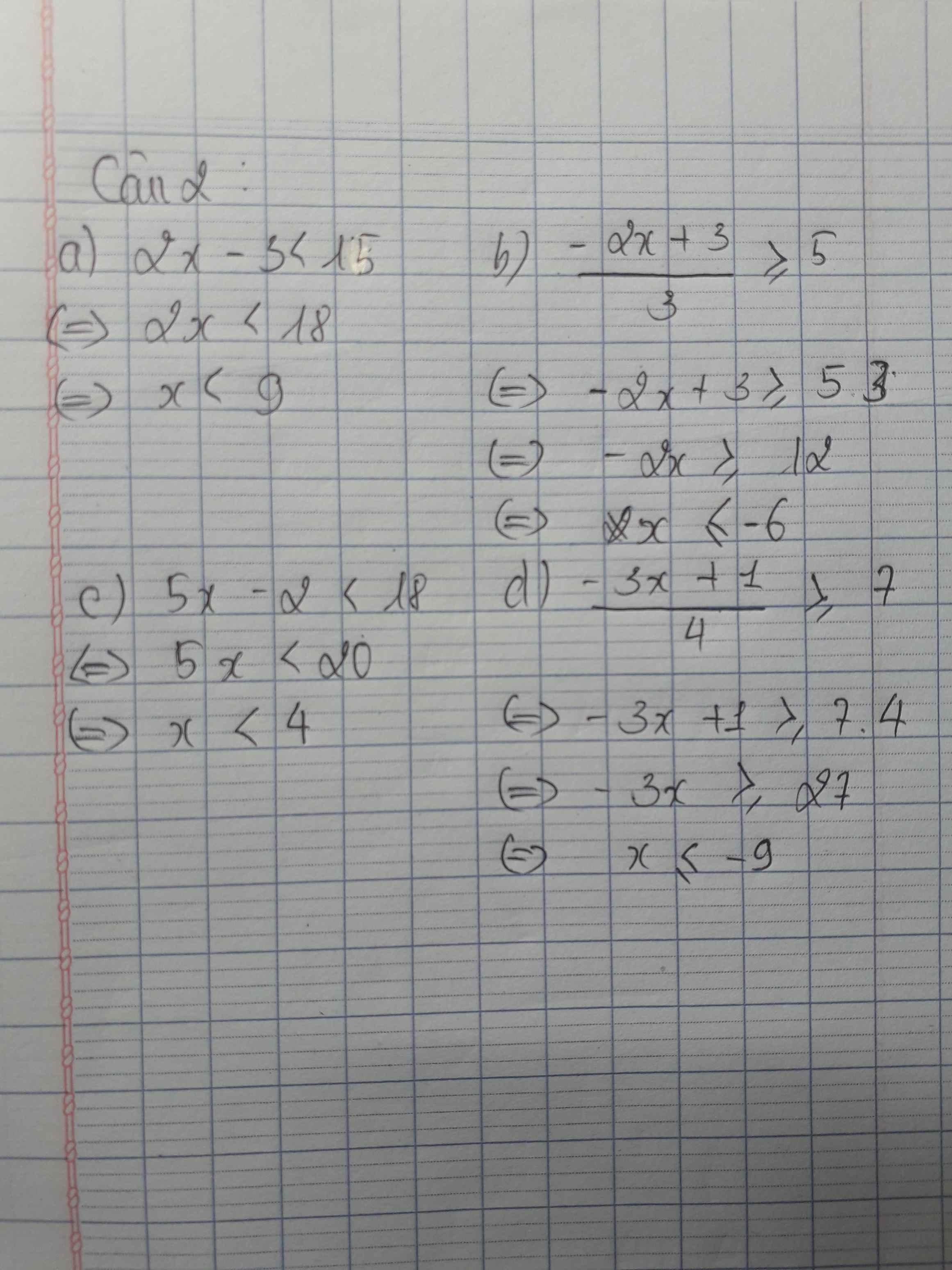
a. \(\sqrt{4x}+\sqrt{x}=2\Leftrightarrow2\sqrt{x}+\sqrt{x}=2\Leftrightarrow3\sqrt{x}=2\Leftrightarrow\sqrt{x}=\frac{2}{3}\Leftrightarrow x=\frac{4}{9}\)
b. \(\sqrt{x^2-4}=\sqrt{x-2}\Leftrightarrow\hept{\begin{cases}x^2-4=x-2\\x-2\ge0\end{cases}}\Leftrightarrow\hept{\begin{cases}\orbr{\begin{cases}x=2\\x=-1\end{cases}}\\x\ge2\end{cases}}\Leftrightarrow x=2\)\(\sqrt{x^2-4}=\sqrt{x-2}\Leftrightarrow\hept{\begin{cases}x^2-4=x-2\\x-2\ge2\end{cases}}\Leftrightarrow\hept{\begin{cases}\left(x-2\right)\left(x+1\right)=0\\x\ge2\end{cases}}\Leftrightarrow x=2\)
c.\(\sqrt{x^2-2x}+\sqrt{2x^2+4x}=2x\Leftrightarrow\hept{\begin{cases}x\ge0\\x^2-2x+2x^2+4x+2\sqrt{x^2-2x}.\sqrt{2x^2+4x}=4x^2\end{cases}}\)
\(\Rightarrow x^2-2x=2\sqrt{x^2-2x}.\sqrt{2x^2+4x}\Leftrightarrow\orbr{\begin{cases}\sqrt{x^2-2x}=0\\\sqrt{x^2-2x}=2\sqrt{2x^2+4x}\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=0\text{ hoặc }x=2\\x^2-2x=8x^2+16x\end{cases}\Leftrightarrow}\)hoặc x=0 hoặc x=2 hoặc x= -18/7
Kết hợp điều kiện ta có : \(x=0\text{ hoặc }x=2\)
d. Điều kiện \(x\ge3\) ta có :
\(\sqrt{x^2+2x-15}=\sqrt{x-3}+\sqrt{x^2-3x}\Leftrightarrow x^2+2x-15=x^2-2x-3+2\sqrt{x-3}\sqrt{x^2-3x}\)
\(\Leftrightarrow2x-6=\sqrt{x-3}.\sqrt{x^2-3x}\Leftrightarrow4\left(x-3\right)^2=\left(x-3\right)\left(x^2-3x\right)\Leftrightarrow\orbr{\begin{cases}x=3\\x=4\end{cases}}\)