tìm x biết 2x^2+3x-27=0
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a) x = 1; x = - 1 3 b) x = 2.
c) x = 3; x = -2. d) x = -3; x = 0; x = 2.

a) 22 + (2x -13) = 83 => 2x -13 = 61 => x = 37.
b) 51 - (-12 + 3x) = 27 => 63 - 3x = 27 => x = 12.
c) - (2x + 2) + 21 = - 23 => 2x + 2 = 44 => x = 21.
d) 25 - (25 - x) = 0 => 25 - 25 + x = 0 => x = 0.

a) \(\left(2x+\frac{3}{5}\right)^2-\frac{9}{25}=0\)
\(\left(2x+\frac{3}{5}\right)^2=\frac{9}{25}\)
\(\left(2x+\frac{3}{5}\right)^2=\left(\frac{3}{5}\right)^2\)
\(=>2x+\frac{3}{5}=\frac{3}{5}\)
\(2x=\frac{3}{5}-\frac{3}{5}\)
\(2x=0\)
\(x=0:2\)
\(x=0\)
b) \(\left(3x-1\right).\left(-\frac{1}{2x}+5\right)=0\)
=> \(\left(3x-1\right)=0\)hoặc \(\left(-\frac{1}{2x}+5\right)=0\)hoặc \(\left(3x-1\right)\)và\(\left(-\frac{1}{2x}+5\right)\)cùng bằng 0.
\(\orbr{\begin{cases}3x-1=0\\-\frac{1}{2x}+5=0\end{cases}}=>\orbr{\begin{cases}3x=1\\-\frac{1}{2x}=-5\end{cases}}=>\orbr{\begin{cases}x\in\varnothing\\2x=\frac{1}{5}\end{cases}}=>x=\frac{1}{5}:2=>x=\frac{1}{10}\)

a ) \(9x^2-49=9\)
\(\Leftrightarrow9x^2=58\)
\(\Leftrightarrow x^2=29\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}x=29\\x=-29\end{array}\right.\)
Vậy ......................
b ) \(\left(x+3\right)\left(x^2-3x+9\right)-x\left(x-1\right)\left(x+1\right)-27=0\)
\(\Leftrightarrow\left(x^3+3^3\right)-x.\left(x^2-1^2\right)-27=0\)
\(\Leftrightarrow x^3+27-x^3+x-27=0\)
\(\Leftrightarrow x=0\)
c ) \(\left(x-1\right)\left(x+2\right)-x-2=0\)
\(\Leftrightarrow x^2+2x-x-2-x-2=0\)
\(\Leftrightarrow x^2-4=0\)
\(\Leftrightarrow x^2=4\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}x=2\\x=-2\end{array}\right.\)
Vây .....................

a, \(3x^2-3x+2x^3-2x^2=0\)
\(\Rightarrow3x.\left(x-1\right)+2x^2.\left(x-1\right)=0\)
\(\Rightarrow\left(x-1\right).\left(3x+2x^2\right)=0\)
\(\Rightarrow\left(x-1\right).x.\left(3+2x\right)=0\)
\(\Rightarrow\left\{{}\begin{matrix}x-1=0\\x=0\\3+2x=0\end{matrix}\right.\Rightarrow\left\{{}\begin{matrix}x=1\\x=0\\x=\dfrac{-3}{2}\end{matrix}\right.\)
Vậy......
Câu b tương tự!!
a, \(3x^2-3x+2x^3-2x^2=0\)
\(\Leftrightarrow3x\left(x-1\right)+2x^2\left(x-1\right)=0\)
\(\Leftrightarrow\left(3x+2x^2\right)\left(x-1\right)=0\)
\(\Leftrightarrow x\left(3+2x\right)\left(x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\3+2x=0\\x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=\dfrac{-2}{3}\\x=1\end{matrix}\right.\)
Vậy...
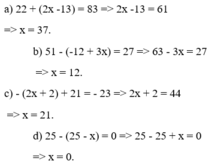
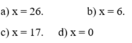
2x^2+3x-27=0
2x^2-6x+9x-27=0
2x(x-3)+9(x-3)=0
(2x-9)(x-3)=0
x-3=0, 2x-9=0
x=3 hay x=9/2
2x2+3x-27=0
<=>2x2-6x+9x-27=0
<=>2x.(x-3)+9.(x-3)=0
<=>(x-3)(2x+9)=0
<=>x-3=0 hoặc 2x+9=0
<=>x=3 hoặc x=-9/2