Giải phương trình
x^17=1
x mủ 17 bằng một
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



a, Khi \(x = 0 ⇔ 0! + y! = y! ⇔ \) Vô lý.
\(\rightarrow x \ne y\)\(\ne 0\)
Khi \(x = y \rightarrow 2 . x! = (2x)! \rightarrow 2x! = x(x+1)(x+2)...(2x)=>x(x+1)(x+2)...(2x) = 2 \rightarrow x = y = 1. \)
Nếu \(x \ne y \rightarrow\) Vì vai trò của \(x,y\) là bình đẳng nên giả sử \(x < y\)
\(\rightarrow x!+y!<2.y!≤(y+1).y!=(y+1)!<(x+y)!\)
Vì \(x \ne y \ne 1 => (x+y) \ne (y+1) \rightarrow (x+y)! \ne (y+1).\)
Vậy \((x,y) = {(1,1)}.\)
b, Chứng minh bằng phương pháp phản chứng:
Giả sử \(x^{17} + y^{17} = 19^{17} \) có nghiệm nguyên.
Không mất tổng quát, giả sử \(x < y\)
\(\rightarrow x^{17} < y^{17} ≤ 19^{17}\)
\(\rightarrow (y+1)^{17} ≤ 19^{17} \)
\(\rightarrow y^{17} + 17y^{16} = 19^{17}\)
Mà \(\rightarrow x > 17 \rightarrow x = y =18.\)
Thử lại không đúng, suy ra giả sử sai.
\(\rightarrow\) Không tồn tại số nguyên thỏa mãn.

\(2x^4-10x^2+17=2\left(x^4-5x^2+\frac{25}{4}\right)+\frac{9}{2}=2\left(x^2-\frac{5}{2}\right)^2+\frac{9}{2}>0\left(vl\right)\)
=> PT vô nghiệm
\(x^4-x^3+2x^2-x+1=x^2\left(x^2-x+1\right)+x^2-x+1=\left(x^2-x+1\right)\left(x^2+1\right)=\left(x^2+1\right)\left(\left(x-\frac{1}{2}\right)^2+\frac{3}{4}\right)>0\forall x\)=> Pt vô nghiệm


Đáp án D
Ta có:
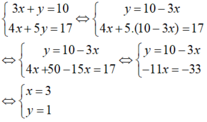
Vậy nghiệm của hệ phương trình đã cho là: (3; 1).

ĐKXĐ: \(x\ge15\)
Đặt \(\sqrt{x-15}=t\ge0\Rightarrow x=t^2+15\)
Pt trở thành:
\(t^2+15-t=17\Leftrightarrow t^2-t-2=0\)
\(\Leftrightarrow\left[{}\begin{matrix}t=-1< 0\left(loại\right)\\t=2\end{matrix}\right.\)
\(\Rightarrow\sqrt{x-15}=2\Rightarrow x=19\)

Đk:\(-\sqrt{17}\le x\le\sqrt{17}\)
Đặt \(t=x+\sqrt{17-x^2}\left(t>0\right)\)
\(\Rightarrow t^2=17+2x\sqrt{17-x^2}\)
\(\Rightarrow x\sqrt{17-x^2}=\frac{t^2-17}{2}\)
thay vào pt
\(t+\frac{t^2-17}{2}=9\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}t=-7\left(loai\right)\\t=5\left(tm\right)\end{array}\right.\)
\(\Rightarrow x+\sqrt{17-x^2}=5\)
\(\Leftrightarrow\sqrt{17-x^2}=5-x\)
Với \(x< \sqrt{17}\) bình 2 vế ta có:
\(17-x^2=x^2-10x+25\)
\(\Leftrightarrow2x^2-10x+8=0\)
\(\Leftrightarrow\left[\begin{array}{nghiempt}x=1\\x=4\end{cases}\left(tm\right)}\)
dòng cuối là \(\Leftrightarrow\left[\begin{array}{nghiempt}x=1\\x=4\end{array}\right.\)(thỏa mãn)
Ta có:\(x^{17}=1\)
\(\Rightarrow x^{17}=1^{17}\)
\(\Rightarrow x=1\)