bài 5 : tìm sỗ nguyên x, biết:
a/ 5 . \(3^x\) = 5 . \(3^4\)
b/ 7 . \(4^x\) = 7 . \(4^3\)
c/ \(\dfrac{3}{5}\) . \(4^x\) = 7 . \(4^3\)
d/ \(\dfrac{3}{2}\) . \(5^x\) = \(\dfrac{3}{2}\) . \(5^{12}\)
e/ 9 . \(5^x\) = 6 . \(5^6\) + 3 . \(5^6\)
f/ 5 . \(3^x\) = 7 . \(3^5\) - 2 . \(3^5\)
g/ 5 . \(3^{x+6}\) = 2 . \(3^5\)+ 3 . \(3^5\)

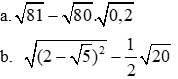
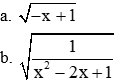
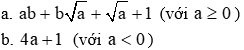
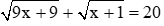
\(a.5\cdot3^x=5\cdot3^4\\ =>3^x=\dfrac{5\cdot3^4}{5}=3^4\\ =>x=4\\ b.7\cdot4^x=7\cdot4^3\\ =>4^x=\dfrac{7\cdot4^3}{7}=4^3\\ =>x=3\\ c.\dfrac{3}{5}\cdot4^x=7\cdot4^3\\ =>4^x=\dfrac{7\cdot4^3}{\dfrac{3}{5}}=\dfrac{35}{3}\cdot4^3\\ =>\dfrac{4^x}{4^3}=\dfrac{35}{3}\\ =>4^{x-3}=\dfrac{35}{3}\\ =>x-3=log_4\dfrac{35}{3}\\ =>x=log_4\dfrac{35}{3}+3\\ d.\dfrac{3}{2}\cdot5^x=\dfrac{3}{2}\cdot5^{12}\\ =>5^x=\dfrac{5^{12}\cdot\dfrac{3}{2}}{\dfrac{3}{2}}=5^{12}\\ =>x=12\)
e: \(9\cdot5^x=6\cdot5^6+3\cdot5^6\)
=>\(9\cdot5^x=9\cdot5^6\)
=>\(5^x=5^6\)
=>x=6
f: \(5\cdot3^x=7\cdot3^5-2\cdot3^5\)
=>\(5\cdot3^x=5\cdot3^5\)
=>\(3^x=3^5\)
=>x=5
g: \(5\cdot3^{x+6}=2\cdot3^5+3\cdot3^5\)
=>\(5\cdot3^{x+6}=5\cdot3^5\)
=>\(3^{x+6}=3^5\)
=>x+6=5
=>x=-1