Cần gấp bài 14,15 và bài 16 câu b ạ. Mình cảm ơn!!!
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


1b)
Song song => (d): x-y +a =0
Vì d đi qua C(2;-2) => 2- (-2)+a=0
<=>a=4
=> d: x-y+4=0


1) Vì x=25 thỏa mãn ĐKXĐ nên Thay x=25 vào biểu thức \(A=\dfrac{\sqrt{x}-2}{x+1}\), ta được:
\(A=\dfrac{\sqrt{25}-2}{25+1}=\dfrac{5-2}{25+1}=\dfrac{3}{26}\)
Vậy: Khi x=25 thì \(A=\dfrac{3}{26}\)
2) Ta có: \(B=\dfrac{\sqrt{x}-3}{\sqrt{x}+1}+\dfrac{2x+8\sqrt{x}-6}{x-\sqrt{x}-2}\)
\(=\dfrac{\left(\sqrt{x}-3\right)\left(\sqrt{x}-2\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}+\dfrac{2x+8\sqrt{x}-6}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{x-5\sqrt{x}+6+2x+8\sqrt{x}-6}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{3x+3\sqrt{x}}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{3\sqrt{x}\left(\sqrt{x}+1\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{3\sqrt{x}}{\sqrt{x}-2}\)

Câu 4:
D và F cùng nhìn AC dưới 1 góc vuông nên tứ giác ACDF nội tiếp
\(\Rightarrow\widehat{ADF}=\widehat{ACF}\) (cùng chắn AF)
Tương tự, ABDE nội tiếp \(\Rightarrow\widehat{ABE}=\widehat{ADE}\) (cùng chắn AE)
Lại có \(\widehat{ABE}=\widehat{ACF}\) (cùng phụ góc \(\widehat{A}\))
\(\Rightarrow\widehat{ADE}=\widehat{ADF}\) hay AD là phân giác góc \(\widehat{FDE}\)
./
Hoàn toàn tương tự, ta cũng có CF là phân giác \(\widehat{DFE}\Rightarrow\widehat{BFD}=\widehat{AFE}\)
Mà \(\widehat{AFE}=\widehat{BFK}\Rightarrow\widehat{BFK}=\widehat{BFD}\)
\(\Rightarrow\dfrac{BK}{BD}=\dfrac{FK}{FD}\) theo định lý phân giác
Đồng thời \(\dfrac{CK}{CD}=\dfrac{FK}{FD}\) (CF là phân giác ngoài góc \(\widehat{DFK}\))
\(\Rightarrow\dfrac{BK}{BD}=\dfrac{CK}{CD}\Rightarrow\dfrac{BK}{CK}=\dfrac{BD}{CD}\)
Qua B kẻ đường thẳng song song AC cắt AK và AD tại P và Q
Theo Talet: \(\dfrac{BK}{CK}=\dfrac{BP}{AC}\) đồng thời \(\dfrac{BD}{DC}=\dfrac{BQ}{AC}\)
\(\Rightarrow\dfrac{BP}{AC}=\dfrac{BQ}{AC}\Rightarrow BP=BQ\)
Mặt khác BP song song MF (cùng song song AC)
\(\Rightarrow\dfrac{MF}{BP}=\dfrac{AF}{AB}\) ; \(\dfrac{NF}{BQ}=\dfrac{AF}{AB}\) (Talet)
\(\Rightarrow\dfrac{MF}{BP}=\dfrac{NF}{BQ}\Rightarrow MF=NF\)








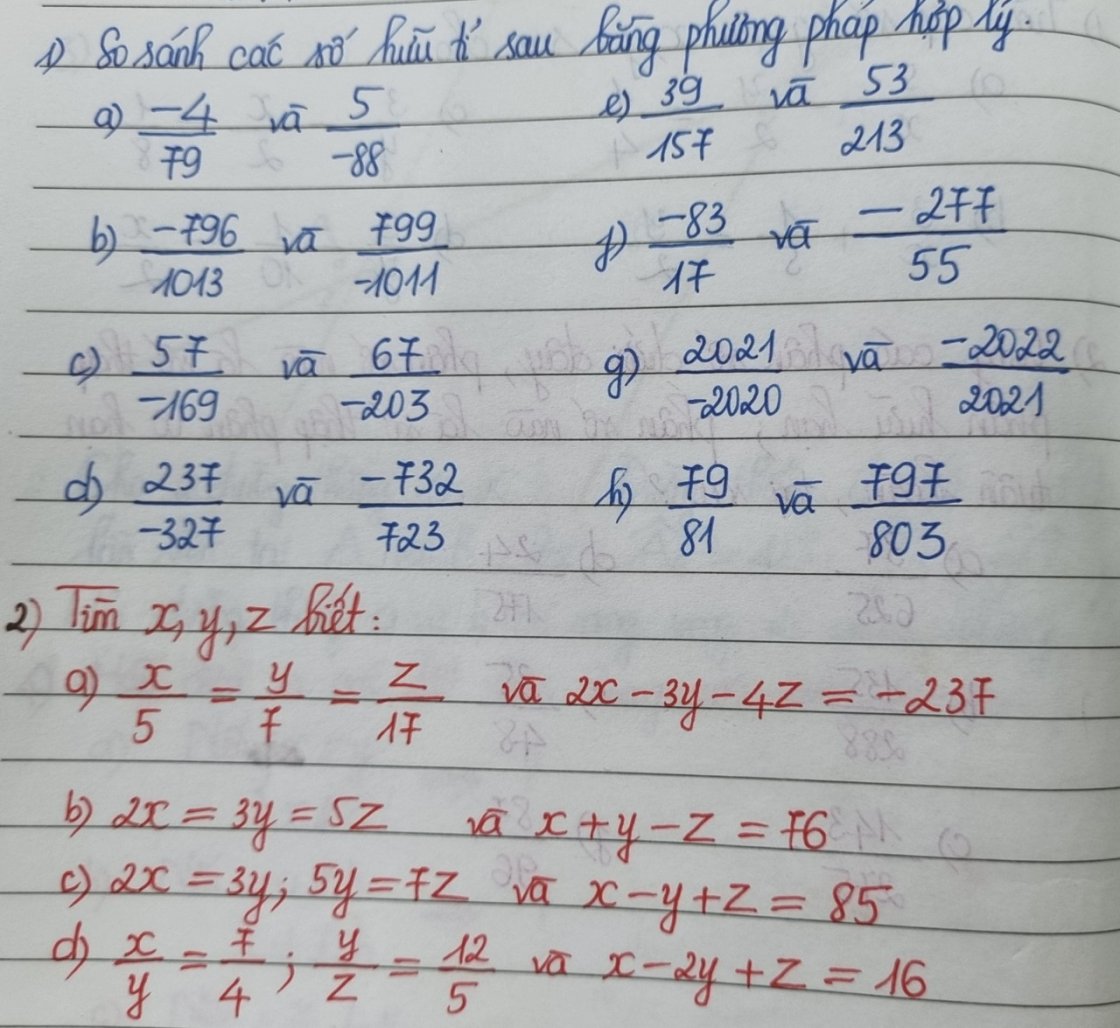
20.
a) \(A=4x^2-4x+m\)
\(=4x^2-4x+1-1+m\)
\(=\left(4x^2+4x+1\right)+\left(m-1\right)\)
\(=\left(2x+1\right)^2+\left(m-1\right)\)
Để biếu thức không âm thì \(A\ge0\Rightarrow\left(2x+1\right)^2+\left(m-1\right)\ge0\)
Mà: \(\left(2x+1\right)^2\ge0\)
\(\Rightarrow\) Để \(A\ge0\Rightarrow m-1\ge0\Leftrightarrow m\ge1\)
b) \(B=x^2-6x+2-m\)
\(=x^2-6x+9-9+2-m\)
\(=\left(x^2+6x+9\right)+\left(2-m-9\right)\)
\(=\left(x+3\right)^2+\left(-m-7\right)\)
Để bt không âm thì \(B\ge0\Rightarrow\left(x+3\right)^2+\left(-m-7\right)\ge0\)
Mà: \(\left(x+3\right)^2\ge0\)
⇒ Để \(B\ge0\Rightarrow-m-7\ge0\Leftrightarrow-m\ge7\Leftrightarrow m\le-7\)
Câu 16:
a) Số chính phương lẻ có dạng: \(\left(2x+1\right)^2=4x^2+4x+1=4x\left(x+1\right)+1\)
Vì \(x\in N\Rightarrow x\left(x+1\right)\) là tích của 2 số tự nhiên liên tiếp
\(\Rightarrow x\left(x+1\right)\) ⋮ 2
\(\Rightarrow4x\left(x+1\right)\) ⋮ 8
\(\Rightarrow4x\left(x+1\right)+1\) chia 8 dư 1
b) Theo câu a ta biết số chính phương lẻ chia 8 sẽ dư 1 mà `1^2;3^2;5^2;7^2;...;2023^2` đều là các số tự nhiên lẻ ⇒ Chúng đều chia 8 dư 1
Từ 1 đến 2023 có số lượng số lẻ là: \(\left(2023-1\right):2+1=1012\) (số)
Khi đó `1^2+3^2+5^2+...+2023^2` chia 8 dư `1*1012=1012`
Mà: 1012 chia 8 dư 4 ⇒ Tổng `1^2+3^2+5^2+...+2023^2` chia 8 dư 4