\(\dfrac{3}{5}\)x = -12 tìm tập nghiệm của phuongtrinh
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a) \(2x-\dfrac{x-3}{5}-4x+1\le0\)
\(\Leftrightarrow10x-x+3-20x+5\le0\)
\(\Leftrightarrow-11x+8\le0\)
\(\Leftrightarrow x\ge\dfrac{8}{11}\)
\(\Rightarrow x\in\left(\dfrac{8}{11};+\infty\right)\)
b) \(\sqrt{x^2+2}\le x-1\)
\(\Leftrightarrow x^2+2\le x^2-2x+1\) \(\left(x-1\ge\sqrt{x^2+2}\ge\sqrt{2}\Rightarrow x\ge1+\sqrt{2}\right)\)
\(\Leftrightarrow x\le-\dfrac{1}{2}\)
\(\Rightarrow x\in\varnothing\)
c) \(\sqrt{x-1}+\sqrt{5-x}+\dfrac{1}{x-3}>\dfrac{1}{x-3}\) (\(x\in\left[1;5\right]\backslash\left\{3\right\}\))
\(\Leftrightarrow\sqrt{x-1}+\sqrt{5-x}>0\)
\(\Leftrightarrow4+2\sqrt{\left(x-1\right)\left(5-x\right)}>0\) ( luôn đúng )
vậy \(x\in\left[1;5\right]\backslash\left\{3\right\}\)

\(\dfrac{5}{x}-\dfrac{y}{4}=\dfrac{1}{12}\Leftrightarrow\dfrac{20-xy}{4x}=\dfrac{1}{12}\Leftrightarrow240-12xy=4x\Leftrightarrow240-12xy-4x=0\Leftrightarrow60-3xy-x=0\Leftrightarrow-3xy-x=-60\Leftrightarrow-x\left(3y+1\right)=60\)Đến đây do x,y nguyên nên bạn lập bảng xét ước nhá, lưu ý 3y + 1 chia 3 dư 1 để bớt trường hợp xét nhá.

Bài 1 :
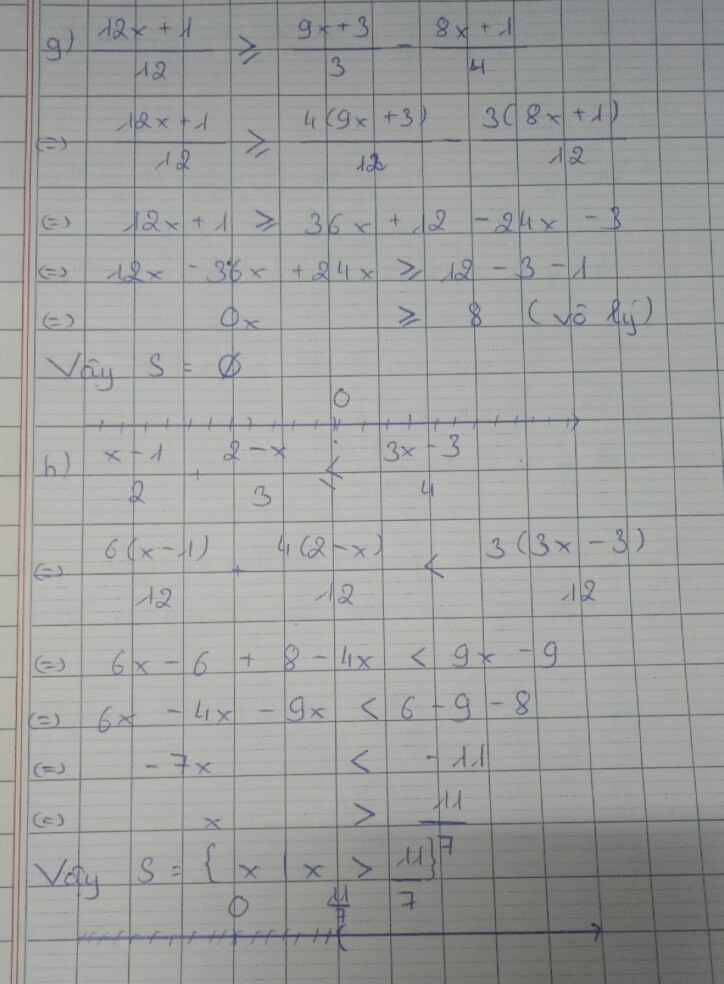
Ta có : \(\dfrac{3x+5}{2}-1\le\dfrac{x+2}{3}+x\)
\(\Leftrightarrow\dfrac{3x+5}{2}-1-\dfrac{x+2}{3}-x\le0\)
\(\Leftrightarrow\dfrac{3\left(3x+5\right)-6-2\left(x+2\right)-6x}{6}\le0\)
\(\Leftrightarrow9x+15-6-2x-4-6x\le0\)
\(\Leftrightarrow x\le-5\)
Mà \(\left\{{}\begin{matrix}x\in Z\\x>-10\end{matrix}\right.\)
Vậy \(x\in\left\{-5;-6;-7;-8;-9\right\}\)
b3\(\Leftrightarrow2x^2+5x-3-3x+1\le x^2+2x-3+x^2-5\\ \Leftrightarrow0.x\le-6\Leftrightarrow x\in\varnothing\)

\(-\dfrac{1}{2}x+6< 0\Leftrightarrow-\dfrac{1}{2}x< -6\Leftrightarrow\cdot\dfrac{1}{2}x>6\Leftrightarrow x>12\)
(sai thì thoi nha)
\(-\dfrac{1}{2}x+6< 0\)
\(\Leftrightarrow-\dfrac{1}{2}x< -6\)
\(\Leftrightarrow x>\left(-6\right):\left(-\dfrac{1}{2}\right)\)
\(\Leftrightarrow x>12\)
--> Chọn A

a) \(x-\sqrt{2x+3}=-2x\)
\(\Leftrightarrow\sqrt{2x+3}=x+2x\)
\(\Leftrightarrow\sqrt{2x+3}=3x\)
\(\Leftrightarrow2x+3=9x^2\)
\(\Leftrightarrow9x^2-2x-3=0\)
\(\Rightarrow\Delta=\left(-2\right)^2-4\cdot9\cdot\left(-3\right)=112>0\)
\(\Leftrightarrow\left[{}\begin{matrix}x_1=\dfrac{2+\sqrt{112}}{18}=\dfrac{1+2\sqrt{7}}{9}\\x_2=\dfrac{2-\sqrt{112}}{18}=\dfrac{1-2\sqrt{7}}{9}\end{matrix}\right.\)
b) \(\dfrac{1}{x}=1-\dfrac{1}{x+1}\) (ĐK: \(x\ne0,x\ne-1\))
\(\Leftrightarrow\dfrac{1}{x}+\dfrac{1}{x+1}=1\)
\(\Leftrightarrow\dfrac{x+1}{x\left(x+1\right)}+\dfrac{x}{x\left(x+1\right)}=1\)
\(\Leftrightarrow\dfrac{x+1+x}{x\left(x+1\right)}=1\)
\(\Leftrightarrow\dfrac{2x+1}{x^2+x}=1\)
\(\Leftrightarrow2x+1=x^2+1\)
\(\Leftrightarrow x^2-2x=0\)
\(\Leftrightarrow x\left(x-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\left(ktm\right)\\x-2=0\end{matrix}\right.\)
\(\Leftrightarrow x=2\left(tm\right)\)
c) \(\dfrac{2}{\sqrt{x+3}}=\dfrac{1}{\sqrt{x^2-9}}\) (ĐK: \(x\ge3\))
\(\Leftrightarrow2\sqrt{x^2-2}=\sqrt{x+3}\)
\(\Leftrightarrow\sqrt{4\left(x^2-9\right)}=\sqrt{x+3}\)
\(\Leftrightarrow4\left(x^2-9\right)=x+3\)
\(\Leftrightarrow4x^2-36=x+3\)
\(\Leftrightarrow4x^2-x-36-3=0\)
\(\Leftrightarrow4x^2-x-39=0\)
\(\Rightarrow\Delta=\left(-1\right)^2-4\cdot4\cdot\left(-39\right)=625>0\)
\(\Leftrightarrow\left[{}\begin{matrix}x_1=\dfrac{1+\sqrt{625}}{8}=\dfrac{13}{4}\left(tm\right)\\x_2=\dfrac{1-\sqrt{625}}{8}=-3\left(ktm\right)\end{matrix}\right.\)

a: TXĐ: D=[0;+\(\infty\))\{1}
Ta có: \(P=\left(\dfrac{3}{\sqrt{x}+1}-\dfrac{1}{x-1}\right):\dfrac{1}{\sqrt{x}+1}\)
\(=\dfrac{3\sqrt{x}-3-1}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\cdot\dfrac{\sqrt{x}+1}{1}\)
\(=\dfrac{3\sqrt{x}-4}{\sqrt{x}-1}\)

1) dư số 9 trước dấu lớn và cái (2) mình xin sửa đề là \(\ge3\).. mới làm được ấy: )
1)
`=>3(2x+1)-2(x-2)>18(x-3)`
`<=>6x+3-2x+4>18x-54`
`<=>-14x>-61`
`=>x<61/14`
2)
`=>12x-3(x-3)>=36-(x-3)`
`<=>12x-3x+9>=36-x+3`
`<=>10x>=30`
`<=>x>=3`
`=> T:3<=x<61/14`
Mà x là các giá trị nguyên nên x thuộc {3; 4}
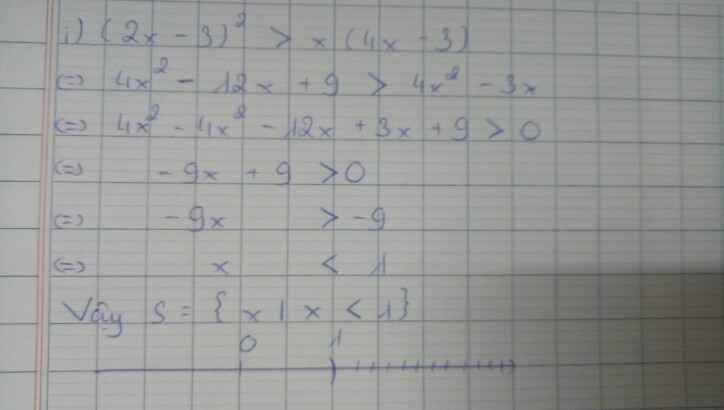
\(\dfrac{3}{5}x=-12\)
\(\Rightarrow x=-12:\dfrac{3}{5}\)
\(\Rightarrow x=-20\)