Ví dụ: Cho hai đa thức: P(x) = x²-3x²-2x²-1
và Q(x) = x²-x²+x+1. Tính P(x)-Q(x).
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
NT
1
Những câu hỏi liên quan
14 tháng 4 2022
a: \(P\left(x\right)=3x^2-x-1\)
\(Q\left(x\right)=-3x^2-4x-2\)
b: \(G\left(x\right)=3x^2-x-1+3x^2+4x+2=6x^2+3x+1\)
c: Để G(x)-6x-1=0 thì 6x2-3x=0
=>3x(2x-1)=0
=>x=0 hoặc x=1/2
18 tháng 4 2023
`P(x)=`\( 2x^4 + 3x^3 + 3x^2 - x^4 - 4x + 2 - 2x^2 + 6x\)
`= (2x^4-x^4)+3x^3+(3x^2-2x^2)+(-4x+6x)+2`
`= x^4+3x^3+x^2+2x+2`
`Q(x)=`\(x^4 + 3x^2 + 5x - 1 - x^2 - 3x + 2 + x^3\)
`= x^4+x^3+(3x^2-x^2)+(5x-3x)+(-1+2)`
`= x^4+x^3+2x^2+2x+1`
`P(x)+Q(x)=(x^4+3x^3+x^2+2x+2)+(x^4+x^3+2x^2+2x+1)`
`=x^4+3x^3+x^2+2x+2+x^4+x^3+2x^2+2x+1`
`=(x^4+x^4)+(3x^3+x^3)+(x^2+2x^2)+(2x+2x)+(2+1)`
`= 2x^4+4x^3+3x^2+4x+3`
`@`\(\text{dn inactive.}\)
18 tháng 4 2023
P(x)=x^4+3x^3+x^2+2x+2
Q(x)=x^4+x^3+2x^2+2x+1
P(x)+Q(x)=2x^4+4x^3+3x^2+4x+3
24 tháng 4 2023
a: P(x)=-5x^3+6x^2+3x-1
Q(x)=-5x^3+6x^2+4x+2
b: H(x)=-5x^3+6x^2+3x-1-5x^3+6x^2+4x+2
=-10x^3+12x^2+7x+1
T(x)=-5x^3+6x^2+3x-1+5x^3-6x^2-4x-2
=-x-3
c: T(x)=0
=>-x-3=0
=>x=-3
d: G(x)=-(-10x^3+12x^2+7x+1)
=10x^3-12x^2-7x-1
24 tháng 6 2020
a, \(P\left(x\right)=5x^3-3x+7-x\)
\(=5x^3-4x+7\)
\(Q\left(x\right)=-5x^3+2x-3+2x-x^2-2\)
\(=-5x^3-x^2+4x-5\)
Ta có \(P\left(x\right)+Q\left(x\right)=-x^2+2\)
\(P\left(x\right)-Q\left(x\right)=10x^3+x^2-8x+12\)
b, \(P\left(x\right)+Q\left(x\right)=0\)
\(\Leftrightarrow-x^2+2=0\)
\(\Leftrightarrow-x^2=-2\)
\(\Leftrightarrow x^2=2=\left(\pm\sqrt{2}\right)^2\)
\(\Rightarrow x=\pm\sqrt{2}\)
Vậy \(x=\pm\sqrt{2}\)
24 tháng 6 2020
P(x) = 5x3 - 3x + 7 - x
= 5x3 - 4x + 7
Q(x) = -5x3 + 2x - 3 + 2x - x2 - 2
= -5x3 - x2 + 4x - 5
P(x) + Q(x) = ( 5x3 - 4x + 7 ) + ( -5x3 - x2 + 4x - 5 )
= 5x3 - 4x + 7 - 5x3 - x2 + 4x - 5
= -x2 + 2
P(x) - Q(x) = ( 5x3 - 4x + 7 ) - ( -5x3 - x2 + 4x - 5 )
= 5x3 - 4x + 7 + 5x3 + x2 - 4x + 5
= 10x3 + x2 - 8x + 12
Đặt H(x) = P(x) + Q(x)
=> H(x) = -x2 + 2
H(x) = 0 <=> -x2 + 2 = 0
<=> -x2 = -2
<=> x2 = 2
<=> x = \(\pm\sqrt{2}\)
Vậy nghiệm của đa thức là \(\pm\sqrt{2}\)
6 tháng 5 2021
a)
P(x) + O(x) = \(\left(x^3+2x^2-3x+2020\right)+\left(2x^3-3x^2+4x+2021\right)\)
P(x) + O(x) = \(3x^3-x^2+x+4041\)
b)
P(x) - O(x) = \(x^3+2x^2-3x+2020-2x^3+3x^2-4x-2021\)
P(x) - O(x) = \(-x^3+5x^2-7x-1\)
YN
27 tháng 4 2022
a) \(P\left(x\right)=x^2+4x+9-2x^3\)\(=-2x^3+x^2+4x+9\)
\(Q\left(x\right)=2x^3-3x+2x^2-9=2x^3+2x^2-3x-9\)
b) \(M\left(x\right)=P\left(x\right)+Q\left(x\right)=\left(-2x^3+x^2+4x+9\right)+\left(2x^3+2x^2-3x-9\right)\)
\(=\left(-2x^3+2x^3\right)+\left(x^2+2x^2\right)+\left(4x-3x\right)+\left(9-9\right)\)
\(=3x^2+x\)
c) Ta có: \(M\left(x\right)=3x^2+x\)
\(\Rightarrow M\left(-\dfrac{1}{3}\right)=3.\left(-\dfrac{1}{3}\right)^2+\left(-\dfrac{1}{3}\right)=\dfrac{1}{3}+\left(-\dfrac{1}{3}\right)=0\)
Vậy \(x=-\dfrac{1}{3}\) là nghiệm của đa thức \(M\left(x\right)\)
TC
9 tháng 3 2023
` P(x) = x^3-2x^2+x-2`
`Q(x) = 2x^3 - 4x^2+ 3x – 56`
a) `P(x) -Q(x)`
`= x^3-2x^2+x-2 - 2x^3 +4x^2 -3x +56`
`=(x^3-2x^3) +(4x^2-2x^2) +(x-3x) +(-2+56)`
`= -x^2 +2x^2 -2x +54`
b) Thay `x=2` vào `P(x)` ta đc
`P(2) = 2^3 -2*2^2 +2-2`
`= 8-8+2-2 =0`
Vậy chứng tỏ `x=2` là nghiệm của đa thức `P(x)`
Thay `x=2` vào `Q(x)` ta đc
`Q(2) = 2*2^3 -4*2^2 +3*2-56`
`=16 -16+6-56`
`= -50`
Vậy chứng tỏ `x=2` là ko nghiệm của đa thức `Q(x)`
19 tháng 12 2021
\(P\left(x\right)-Q\left(x\right)\)
\(=x^3+3x^3-x-4-x^4-3x^3+3x^5+2x-6\)
\(=3x^5+x-10\)
19 tháng 12 2021
\(P\left(x\right)-Q\left(x\right)=3x^2-5+x^4-x+1-6+2x-3x^3-x^4+3x^5\\ =3x^5-3x^3+3x^2+x-10\\ Q\left(x\right)-P\left(x\right)=6-2x+3x^3+x^4-3x^5-3x^2+5-x^4+x-1=-3x^5+3x^3-3x^2-x+10\)
Đó là 2 biểu thức đối nhau
Các hệ số của 2 đa thức đối nhau
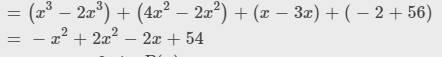
P(x)-Q(x)
\(=x^2-3x^2-2x^2-1-\left(x^2-x^2+x+1\right)\)
\(=-4x^2-1-\left(x+1\right)\)
\(=-4x^2-1-x-1\)
\(=-4x^2-x-2\)