Cho phân thức :
- tìm điều kiện của x để A có nghĩa.
- Rút gọn A.
Tính x để A < 1.
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
T
1
Những câu hỏi liên quan
NH
0
17 tháng 2 2021
a. \(ĐKXĐ:\left\{{}\begin{matrix}x\ne1\\x\ne-1\end{matrix}\right.\)
b. \(A=\dfrac{3x+3}{x^2-1}\\ A=\dfrac{3\left(x+1\right)}{\left(x-1\right)\left(x+1\right)}\\ A=\dfrac{3}{x-1}\)
c. Để \(A=-2\) thì \(\dfrac{3}{x-1}=-2=\dfrac{3}{\dfrac{-3}{2}}\\ \Leftrightarrow x-1=\dfrac{-3}{2}\\ \Leftrightarrow x=\dfrac{-1}{2}\left(\text{t/m ĐKXĐ}\right)\)
Vậy \(x=\dfrac{-1}{2}\) để phân thức nhận giá trị là -2.
TG
17 tháng 2 2021
a) Có: \(x^2-1=\left(x-1\right)\left(x+1\right)\)
ĐKXĐ là x ≠ 1; x ≠ -1
b) \(\dfrac{3x+3}{x^2-1}=\dfrac{3\left(x+1\right)}{\left(x-1\right)\left(x+1\right)}=\dfrac{3}{x-1}\)
c) Theo đề ta có: \(\dfrac{3}{x-1}=2\)
\(\Rightarrow x-1=\dfrac{3}{2}\)
\(\Rightarrow x=\dfrac{5}{2}\) (T/m ĐK)
VL
0
3 tháng 6 2018
1. Để A có nghĩa thì \(x^3-3x-2\ne0\)
\(\Rightarrow\left(x^3-x\right)-\left(2x-2\right)\ne0\)
\(\Rightarrow x\left(x^2-1\right)-2\left(x-1\right)\ne0\)
\(\Rightarrow x\left(x-1\right)\left(x+1\right)-2\left(x-1\right)\ne0\)
\(\Rightarrow\left(x^2+x-2\right)\left(x-1\right)\ne0\)
\(\Rightarrow\left(x^2-1+x-1\right)\left(x-1\right)\ne0\)
\(\Rightarrow\left[\left(x+1\right)\left(x-1\right)+\left(x-1\right)\right]\left(x-1\right)\ne0\)
\(\Rightarrow\left(x-1\right)^2\left(x+2\right)\ne0\)
\(\Rightarrow x\ne1;x\ne-2\)
2. \(A=\frac{x^4-2x^2+1}{x^3-3x-2}=\frac{\left(x^2-1\right)^2}{\left(x-1\right)^2\left(x+2\right)}=\frac{\left[\left(x-1\right)\left(x+1\right)\right]^2}{\left(x-1\right)^2\left(x+2\right)}\)
\(=\frac{\left(x-1\right)^2.\left(x+1\right)^2}{\left(x-1\right)^2\left(x+2\right)}=\frac{\left(x+1\right)^2}{x+2}\)
3/ Để A < 1 \(\Leftrightarrow\frac{\left(x+1\right)^2}{x+2}< 1\Leftrightarrow\left(x+1\right)^2< x+2\)
\(\Leftrightarrow x^2+2x+1< x+2\)
\(\Leftrightarrow x^2+x< 1\)
\(\Leftrightarrow x.\left(x+1\right)< 1\)
Vậy .....
3 tháng 6 2018
1. A có nghĩa khi \(x^3-3x-2\ne0\)
\(\Leftrightarrow x^3+x^2-x^2-x-2x-2\ne0\)
\(\Leftrightarrow x^2\left(x+1\right)-x\left(x+1\right)-2\left(x+1\right)\ne0\)
\(\Leftrightarrow\left(x+1\right)\left(x^2-x-2\right)\ne0\)
\(\Leftrightarrow\left(x+1\right)\left(x^2+x-2x-2\right)\)
\(\Leftrightarrow\left(x+1\right)\left(x+1\right)\left(x-2\right)\ne0\)
\(\Leftrightarrow\left(x+1\right)^2\left(x-2\right)\ne0\Leftrightarrow x-2\ne0\)(do \(\left(x+1\right)^2\ge0\)) \(\Leftrightarrow x\ne2\)
2. Ta có :
Tử = \(x^4-2x^2+1=x^4-x^3+x^3-x^2-x^2+x-x+1\)
=\(x^3\left(x-1\right)+x^2\left(x-1\right)-x\left(x-1\right)-\left(x-1\right)\)
=\(\left(x-1\right)\left(x^3+x^2-x-1\right)=\left(x-1\right)\left[x^2\left(x+1\right)-x\left(x+1\right)\right]\)
=\(\left(x-1\right)\left(x+1\right)\left(x^2-1\right)=\left(x-1\right)\left(x+1\right)\left(x-1\right)\left(x+1\right)\)
\(=\left(x+1\right)^2\left(x-1\right)^2\)
Vậy \(A=\frac{\left(x+1\right)^2\left(x-1\right)^2}{\left(x+1\right)^2\left(x-2\right)}=\frac{\left(x-1\right)^2}{x-2}\)
3. \(A< 1\Leftrightarrow\frac{\left(x-1\right)^2}{x-2}< 1\Leftrightarrow\frac{\left(x-1\right)^2}{x-2}-1< 0\Leftrightarrow\frac{x^2-2x+1-x+2}{x-2}< 0\)
\(\Leftrightarrow\frac{x^2-3x+3}{x-2}< 0\)ta có \(x^2-3x+3=x^2-2.\frac{3}{2}x+\frac{9}{4}+\frac{3}{4}=\left(x-\frac{3}{4}\right)^2+\frac{3}{4}>0\)
\(\Rightarrow\)(1) \(\Leftrightarrow x-2< 0\Leftrightarrow x< 2\)(Thỏa mãn)
Vậy x<2 thì A<1
AH
Akai Haruma
Giáo viên
30 tháng 10 2023
Lời giải:
a. ĐKXĐ: $x^2-16\neq 0\Leftrightarrow (x-4)(x+4)\neq 0$
$\Leftrightarrow x\neq \pm 4$
b. $A=\frac{x^2+8x+16}{x^2-16}=\frac{(x+4)^2}{(x-4)(x+4)}=\frac{x+4}{x-4}$
c. $A=3\Leftrightarrow \frac{x+4}{x-4}=3$
$\Rightarrow x+4=3(x-4)$
$\Leftrightarrow -2x+16=0$
$\Leftrightarrow x=8$ (tm)
d.
$A=0\Leftrightarrow \frac{x+4}{x-4}=0\Leftrightarrow x+4=0\Leftrightarrow x=-4$
Mà theo ĐKXĐ thì $x\neq \pm 4$ nên không tồn tại $x$ để $A=0$
13 tháng 1 2022
a: ĐKXĐ: \(x\notin\left\{0;1;-1\right\}\)
b: \(A=\dfrac{x\left(x+1\right)^2}{x\left(x+1\right)\left(x-1\right)}=\dfrac{x+1}{x-1}\)
c: Thay x=2 vào A, ta được:
\(A=\dfrac{2+1}{2-1}=3\)
d: Để A=2 thì x+1=2x-2
=>-x=-3
hay x=3(nhận)
PT
Cho biểu thức A: 2/√x-1. √x/√x-1. 2+3/x-1
a, tìm điều kiện của x để A có nghĩa rút gọn
b, tìm x để A
2
CM
25 tháng 12 2018
a) Phân thức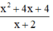 
xác định
⇔ x + 2 ≠ 0
⇔ x ≠ -2
Vậy điều kiện xác định của phân thức là x ≠ -2.
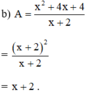
c) A = 1 ⇔ x + 2 = 1 ⇔ x = -1 ≠ -2 (Thỏa mãn điều kiện)
Vậy với x = -1 thì A = 1.
d) A = 0 ⇔ x + 2 = 0 ⇔ x = -2 (Không thỏa mãn điều kiện)
Vậy không có giá trị nào của x để A = 0.
M
29 tháng 12 2022
\(A=\dfrac{3}{x+3}+\dfrac{1}{x-3}+\dfrac{18}{x^2-9}\)
\(a,\) Điều kiện xác định: \(\left\{{}\begin{matrix}x+3\ne0\\x-3\ne0\\x^2-9\ne0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\ne-3\\x\ne3\end{matrix}\right.\)
\(b,A=\dfrac{3}{x+3}+\dfrac{1}{x-3}+\dfrac{18}{x^2-9}\)
\(=\dfrac{3\left(x-3\right)}{\left(x-3\right)\left(x+3\right)}+\dfrac{x+3}{\left(x-3\right)\left(x+3\right)}+\dfrac{18}{\left(x-3\right)\left(x+3\right)}\)
\(=\dfrac{3x-9+x+3+18}{\left(x-3\right)\left(x+3\right)}\)
\(=\dfrac{4x+12}{\left(x-3\right)\left(x+3\right)}\)
\(=\dfrac{4\left(x+3\right)}{\left(x-3\right)\left(x+3\right)}\)
\(=\dfrac{4}{x-3}\)
\(c,x=1\Rightarrow A=\dfrac{4}{1-3}=-2\)
1.A=\(\frac{x^4-2x^2+1}{x^3-3x-2}\)
A có nghĩa \(\Leftrightarrow x^3-3x-2\ne0\Leftrightarrow\left(x+1\right)^2\left(x-2\right)\ne0\Leftrightarrow\hept{\begin{cases}x\ne-1\\x\ne2\end{cases}}\)
2 .A = \(\frac{x^4-2x^2+1}{x^3-3x-2}\)=\(\frac{\left(x^2-1\right)^2}{\left(x+1\right)^2\left(x-2\right)}=\frac{\left(x+1\right)^2\left(x-1\right)^2}{\left(x+1\right)^2\left(x-2\right)}=\frac{\left(x-1\right)^2}{x-2}\)
A<1\(\Rightarrow\frac{\left(x-1\right)^2}{x-2}-1< 0\Rightarrow\frac{x^2-2x+1-x+2}{x-2}< 0\)
\(\Rightarrow\frac{x^2-3x+3}{x-2}< 0\Rightarrow x-2< 0\)vì \(x^2-3x+3=\left(x-\frac{3}{2}\right)^2+\frac{3}{4}\ge\frac{3}{4}\)
Vậy x<2 thỏa mãn yêu cầu A<1