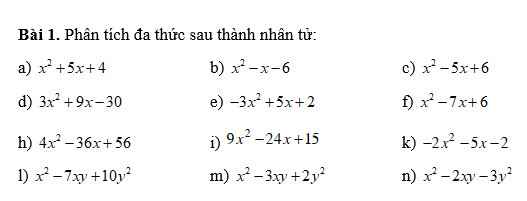ANH CHỊ ƠI GIÚP EM BÀI TẬP ĐỊA LÍ LỚP10 VỚI Ạ , NGÀY MAI E CÓ KIỂM TRA 15P, nhma cô giảng em chưa hiểu anh chị giúp e với , em cảm ơn ạ
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
26 tháng 10 2021
câu 5:
x=3,6
y=6,4
câu 6: chụp lại đề
câu 7:
a)ĐKXĐ: \(x\ge0\)
\(3\sqrt{x}=\sqrt{12}\\ \Rightarrow9x=12\\ \Rightarrow x=\dfrac{4}{3}\)
b) ĐKXĐ: \(x\ge6\)
\(\sqrt{x-6}=3\\ \Rightarrow x-6=9\\ \Rightarrow x=15\)
16 tháng 11 2021
Bài 2:
\(\dfrac{1}{2}:\dfrac{5}{4}=x:\dfrac{10}{3}\Leftrightarrow\dfrac{1}{2}.\dfrac{4}{5}=\dfrac{3}{10}x\Leftrightarrow\dfrac{3}{10}x=\dfrac{2}{5}\Leftrightarrow x=\dfrac{2}{5}:\dfrac{3}{10}=\dfrac{4}{3}\)
Bài 3:
Áp dụng t/c dtsbn:
\(\dfrac{x}{4}=\dfrac{y}{12}=\dfrac{x+y}{4+12}=\dfrac{48}{16}=3\)
\(\Rightarrow\left\{{}\begin{matrix}x=3.4=12\\y=3.12=36\end{matrix}\right.\)
28 tháng 10 2021
\(a,P=\dfrac{3\sqrt{a}-3}{\sqrt{a}\left(\sqrt{a}+1\right)}\cdot\dfrac{\left(\sqrt{a}+1\right)^2}{\sqrt{a}-1}\left(a\ge0;a\ne1\right)\\ P=\dfrac{3\left(\sqrt{a}-1\right)\left(\sqrt{a}+1\right)}{\sqrt{a}\left(\sqrt{a}-1\right)}=\dfrac{3\left(\sqrt{a}+1\right)}{\sqrt{a}}\\ b,a=4\Leftrightarrow\sqrt{a}=2\\ \Leftrightarrow P=\dfrac{3\left(2+1\right)}{2}=\dfrac{9}{2}\)
TS
0
15 tháng 11 2021
\(a,=x^2+x+4x+4=\left(x+1\right)\left(x+4\right)\\ b,=x^2+2x-3x-6=\left(x-3\right)\left(x+2\right)\\ c,=x^2-2x-3x+6=\left(x-2\right)\left(x-3\right)\\ d,=3\left(x^2-2x+5x-10\right)=3\left(x-2\right)\left(x+5\right)\\ e,=-3x^2+6x-x+2=\left(x-2\right)\left(1-3x\right)\\ f,=x^2-x-6x+6=\left(x-1\right)\left(x-6\right)\\ h,=4\left(x^2-3x-6x+18\right)=4\left(x-3\right)\left(x-6\right)\\ i,=3\left(3x^2-3x-8x+5\right)=3\left(x-1\right)\left(3x-8\right)\\ k,=-\left(2x^2+x+4x+2\right)=-\left(2x+1\right)\left(x+2\right)\\ l,=x^2-2xy-5xy+10y^2=\left(x-2y\right)\left(x-5y\right)\\ m,=x^2-xy-2xy+2y^2=\left(x-y\right)\left(x-2y\right)\\ n,=x^2+xy-3xy-3y^2=\left(x+y\right)\left(x-3y\right)\)
TN
1
JP
1
TN
2
27 tháng 10 2021
a: \(P=\left(\dfrac{2}{\sqrt{x}-1}+\dfrac{\sqrt{x}}{\sqrt{x}+1}\right)\cdot\dfrac{\sqrt{x}}{x+\sqrt{x}+2}\)
\(=\dfrac{2\sqrt{x}+2+x-\sqrt{x}}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\cdot\dfrac{\sqrt{x}}{x+\sqrt{x}+2}\)
\(=\dfrac{\sqrt{x}}{x-1}\)
27 tháng 10 2021
\(P=\left(\dfrac{2}{\sqrt{x}-1}+\dfrac{\sqrt{x}}{\sqrt{x}+1}\right).\dfrac{\sqrt{x}}{x+\sqrt{x}+2}\)
\(\Rightarrow P=\dfrac{2\left(\sqrt{x}+1\right)+\sqrt{x}\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}.\dfrac{\sqrt{x}}{x+\sqrt{x}+2}\)
\(\Rightarrow P=\dfrac{x+\sqrt{x}+2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}.\dfrac{\sqrt{x}}{x+\sqrt{x}+2}\)
\(\Rightarrow P=\dfrac{\sqrt{x}}{x-1}\)
\(\Rightarrow P=\dfrac{\sqrt{3+2\sqrt{2}}}{3+2\sqrt{2}-1}\)
\(\Rightarrow P=\dfrac{\sqrt{\left(\sqrt{2}+1\right)^2}}{2+2\sqrt{2}}\)
\(\Rightarrow P=\dfrac{\sqrt{2}+1}{2\left(\sqrt{2}+1\right)}\)
\(\Rightarrow P=\dfrac{1}{2}\)
TN
2
SC
11 tháng 1 2022
Câu 20: Giao điểm của đồ thị và trục tung là điểm có toạ độ (0;-3)
Thay ngược vào phương trình => b = -3. Vậy chọn D