thu gọn biểu thức A=3x(4x-3)-(x+1) x^2-(11x^2-12)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
CM
12 tháng 7 2017
x + 2 x - 3 = x - x + 3 x - 3 = x ( x - 1) + 3( x - 1) = ( x - 1)( x + 3)
a) Với điểu kiện x ≥ 0; x ≠ 1 ta có:
NM
1
5 tháng 12 2015
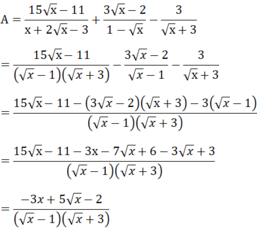
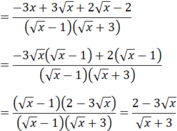
\(y=\frac{\frac{^x}{x^2}-x-6-x-\frac{1}{3}x^2-4x-15}{x^4}-2x^2+\frac{1}{3}x^2+11x+10b\)
\(y=\frac{-\left(5x^7-33x^6-30bx^5+x^3+18x^2+63x-3\right)}{3x^5}\)
H9
HT.Phong (9A5)
CTVHS
7 tháng 10 2023
a) \(C=\left(\dfrac{x}{x^2-x-6}-\dfrac{x-1}{3x^2-4x-15}\right):\dfrac{x^4-2x^2+1}{3x^2+11x+10}\cdot\left(x^2-2x+1\right)\) (ĐK: \(x\ne-\dfrac{5}{3};x\ne3;x\ne-2;x\ne1\))
\(C=\left[\dfrac{x}{\left(x-3\right)\left(x+2\right)}-\dfrac{x-1}{\left(x-3\right)\left(3x+5\right)}\right]:\dfrac{\left(x^2-1\right)^2}{\left(3x+5\right)\left(x+2\right)}\cdot\left(x-1\right)^2\)
\(C=\left[\dfrac{x\left(3x+5\right)}{\left(3x+5\right)\left(x+2\right)\left(x-3\right)}-\dfrac{\left(x-1\right)\left(x+2\right)}{\left(x-3\right)\left(3x+5\right)\left(x+2\right)}\right]\cdot\dfrac{\left(3x+5\right)\left(x+2\right)}{\left(x^2-1\right)^2\left(x-1\right)^2}\)
\(C=\dfrac{3x^2+5x-x^2-2x+x+2}{\left(3x+5\right)\left(x+2\right)\left(x-3\right)}\cdot\dfrac{\left(3x+5\right)\left(x+2\right)}{\left(x^2-1\right)^2\left(x-1\right)^2}\)
\(C=\dfrac{2x^2+4x+2}{\left(3x+5\right)\left(x+2\right)\left(x-3\right)}\cdot\dfrac{\left(3x+5\right)\left(x+2\right)}{\left(x+1\right)^2\left(x-1\right)^4}\)
\(C=\dfrac{2\left(x+1\right)^2}{\left(3x+5\right)\left(x-3\right)\left(x+2\right)}\cdot\dfrac{\left(3x+5\right)\left(x+2\right)}{\left(x+1\right)^2\left(x-1\right)^4}\)
\(C=\dfrac{2}{\left(x-1\right)^4\left(x-3\right)}\)
b) Thay x = 2003 ta có:
\(C=\dfrac{2}{\left(2003-1\right)^4\left(2003-3\right)}=\dfrac{2}{2002^4\cdot2000}=\dfrac{1}{2002^4\cdot1000}\)
c) \(C>0\) khi:
\(\dfrac{2}{\left(x-1\right)^4\left(x-3\right)}>0\) mà: \(\left\{{}\begin{matrix}2>0\\\left(x-1\right)^4>0\end{matrix}\right.\)
\(\Leftrightarrow x-3>0\)
\(\Leftrightarrow x>3\) (đpcm)
NM
0
LM
0
17 tháng 3 2021
TXĐ: \(\left\{{}\begin{matrix}x\in R\\x\notin\left\{0;-1\right\}\end{matrix}\right.\)
30 tháng 7 2019
a) 3x(x + 2) + 4x(-2x + 3) + (2x - 3)(3x + 1)
= 3x2 + 6x - 8x2 + 12x + 6x2 + 2x - 9x - 3
= (3x2 - 8x2 + 6x2) + (6x + 12x + 2x - 9x) - 3
= x3 + 11x - 3
b) (x2 + 1)(x2 - x + 2) - (x2 - 1)(x2 + x - 2)
= x4 - x3 + 3x2 - x + 2 - x4 - x3 + 3x2 + x - 2
= (x4 - x4) + (-x3 - x3) + (3x2 + 3x2) + (-x + x) + (2 - 2)
= -2x3 + 6x2
c) (-2x - 3)2 + (3x + 2)2 + (4x + 1)
= 4x2 + 12x + 9 + 9x2 + 12x + 4 + 4x + 1
= (4x2 + 9x2) + (12x + 12x + 4x) + (9 + 4 + 1)
= 13x2 + 28x + 14
CW
27 tháng 6 2016
a) \(\left(2x-1\right)^2-\left(x+2\right)^2-3x^2+5x\)
\(=4x^2-4x+1-\left(x^2+4x+4\right)-3x^2+5x\)
\(=x^2-3x-3\)
b) \(\left(x+2\right)\left(x-1\right)+2\left(3x-2\right)^2+4x-19x^2\)
\(=x^2+2x-x-2+2\left(9x^2-12x+4\right)+4x-19x^2\)
\(=x^2+2x-x-2+18x^2-24x+8+4x-19x^2\)
\(=-19x+6\)
c) \(2\left(3-x\right)\left(x-2\right)-\left(3x+1\right)^2+5x-11x^2\)
\(=6-2x\left(x-2\right)-\left(9x^2+6x+1\right)+5x-11x^2\)
\(=6-2x^3+4x-9x^2-6x-1+5x-11x^2\)
\(=-2x^3-20x^2+3x+5\)
29 tháng 7 2020
Ta có: \(A=\left(4x-2\right)^2+\left(-3x+1\right)^2-\left(4x-1\right)\left(3-3x\right)\)
\(=16x^2-16x+4+9x^2-6x+1-\left(12x-12x^2-3+3x\right)\)
\(=25x^2-22x+5-15x+12x^2+3\)
\(=37x^2-37x+8\)
Thay x=-3 vào biểu thức \(A=37x^2-37x+8\), ta được:
\(A=37\cdot\left(-3\right)^2-37\cdot\left(-3\right)+8\)
\(=37\cdot9+111+8\)
\(=333+111+8\)
\(=452\)
Vậy: 452 là giá trị của biểu thức \(A=\left(4x-2\right)^2+\left(-3x+1\right)^2-\left(4x-1\right)\left(3-3x\right)\) tại x=-3
A= 12x^2-9x-x^3-x^2-11x^2+12
A=-x^3-9x+12