Câu 1 : tính đạo hàng của hàm số sau : a, y = 1/| cosx |
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
CM
2 tháng 4 2019
Đáp án A
Ta có y ' = cos x sin x − cos x − cos x + sin x sin x sin x − cos x 2 = − cos 2 x − sin 2 x sin x − cos x 2 = − 1 sin x − cos x 2
CM
5 tháng 1 2020
y ' = sin x + cos x sin x − cos x ' = ( sin x + cos x ) ' . ( sin x − cos x ) − ( sin x − cos x ) ' . ( sin x + cos x ) ( sin x − cos x ) 2 = ( cos x − sin x ) ( sin x − cos x ) − ( cos x + sin x ) ( sin x + cos x ) ( sin x − cos x ) 2 = − ( cos x − sin x ) ( − sin x + cos x ) − ( sin x + cos x ) ( sin x + cos x ) ( sin x − cos x ) 2
= − ( cos x − sin x ) 2 − ( sin x + cos x ) 2 ( sin x − cos x ) 2 = − ( cos 2 x − 2 cos x sin x + sin 2 x ) − ( sin 2 x + 2 sin x cos x + cos 2 x ) ( sin x − cos x ) 2 = − ( 1 − 2 cos x sin x ) − ( 1 + 2 sin x cos x ) ( sin x − cos x ) 2
= − 2 ( sin x − cos x ) 2
Chọn đáp án C
NV
Nguyễn Việt Lâm
Giáo viên
3 tháng 5 2021
1.
\(\lim\limits_{x\rightarrow-1}\dfrac{2x^2-x-3}{x^2-1}=\lim\limits_{x\rightarrow-1}\dfrac{\left(x+1\right)\left(2x-3\right)}{\left(x+1\right)\left(x-1\right)}=\lim\limits_{x\rightarrow-1}\dfrac{2x-3}{x-1}=\dfrac{5}{2}\)
2.
a. \(y'=6x^2-sinx-\dfrac{1}{2\sqrt{x}}\)
b. \(y'=10\left(x^2-5\right)^9.\left(x^2-5\right)'=20x\left(x^2-5\right)^9\)
3.
\(y'=-2x\)
\(k=4\Rightarrow-2x=4\Rightarrow x=-2\Rightarrow y\left(-2\right)=-24\)
Phương trình tiếp tuyến:
\(y=4\left(x+2\right)-24\Leftrightarrow y=4x-16\)
PT
1
PT
1
24 tháng 2 2022
mình tìm thấy nguồn này nhưng cách làm này không đúng nhé nên mình mới đăng câu hỏi để hỏi m.n
NV
Nguyễn Việt Lâm
Giáo viên
3 tháng 5 2021
a.
\(y'=\dfrac{\left(sinx+cosx\right)'}{2\sqrt{sinx+cosx}}=\dfrac{cosx-sinx}{2\sqrt{sinx+cosx}}\)
b.
\(y'=\dfrac{-4}{\left(x-1\right)^2}\)
Tiếp tuyến vuông góc với \(y=\dfrac{1}{4}x+5\) nên có hệ số góc thỏa mãn \(k.\left(\dfrac{1}{4}\right)=-1\Rightarrow k=-4\)
\(\Rightarrow\dfrac{-4}{\left(x-1\right)^2}=-4\Rightarrow\left(x-1\right)^2=1\)
\(\Rightarrow\left[{}\begin{matrix}x=0\Rightarrow y=-3\\x=2\Rightarrow y=5\end{matrix}\right.\)
Có 2 tiếp tuyến thỏa mãn: \(\left[{}\begin{matrix}y=-4x-3\\y=-4\left(x-2\right)+5\end{matrix}\right.\)
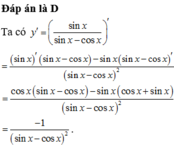
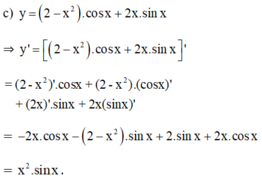
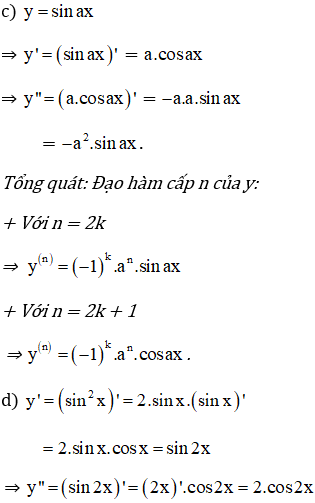
Lời giải:
\(y=\frac{1}{|\cos x|}\Rightarrow y'=\frac{\sin x\cos x}{|\cos x|^3}\)