Giúp em với ạ. Cần gấp 😥😥😥😥🙏🙏🙏🙏
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
VT
2
NV
Nguyễn Việt Lâm
Giáo viên
27 tháng 3 2022
23.
Gọi I là trung điểm MN \(\Rightarrow I\left(3;3\right)\)
\(\Rightarrow\overrightarrow{IN}=\left(2;-1\right)\Rightarrow IN=\sqrt{5}\)
Phương trình đường tròn đường kính MN, nhận I là tâm và có bán kính \(R=IN\) là:
\(\left(x-3\right)^2+\left(y-3\right)^2=5\)
Thay tọa độ E vào pt ta được:
\(\left(x-3\right)^2+4=5\Rightarrow\left(x-3\right)^2=1\)
\(\Rightarrow\left[{}\begin{matrix}x=4\\x=2\end{matrix}\right.\) \(\Rightarrow x_1x_2=8\)
Cả 4 đáp án của câu này đều sai
NV
Nguyễn Việt Lâm
Giáo viên
27 tháng 3 2022
24.
Gọi \(M\left(x;y\right)\) là 1 điểm bất kì thuộc \(\Delta\)
Do \(\Delta\) là đường phân giác của góc tạo bởi d và k nên:
\(d\left(M;d\right)=d\left(M;k\right)\Leftrightarrow\dfrac{\left|2x+y\right|}{\sqrt{2^2+1^2}}=\dfrac{\left|x+2y-3\right|}{\sqrt{1^2+2^2}}\)
\(\Leftrightarrow\left|2x+y\right|=\left|x+2y-3\right|\)
\(\Leftrightarrow\left[{}\begin{matrix}2x+y=x+2y-3\\2x+y=-x-2y+3\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x-y+3=0\\x+y-1=0\end{matrix}\right.\)
- Với \(x-y+3=0\), ta có:
\(\left(x_E-y_E+3\right)\left(x_F-y_F+3\right)=2.1=2>0\Rightarrow E;F\) nằm cùng phía so với \(x-y+3=0\) (thỏa mãn)
- Với \(x+y-1=0\) ta có:
\(\left(x_E+y_E-1\right)\left(x_F+y_F-1\right)=2.7=14>0\Rightarrow E;F\) nằm cùng phía so với \(x+y-1=0\) (thỏa mãn)
Vậy cả đáp án A và D đều đúng
Tương tự như câu 23, câu 24 đề bài tiếp tục sai
4 tháng 12 2019
Bạn ơi , bạn xem lại đề nhé! Mình làm thế này không biết có đúng đề không nữa?
Ta có \(a^2+c^2\ge0\) (gt) mà \(a^2\ge0 \forall a, c^2\ge0 \forall c\)=> \(a\ne0 , c\ne0\)=> \(b\ne0\)( vì \(ab=c^2\))
Với \(a,b,c \ne0\), \(ab=c^2\)=> \(\frac{a}{c}=\frac{c}{b}\)
=> \(\left(\frac{a}{c}\right)^2=\left(\frac{c}{b}\right)^2\)
=> \(\frac{a^2}{c^2}=\frac{c^2}{b^2}=\frac{a^2+c^2}{c^2+b^2}\) mà \(\frac{a}{c}=\frac{c}{b}\)
=> \(\frac{a^2+c^2}{c^2+b^2}=\frac{a}{c}.\frac{c}{b}=\frac{a}{b}\)
O
4 tháng 12 2017
Hello everybody, I'm Thao and I'm an A.R.M.Y . I am living in a happy family. There are four people in my family. Their names are : Jimin, Taehyung , Jungkook and me. They are handsome and cute singers. First, Jimin. His full name is Park Jimin. He was born on 13/10/1995 in Busan. Jimin is a cute boy with a round face and full lips. Selfie is his hobby. His lucky number is number three. Second, Kim Taehyung. Taehyung was born on 30/12/1995 in Deagu, Korea. His favorite colors are black, white and green. He's outgoing and he's so lovely . Joen Jungkook, was born on 1/9/1997 in Busan. "Fat Rabbit" is his nick name. Kook is very cute, too. He love dancing and skateboarding. I love my family so much ! Thanks for your listening !
NN
1
S
10 tháng 2 2023
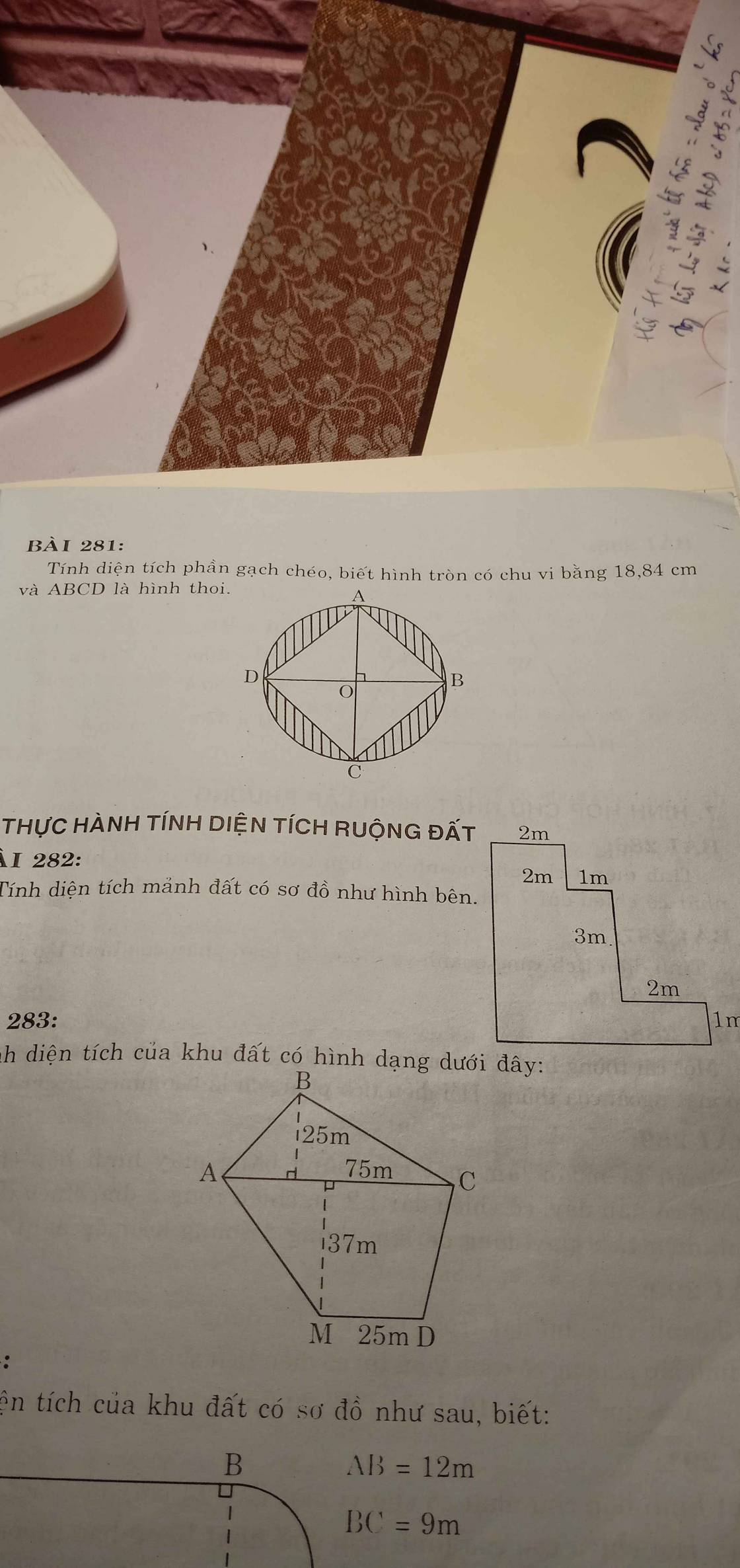
Bán kính hình tròn:
\(18,84:3,14:2=3\left(cm\right)\)
Diện tích hình tròn:
\(3\times3\times3,14=28,26\left(cm^2\right)\)
Đường kính hình tròn:
\(3\times2=6\left(cm\right)\)
Diện tích hình thoi:
\(\dfrac{6\times6}{2}=18\left(cm^2\right)\)
Diện tích phần gạch chéo:
\(28,26-18=10,26\left(cm^2\right)\)
NV
Nguyễn Việt Lâm
Giáo viên
10 tháng 4 2022
4b.
\(\dfrac{\pi}{2}< a< \pi\Rightarrow cosa< 0\Rightarrow cosa=-\sqrt{1-sin^2a}=-\dfrac{4}{5}\)
\(\Rightarrow tana=\dfrac{sina}{cosa}=-\dfrac{3}{4}\)
\(tan\left(a+\dfrac{\pi}{3}\right)=\dfrac{tana+tan\left(\dfrac{\pi}{3}\right)}{1-tana.tan\left(\dfrac{\pi}{3}\right)}=\dfrac{-\dfrac{3}{4}+\sqrt{3}}{1-\left(-\dfrac{3}{4}\right).\sqrt{3}}=...\)
c.
\(\dfrac{3\pi}{2}< a< 2\pi\Rightarrow cosa>0\Rightarrow cosa=\sqrt{1-sin^2a}=\dfrac{5}{13}\)
\(cos\left(\dfrac{\pi}{3}-a\right)=cos\left(\dfrac{\pi}{3}\right).cosa+sin\left(\dfrac{\pi}{3}\right).sina=\dfrac{1}{2}.\dfrac{5}{13}+\left(-\dfrac{12}{13}\right).\dfrac{\sqrt{3}}{2}=...\)
16 tháng 6 2023
5:
a: sin x=2*cosx
\(A=\dfrac{6cosx+2cosx-4\cdot8\cdot cos^3x}{cos^3x-2cosx}\)
\(=\dfrac{8-32cos^2x}{cos^2x-2}\)
b: VT=sin^4(pi/2-x)+cos^4(x+pi/2)+6*1/2*sin^22x+1/2*cos4x
=cos^4x+sin^4x+3*sin^2(2x)+1/2*(1-2*sin^2(2x))
=1-2*sin^2x*cos^2x+3*sin^2(2x)+1/2-sin^2(2x)
==3/2=VP
2 tháng 7 2023
4:
a: -90<a<0
=>cos a>0
cos^2a=1-(-4/5)^2=9/25
=>cosa=3/5
\(sin\left(45-a\right)=sin45\cdot cosa-cos45\cdot sina=\dfrac{\sqrt{2}}{2}\left(cosa-sina\right)\)
\(=\dfrac{\sqrt{2}}{2}\left(\dfrac{3}{5}-\dfrac{4}{5}\right)=\dfrac{-\sqrt{2}}{10}\)
b: pi/2<a<pi
=>cosa<0
cos^2a+sin^2a=0
=>cos^2a=16/25
=>cosa=-4/5
tan a=3/5:(-4/5)=-3/4
\(tan\left(a+\dfrac{pi}{3}\right)=\dfrac{tana+\dfrac{tanpi}{3}}{1-tana\cdot tan\left(\dfrac{pi}{3}\right)}\)
\(=\dfrac{-\dfrac{3}{4}+\sqrt{3}}{1-\dfrac{-3}{4}\cdot\sqrt{3}}=\dfrac{48-25\sqrt{3}}{11}\)
c: 3/2pi<a<pi
=>cosa>0
cos^2a+sin^2a=1
=>cos^2a=25/169
=>cosa=5/13
cos(pi/3-a)
\(=cos\left(\dfrac{pi}{3}\right)\cdot cosa+sin\left(\dfrac{pi}{3}\right)\cdot sina\)
\(=\dfrac{5}{13}\cdot\dfrac{1}{2}+\dfrac{-12}{13}\cdot\dfrac{\sqrt{3}}{2}=\dfrac{5-12\sqrt{3}}{26}\)
TL
1