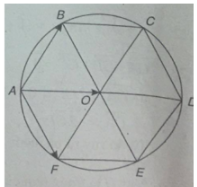
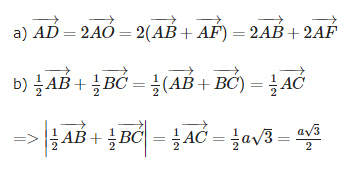Cho lục giác đều $A B C D E F$ tâm $O$. Chứng minh: $\overrightarrow{O A}+\overrightarrow{O B}+\overrightarrow{O C}+\overrightarrow{O D}+\overrightarrow{O E}+\overrightarrow{O F}=\overrightarrow{0}$.
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
L
✎﹏l๏gคภlєє︵²ᵏ¹⁰
25 tháng 3 2022
Đúng(0)
S
SANS:))$$^
25 tháng 3 2022
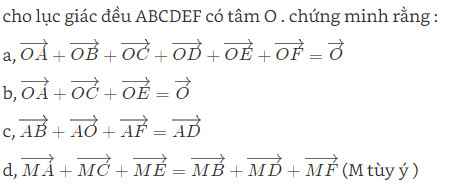
đúng ko v ???????
Đúng(0)
Những câu hỏi liên quan
23 tháng 9 2017
(*) mk mới hok dạng toán này trên mạng ; nên lm thử thôi nha bn
hình :
a) ta có : \(VT=\overrightarrow{OA}+\overrightarrow{OB}+\overrightarrow{OC}+\overrightarrow{OD}+\overrightarrow{OE}+\overrightarrow{OF}\)
\(=\overrightarrow{OA}+\overrightarrow{EO}+\overrightarrow{OC}+\overrightarrow{AO}+\overrightarrow{OE}+\overrightarrow{CO}\)
\(=\left(\overrightarrow{AO}+\overrightarrow{OA}\right)+\left(\overrightarrow{CO}+\overrightarrow{OC}\right)+\left(\overrightarrow{EO}+\overrightarrow{OE}\right)\)
\(=\overrightarrow{AA}+\widehat{CC}+\overrightarrow{EE}=\overrightarrow{0}+\overrightarrow{0}+\overrightarrow{0}=\overrightarrow{0}=VP\left(đpcm\right)\)
b) ta có : \(VT=\overrightarrow{OA}+\overrightarrow{OC}+\overrightarrow{OE}=\overrightarrow{FO}+\overrightarrow{OE}-\overrightarrow{AO}\)
\(=\overrightarrow{FE}-\overrightarrow{FE}=\overrightarrow{EE}=\overrightarrow{0}=VP\left(đpcm\right)\)
c) ta có : \(VT=\overrightarrow{AB}+\overrightarrow{AO}+\overrightarrow{AF}=\overrightarrow{AB}+\overrightarrow{AF}+\overrightarrow{FE}\)
\(=\overrightarrow{AB}+\overrightarrow{AE}=\overrightarrow{AB}+\overrightarrow{BD}=\overrightarrow{AD}=VP\left(đpcm\right)\)
d) ta có : \(VT=\overrightarrow{MA}+\overrightarrow{MC}+\overrightarrow{ME}=\overrightarrow{MB}+\overrightarrow{BA}+\overrightarrow{MD}+\overrightarrow{DC}+\overrightarrow{MF}+\overrightarrow{FE}\)
\(=\left(\overrightarrow{MB}+\overrightarrow{MD}+\overrightarrow{MF}\right)+\left(\overrightarrow{BA}+\overrightarrow{DC}+\overrightarrow{FE}\right)\)
\(=\left(\overrightarrow{MB}+\overrightarrow{MD}+\overrightarrow{MF}\right)+\left(\overrightarrow{BA}+\overrightarrow{FE}+\overrightarrow{EO}\right)\) \(=\left(\overrightarrow{MB}+\overrightarrow{MD}+\overrightarrow{MF}\right)+\left(\overrightarrow{BA}+\overrightarrow{FO}\right)\) \(=\left(\overrightarrow{MB}+\overrightarrow{MD}+\overrightarrow{MF}\right)+\left(\overrightarrow{BA}-\overrightarrow{OF}\right)\) \(=\left(\overrightarrow{MB}+\overrightarrow{MD}+\overrightarrow{MF}\right)+\left(\overrightarrow{BA}-\overrightarrow{BA}\right)\) \(=\left(\overrightarrow{MB}+\overrightarrow{MD}+\overrightarrow{MF}\right)+\overrightarrow{AA}=\left(\overrightarrow{MB}+\overrightarrow{MD}+\overrightarrow{MF}\right)+\overrightarrow{0}\) \(=\overrightarrow{MB}+\overrightarrow{MD}+\overrightarrow{MF}=VP\left(đpcm\right)\)
HQ
Hà Quang Minh
Giáo viên
25 tháng 9 2023
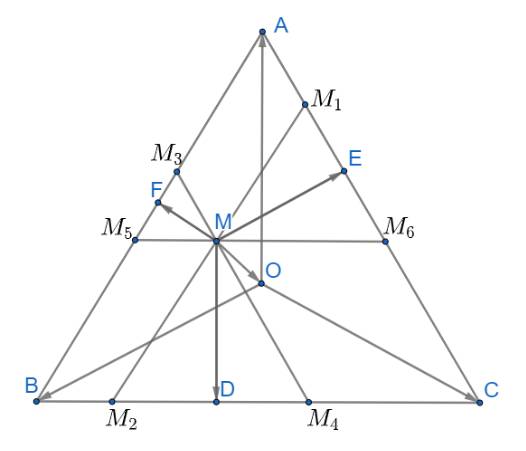
\(\overrightarrow {MD} + \overrightarrow {ME} + \overrightarrow {MF} = \left( {\overrightarrow {MO} + \overrightarrow {OD} } \right) + \left( {\overrightarrow {MO} + \overrightarrow {OE} } \right) + \left( {\overrightarrow {MO} + \overrightarrow {OF} } \right)\)
Qua M kẻ các đường thẳng \({M_1}{M_2}//AB;{M_3}{M_4}//AC;{M_5}{M_6}//BC\)
Từ đó ta có: \(\widehat {M{M_1}{M_6}} = \widehat {M{M_6}{M_1}} = \widehat {M{M_4}{M_2}} = \widehat {M{M_2}{M_4}} = \widehat {M{M_3}{M_5}} = \widehat {M{M_5}{M_3}} = 60^\circ \)
Suy ra các tam giác \(\Delta M{M_3}{M_5},\Delta M{M_1}{M_6},\Delta M{M_2}{M_4}\) đều
Áp dụng tính chất trung tuyến \(\overrightarrow {AM} = \frac{1}{2}\left( {\overrightarrow {AB} + \overrightarrow {AC} } \right)\)(với M là trung điểm của BC) ta có:
\(\overrightarrow {ME} = \frac{1}{2}\left( {\overrightarrow {M{M_1}} + \overrightarrow {M{M_6}} } \right);\overrightarrow {MD} = \frac{1}{2}\left( {\overrightarrow {M{M_2}} + \overrightarrow {M{M_4}} } \right);\overrightarrow {MF} = \frac{1}{2}\left( {\overrightarrow {M{M_3}} + \overrightarrow {M{M_5}} } \right)\)
\( \Rightarrow \overrightarrow {MD} + \overrightarrow {ME} + \overrightarrow {MF} = \frac{1}{2}\left( {\overrightarrow {M{M_2}} + \overrightarrow {M{M_4}} } \right) + \frac{1}{2}\left( {\overrightarrow {M{M_1}} + \overrightarrow {M{M_6}} } \right) + \frac{1}{2}\left( {\overrightarrow {M{M_3}} + \overrightarrow {M{M_5}} } \right)\)
Ta có: các tứ giác \(A{M_3}M{M_1};C{M_4}M{M_6};B{M_2}M{M_5}\) là hình bình hành
Áp dụng quy tắc hình bình hành ta có
\(\overrightarrow {MD} + \overrightarrow {ME} + \overrightarrow {MF} = \frac{1}{2}\left( {\overrightarrow {M{M_2}} + \overrightarrow {M{M_4}} } \right) + \frac{1}{2}\left( {\overrightarrow {M{M_1}} + \overrightarrow {M{M_6}} } \right) + \frac{1}{2}\left( {\overrightarrow {M{M_3}} + \overrightarrow {M{M_5}} } \right)\)
\( = \frac{1}{2}\left( {\overrightarrow {M{M_1}} + \overrightarrow {M{M_3}} } \right) + \frac{1}{2}\left( {\overrightarrow {M{M_2}} + \overrightarrow {M{M_5}} } \right) + \frac{1}{2}\left( {\overrightarrow {M{M_4}} + \overrightarrow {M{M_6}} } \right)\)
\( = \frac{1}{2}\overrightarrow {MA} + \frac{1}{2}\overrightarrow {MB} + \frac{1}{2}\overrightarrow {MC} = \frac{1}{2}\left( {\overrightarrow {MA} + \overrightarrow {MB} + \overrightarrow {MC} } \right)\)
\( = \frac{1}{2}\left( {\left( {\overrightarrow {MO} + \overrightarrow {OA} } \right) + \left( {\overrightarrow {MO} + \overrightarrow {OB} } \right) + \left( {\overrightarrow {MO} + \overrightarrow {OC} } \right)} \right)\)
\( = \frac{1}{2}\left( {3\overrightarrow {MO} + \left( {\overrightarrow {MA} + \overrightarrow {MB} + \overrightarrow {MC} } \right)} \right) = \frac{3}{2}\overrightarrow {MO} \) (đpcm)
Vậy \(\overrightarrow {MD} + \overrightarrow {ME} + \overrightarrow {MF} = \frac{3}{2}\overrightarrow {MO} \)
28 tháng 3 2022
Giải thích các bước giải:
a) Kẻ đường kính BF.
Ta có: AH⊥BC,CF⊥BC⇒AH//CFAH⊥BC,CF⊥BC⇒AH//CF
Lại có AF⊥AB,CH⊥AB⇒AF//CHAF⊥AB,CH⊥AB⇒AF//CH
⇒AHCF⇒AHCF là hình bình hành.
⇒−−→AH=−−→FC⇒AH→=FC→.
Lại có OIOI là đường trung bình của tam giác BCF nên −→OI=12−−→FCOI→=12FC→
Vậy −−→AH=−−→FC=2−→OIAH→=FC→=2OI→.
b) Ta có: −−→OH=−−→OA+−−→AH=−−→OA+2−→OI=−−→OA+−−→OB+−−→OCOH→=OA→+AH→=OA→+2OI→=OA→+OB→+OC→
c) Do GG là trọng tâm tam giác ABC nên−−→OA+−−→OB+−−→OC=3−−→OG⇒−−→OG=13(−−→OA+−−→OB+−−→OC)=13−−→OHOA→+OB→+OC→=3OG→⇒OG→=13(OA→+OB→+OC→)=13OH→
Vậy ba điểm O,H,GO,H,G thẳng hàng.
NV
Nguyễn Việt Lâm
Giáo viên
24 tháng 8 2021
\(\overrightarrow{AB}+\overrightarrow{CD}=\overrightarrow{AB}+\overrightarrow{CB}+\overrightarrow{BD}=\overrightarrow{AB}+\overrightarrow{BD}+\overrightarrow{CB}=\overrightarrow{AD}+\overrightarrow{CB}\)
\(\overrightarrow{OA}+\overrightarrow{OB}+\overrightarrow{OC}+\overrightarrow{OD}=\left(\overrightarrow{OE}+\overrightarrow{EA}\right)+\left(\overrightarrow{OF}+\overrightarrow{FB}\right)+\left(\overrightarrow{OE}+\overrightarrow{EC}\right)+\left(\overrightarrow{OF}+\overrightarrow{FD}\right)\)
\(=2\left(\overrightarrow{OE}+\overrightarrow{EF}\right)+\left(\overrightarrow{EA}+\overrightarrow{EC}\right)+\left(\overrightarrow{FB}+\overrightarrow{FD}\right)\)
\(=2.\overrightarrow{0}+\overrightarrow{0}+\overrightarrow{0}=\overrightarrow{0}\)