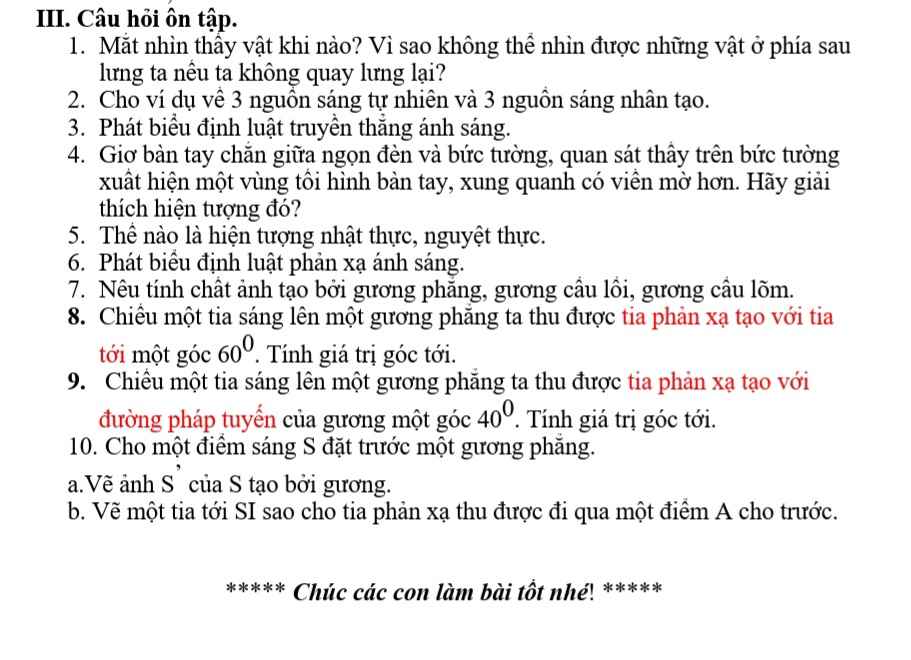
Giúp mình với, mình đang cần rất gấp!!!
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(n_{Fe_3O_4}=\dfrac{8}{232}=\dfrac{1}{29}\left(mol\right)\)
\(Fe_3O_4+8HCl\rightarrow FeCl_2+2FeCl_3+4H_2O\)
\(\dfrac{1}{29}.......\dfrac{8}{29}................\dfrac{2}{29}\)
\(m_{HCl}=\dfrac{8}{29}\cdot36.5=10.06\left(g\right)\)
\(m_{FeCl_3}=\dfrac{2}{29}\cdot162.5=11.21\left(g\right)\)

3. are there any eggs?-No, there aren't some
4.is there any salt?- yes, there is any
5.are there any carrots?-yes, there are some
6.are there any apples?-no there aren't some
7.is there any suger?-yes, there is any
8.are there any cakes?-no there aren't some
9.is there any butter?-no. there isn't any
10. is there any mineral water?- yes. there is any

a: \(A=\sqrt{x^2+2x+5}=\sqrt{x^2+2x+1+4}\)
=>\(A=\sqrt{\left(x+1\right)^2+4}>=\sqrt{4}=2\)
b: \(B=\sqrt{x^2-4x+4+1}=\sqrt{\left(x-2\right)^2+1}>=1\)

Bài 1:
a) \(=\dfrac{\sqrt{5}.\sqrt{7}}{5}=\dfrac{\sqrt{35}}{5}\)
b) \(=\dfrac{\left|y\right|}{\sqrt{3}}=\dfrac{\sqrt{3}y}{3}\)
c) \(=\dfrac{\sqrt{2}}{\sqrt{t}}=\dfrac{\sqrt{2t}}{t}\)
d) \(=\sqrt{\dfrac{7p^2-3p^2}{7}}=\sqrt{\dfrac{4p^2}{7}}=\dfrac{2\left|p\right|}{\sqrt{7}}=\dfrac{-2\sqrt{7}p}{7}\)
Bài 2:
a) \(=\dfrac{\sqrt{21}-\sqrt{15}}{3}\)
b) \(=\dfrac{10\left(4+3\sqrt{2}\right)}{16-18}=-20-15\sqrt{2}\)
c) \(=\dfrac{\left(3\sqrt{10}-5\right)\left(6+\sqrt{10}\right)}{36-10}=\dfrac{18\sqrt{10}+30-30-5\sqrt{10}}{26}=\dfrac{13\sqrt{10}}{26}=\dfrac{\sqrt{10}}{2}\)

\(a) Mg + 2CH_3COOH \to (CH_3COO)_2Mg + H_2\\ n_{H_2} = n_{Mg} = \dfrac{9,6}{24} = 0,4(mol)\\ V_{H_2} = 0,4.22,4 = 8,96(lít)\\ b) n_{(CH_3COO)_2Mg} = n_{Mg} = 0,4(mol)\\ m_{Muối} = 0,4.142 = 56,8(gam)\\ c) n_{CH_3COOH} = 2n_{Mg} = 0,8(mol)\\ m_{dd\ CH_3COOH} = \dfrac{0,8.60}{6\%} = 800(gam)\\ d) C_2H_5OH + O_2 \xrightarrow{men\ giấm} CH_3COOH + H_2O\\ n_{C_2H_5OH} = n_{CH_3COOH} = 0,8(mol)\\ m_{C_2H_5OH} = 0,8.46 = 36,8(gam)\)

Gọi O là trọng tâm tam giác ABC.
Dựng hình bình hành ABCE.
Ta có \(\overrightarrow{MA}+\overrightarrow{MB}+\overrightarrow{MC}=3\overrightarrow{MO}\).
\(\overrightarrow{MA}-\overrightarrow{MB}+\overrightarrow{MC}=\overrightarrow{BA}+\overrightarrow{MC}=\overrightarrow{CE}+\overrightarrow{MC}=\overrightarrow{ME}\).
Từ đó \(T=3MO+3ME\ge3OE\).
Dấu bằng xảy ra khi và chỉ khi M là giao của OE và AC, tức M là trung điểm của AC.
Vậy...

 Giúp mình với mình đang cần rất gấp , vì mình đang thi
Giúp mình với mình đang cần rất gấp , vì mình đang thi
 Các bạn giúp mình với mình đang cần rất gấp, giúp mình nha!!!!!!!!!
Các bạn giúp mình với mình đang cần rất gấp, giúp mình nha!!!!!!!!!


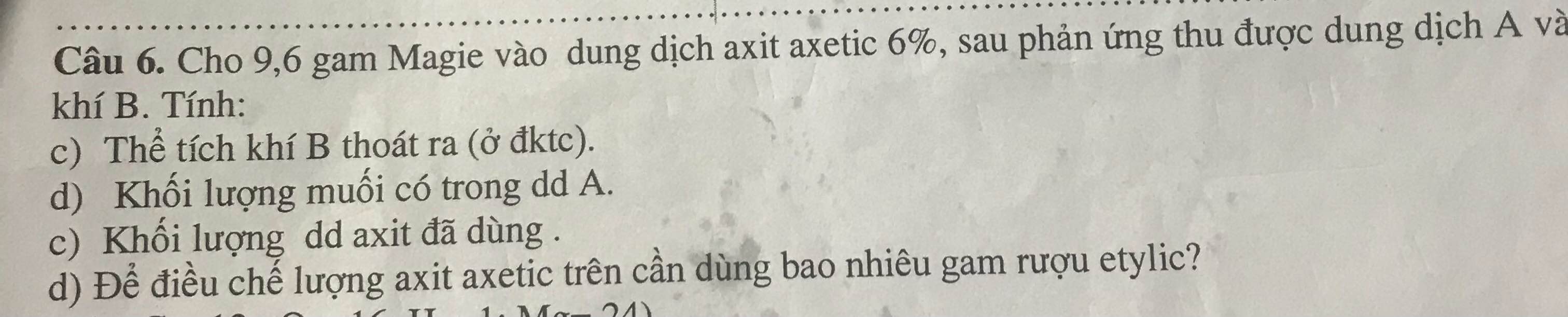

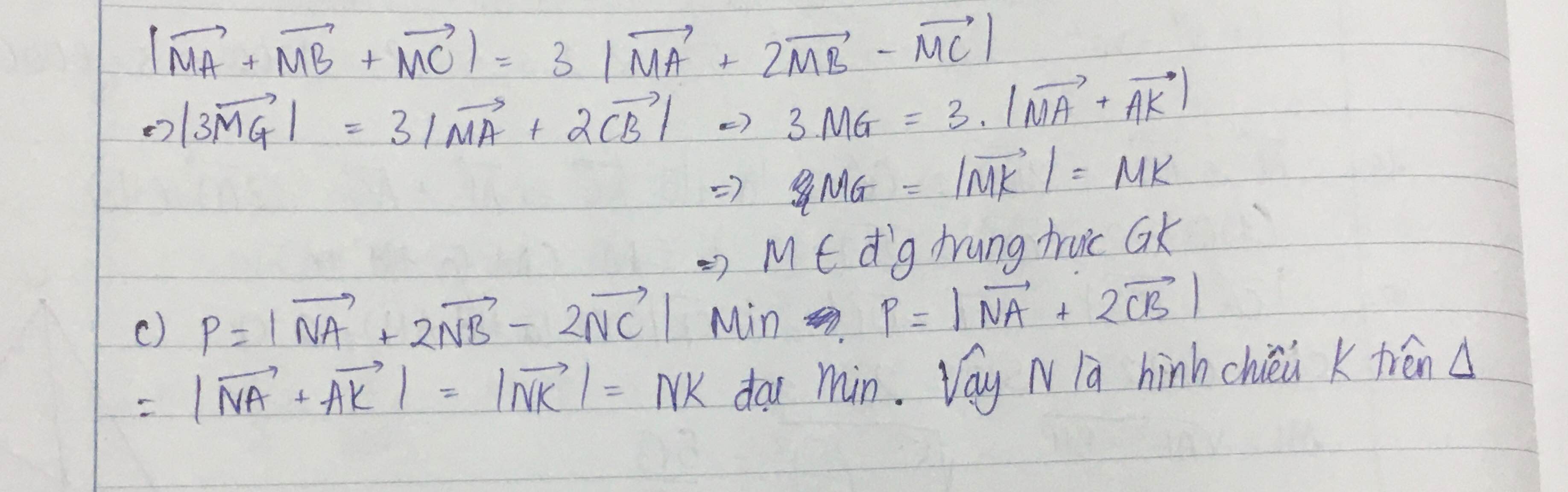 Từ câu a suy ra đc vecto AK = 2 lần vecto CB nhé.
Từ câu a suy ra đc vecto AK = 2 lần vecto CB nhé.
\(f\left(4\right)=16a+4b+c=8a+4\left(2a+b\right)+c=8a+c\) (do \(2a+b=0\))
\(f\left(-2\right)=4a-2b+c=8a-2\left(2a+b\right)+c=8a+c\)
\(\Rightarrow f\left(4\right).f\left(-2\right)=\left(8a+c\right)^2>0\) với mọi a;c (do \(8a+c\ne0\))
\(\Rightarrow f\left(4\right)\) và \(f\left(-2\right)\) cùng dấu