nếu muốn tìm x của F[x] = 32/2 biet nghiem la 1
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Lời giải:
Ta có:\(F(x)=\int (2x-3)\ln xdx\)
Đặt \(\left\{\begin{matrix} u=\ln x\\ dv=(2x-3)dx\end{matrix}\right.\Rightarrow \left\{\begin{matrix} du=\frac{dx}{x}\\ v=\int (2x-3)dx=x^2-3x\end{matrix}\right.\)
Do đó:
\(F(x)=\int (2x-3)\ln xdx=(x^2-3x)\ln x-\int (x^2-3x).\frac{dx}{x}\)
\(=(x^2-3x)\ln x-\int (x-3)dx=(x^2-3x)\ln x-(\frac{x^2}{2}-3x)+c\)
Với \(x=1\)
\(F(1)=\frac{5}{2}+c=0\Rightarrow c=\frac{-5}{2}\)
Vậy \(F(x)=(x^2-3x)\ln x-\frac{x^2}{2}+3x-\frac{5}{2}\)
\(\Rightarrow 2F(x)+x^2-6x+5=2(x^2-3x)\ln x-x^2+6x-5+x^2-6x+5\)
\(=2(x^2-3x)\ln x=0\)
\(\Leftrightarrow \left[\begin{matrix} x=0\\ x=3\\ x=1\end{matrix}\right.\)
Tức là pt có 3 nghiệm.

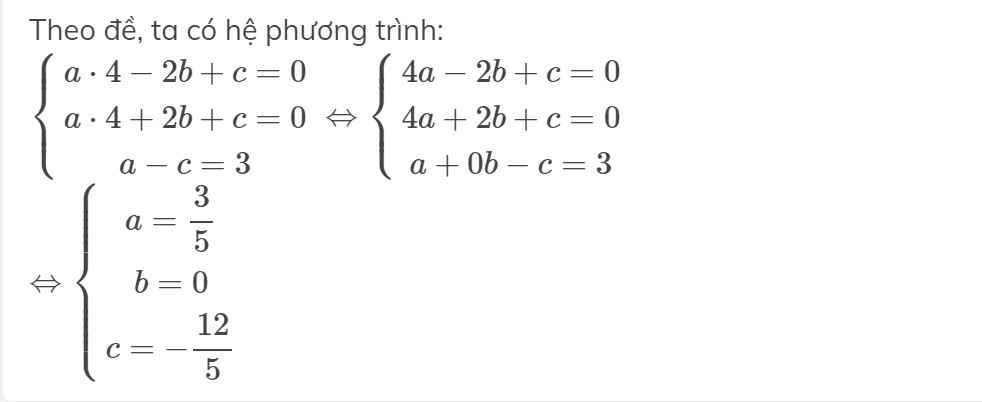
Câu 2:
Theo đề, ta có:
\(\left\{{}\begin{matrix}a-c=3\\f\left(2\right)=0\\f\left(-2\right)=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\left(c+3\right)\cdot2^2+b\cdot2+c=0\\\left(c+3\right)\cdot\left(-2\right)^2+b\cdot\left(-2\right)+c=0\\a=c+3\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}4\left(c+3\right)+2b+c=0\\4\left(c+3\right)-2b+c=0\\a=c+3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}5c+12+2b=0\\5c+12-2b=0\\a=c+3\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}b=0\\c=-\dfrac{12}{5}\\a=c+3=-\dfrac{12}{5}+3=\dfrac{3}{5}\end{matrix}\right.\)

x = 32/2 nha bạn
Có thể bạn cho đề sai, biến x trong đa thức f(x) đâu ạ