Giải phương trinh:\(|x+\text{1}|=|x(x+\text{1})|\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
XO
27 tháng 9 2023
ĐKXĐ \(3x^2-5x+1\ge0;x^2-2\ge0;x^2-x-1\ge0\)
Ta có : \(\sqrt{3x^2-5x+1}-\sqrt{x^2-2}=\sqrt{3.\left(x^2-x-1\right)}-\sqrt{x^2-3x+4}\)
\(\Leftrightarrow\sqrt{3x^2-5x+1}-\sqrt{3\left(x^2-x-1\right)}=\sqrt{x^2-2}-\sqrt{x^2-3x+4}\)
\(\Leftrightarrow\dfrac{3x^2-5x+1-3.\left(x^2-x-1\right)}{\sqrt{3x^2-5x+1}+\sqrt{3\left(x^2-x-1\right)}}=\dfrac{x^2-2-x^2+3x-4}{\sqrt{x^2-2}+\sqrt{x^2-3x+4}}\)
\(\Leftrightarrow\dfrac{-2x+4}{\sqrt{3x^2-5x+1}+\sqrt{3\left(x^2-x-1\right)}}=\dfrac{3x-6}{\sqrt{x^2-2}+\sqrt{x^2-3x+4}}\)
\(\Leftrightarrow\left[{}\begin{matrix}x=2\\\dfrac{3}{\sqrt{x^2-2}+\sqrt{x^2-3x+4}}+\dfrac{2}{\sqrt{3x^2-5x+1}+\sqrt{3\left(x^2-x-1\right)}}=0\left(∗\right)\end{matrix}\right.\)
Xét phương trình (*) ta có VT > 0 \(\forall x\) mà VP = 0
nên (*) vô nghiệm
Vậy x = 2 là nghiệm phương trình
4 tháng 10 2023
Giải bằng bất đẳng thức Cô si: (ĐK: \(x^2-x+1\ge0;-2x^2+x+2\ge0;x^2-4x+7\)
Ta có: \(x^2-x+1+1\ge2\sqrt{x^2-x+1}\Leftrightarrow\sqrt{x^2-x+1}\le\dfrac{x^2-x+2}{2}\left(1\right)\\ T,T:\sqrt{-2x^2+x+2}\le\dfrac{-2x^2+x+3}{2}\left(2\right)\\ \left(1\right);\left(2\right)\Rightarrow\sqrt{x^2-x+1}+\sqrt{-2x^2+x+2}\le\dfrac{x^2-x+2-2x^2+x+3}{2}=\dfrac{-x^2+5}{2}\\ \Rightarrow\sqrt{x^2-x+1}+\sqrt{-2x^2+x+2}-\dfrac{x^2-4x+7}{2}\le\dfrac{-x^2+5-x^2+4x-7}{2}\\
=\dfrac{-2x^2+4x-2}{2}\\
=-x^2+2x-1
\\
\Rightarrow-\left(x-1\right)^2\ge0\)
Điều này chỉ thỏa 1 điều kiên khi x-1=0 ⇔x=1(nhận
Vậy x=1 là nghiệm cuả phương trình
MS
1
22 tháng 1 2024
1:
ĐKXĐ: x<>3
\(\dfrac{x-1}{x-3}>1\)
=>\(\dfrac{x-1-\left(x-3\right)}{x-3}>0\)
=>\(\dfrac{x-1-x+3}{x-3}>0\)
=>\(\dfrac{2}{x-3}>0\)
=>x-3>0
=>x>3
2: ĐKXĐ: \(\left[{}\begin{matrix}x>=3\\x< =-4\end{matrix}\right.\)
\(\sqrt{x^2+x-12}< 8-x\)
=>\(\left\{{}\begin{matrix}8-x>=0\\x^2+x-12< \left(8-x\right)^2\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x< =8\\x^2+x-12-x^2+16x-64< 0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x< =8\\17x-76< 0\end{matrix}\right.\)
=>\(x< \dfrac{76}{17}\)
Kết hợp ĐKXĐ, ta được: \(\left[{}\begin{matrix}3< =x< \dfrac{76}{17}\\x< =-4\end{matrix}\right.\)
HN
1
NV
Nguyễn Việt Lâm
Giáo viên
19 tháng 12 2020
ĐKXĐ: ...
\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{3}{x+y}+6x-3y=6\\\dfrac{3}{x+y}+2x-4y=1\end{matrix}\right.\)
\(\Rightarrow4x+y=5\Rightarrow y=5-4x\)
Thế vào phương trình đầu:
\(\dfrac{1}{x+5-4x}+2x-\left(5-4x\right)=2\)
\(\Leftrightarrow\dfrac{1}{5-3x}+6x-7=0\)
\(\Leftrightarrow\left(6x-7\right)\left(5-3x\right)+1=0\)
\(\Leftrightarrow...\)
21 tháng 8 2022
a: Đặt \(x^2-4=a\)
Pt sẽ là \(a=3\sqrt{xa}\)
\(\Rightarrow a^2=9xa\)
\(\Leftrightarrow a\left(a-9x\right)=0\)
\(\Leftrightarrow\left(x^2-4\right)\left(x^2-4-9x\right)=0\)
hay \(x\in\left\{2;-2;\dfrac{9+\sqrt{97}}{2};\dfrac{9-\sqrt{97}}{2}\right\}\)
d: Đặt \(\sqrt{x^2-x+1}=a;\sqrt{x^2+x+1}=b\)
Pt sẽ là 2a+b=ab+2
=>(b-2)(1-a)=0
=>b=2 và 1-a
\(\Leftrightarrow\left\{{}\begin{matrix}x^2+x+1=4\\x^2-x+1=1\end{matrix}\right.\Leftrightarrow x\in\varnothing\)
29 tháng 8 2015
Điều kiện xác định phương trình \(x\ge\frac{1}{4}\).
Nhân cả hai vế với \(\sqrt{2}\) phương trình tương đương với
\(\sqrt{4x-2\sqrt{4x-1}}-\sqrt{4x+2\sqrt{4x-1}=4}\leftrightarrow\left|\sqrt{4x-1}-1\right|-\left|\sqrt{4x-1}+1\right|=4\)
\(\leftrightarrow\left|\sqrt{4x-1}-1\right|-\sqrt{4x-1}=5\).
Trường hợp 1. NẾU \(x\ge\frac{1}{2}\to\sqrt{4x-1}-1-\sqrt{4x-1}=5\to\) loại
Trường hợp 2. NẾU \(\frac{1}{4}\le x<\frac{1}{2}\to-\sqrt{4x-1}+1-\sqrt{4x-1}=5\to-2\sqrt{4x-1}=4\to\) loại
Vậy phương trình vô nghiệm.
29 tháng 1 2020
Mình gửi đề ạ, chứ sao trên đó nó không hiện đề
\(\begin{cases} x.\sqrt[\text{2}]{\text{1-$y^{2}$}}+y.\sqrt[\text{2}]{\text{1-$x^{2}$}} (1)\\ x+y=1 (2) \end{cases} \)
8 tháng 3 2022
\(\dfrac{x-2}{x+1}-\dfrac{3}{x+2}>0.\left(x\ne-1;-2\right).\\ \Leftrightarrow\dfrac{x^2-4-3x-3}{\left(x+1\right)\left(x+2\right)}>0.\\ \Leftrightarrow\dfrac{x^2-3x-7}{\left(x+1\right)\left(x+2\right)}>0.\)
Đặt \(f\left(x\right)=\dfrac{x^2-3x-7}{\left(x+1\right)\left(x+2\right)}>0.\)
Ta có: \(x^2-3x-7=0.\Rightarrow\left[{}\begin{matrix}x=\dfrac{3+\sqrt{37}}{2}.\\x=\dfrac{3-\sqrt{37}}{2}.\end{matrix}\right.\)
\(x+1=0.\Leftrightarrow x=-1.\\ x+2=0.\Leftrightarrow x=-2.\)
Bảng xét dấu:
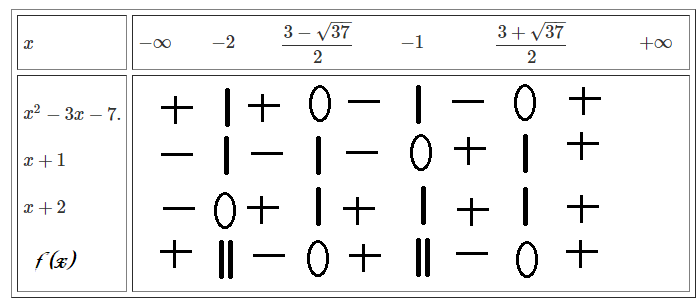
\(\Rightarrow f\left(x\right)>0\Leftrightarrow x\in\left(-\infty-2\right)\cup\left(\dfrac{3-\sqrt{37}}{2};-1\right)\cup\left(\dfrac{3+\sqrt{37}}{2};+\infty\right).\)
\(\sqrt{x^2-3x+2}\ge3.\\ \Leftrightarrow x^2-3x+2\ge9.\\ \Leftrightarrow x^2-3x-7\ge0.\)
\(\Rightarrow\left[{}\begin{matrix}x=\dfrac{3-\sqrt{37}}{2}.\\x=\dfrac{3+\sqrt{37}}{2}.\end{matrix}\right.\)
Đặt \(f\left(x\right)=x^2-3x-7.\)
\(f\left(x\right)=x^2-3x-7.\)
\(\Rightarrow f\left(x\right)\ge0\Leftrightarrow x\in(-\infty;\dfrac{3-\sqrt{37}}{2}]\cup[\dfrac{3+\sqrt{37}}{2};+\infty).\)
\(\Rightarrow\sqrt{x^2-3x+2}\ge3\Leftrightarrow x\in(-\infty;\dfrac{3-\sqrt{37}}{2}]\cup[\dfrac{3+\sqrt{37}}{2};+\infty).\)
PT <=> \(\left(x+1\right)^2=\left[x\left(x+1\right)\right]^2\)
\(\Leftrightarrow\left(x+1\right)^2=x^2\left(x+1\right)^2\)
\(\Leftrightarrow\left(x+1\right)^2-x^2\left(x+1\right)^2=0\)
\(\Leftrightarrow\left(1-x\right)\left(x+1\right)^3=0\Leftrightarrow x=\pm1\)
Vậy tập nghiệm của phương trình là S = { 1 ; -1 }
có 1 cách giải khác nữa áp dụng với công thức tổng quát này
\(\left|f\left(x\right)\right|=\left|g\left(x\right)\right|\Rightarrow\orbr{\begin{cases}f\left(x\right)=g\left(x\right)\\f\left(x\right)=-g\left(x\right)\end{cases}}\)