Cho hàm số f(x) = 1 3 x 3 + x 2 - 3 x + 1 . Tìm nghiệm của bất phương trình f ' ( x ) ≤ 0
![]()
![]()
![]()
![]()
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

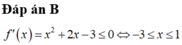

Ta có: \(f'\left(x\right)=x^2-2x-3\)
\(f'\left(x\right)\le0\\ \Rightarrow x^2-2x-3\le0\\ \Leftrightarrow\left(x+1\right)\left(x-3\right)\le0\\ \Leftrightarrow-1\le x\le3\)

2: ĐKXĐ: x<>1
\(f'\left(x\right)=\dfrac{\left(x^2-3x+3\right)'\left(x-1\right)-\left(x^2-3x+3\right)\left(x-1\right)'}{\left(x-1\right)^2}\)
\(=\dfrac{\left(2x-3\right)\left(x-1\right)-\left(x^2-3x+3\right)}{\left(x-1\right)^2}\)
\(=\dfrac{2x^2-5x+3-x^2+3x-3}{\left(x-1\right)^2}=\dfrac{x^2-2x}{\left(x-1\right)^2}\)
f'(x)=0
=>x^2-2x=0
=>x(x-2)=0
=>\(\left[{}\begin{matrix}x=0\\x=2\end{matrix}\right.\)
1:
\(f\left(x\right)=\dfrac{1}{3}x^3-2\sqrt{2}\cdot x^2+8x-1\)
=>\(f'\left(x\right)=\dfrac{1}{3}\cdot3x^2-2\sqrt{2}\cdot2x+8=x^2-4\sqrt{2}\cdot x+8=\left(x-2\sqrt{2}\right)^2\)
f'(x)=0
=>\(\left(x-2\sqrt{2}\right)^2=0\)
=>\(x-2\sqrt{2}=0\)
=>\(x=2\sqrt{2}\)

\(f\left(x-3\right)+5-m>0\)
\(\Leftrightarrow\left(x-3\right)^2+5-m>0\)
\(\Leftrightarrow x^2-6x+14-m>0\)
BPT có tập nghiệm là R khi:
\(\Delta'=9-\left(14-m\right)< 0\)
\(\Leftrightarrow m< 5\)

Với m=−1m=−1 thì PT f(x)=0f(x)=0 có nghiệm x=1x=1 (chọn)
Với m≠−1m≠−1 thì f(x)f(x) là đa thức bậc 2 ẩn xx
f(x)=0f(x)=0 có nghiệm khi mà Δ′=m2−2m(m+1)≥0Δ′=m2−2m(m+1)≥0
⇔−m2−2m≥0⇔m(m+2)≤0⇔−m2−2m≥0⇔m(m+2)≤0
⇔−2≤m≤0⇔−2≤m≤0
Tóm lại để f(x)=0f(x)=0 có nghiệm thì m∈[−2;0]

Ta có:
\(f'\left(x\right)=6x^2-2x\\ g'\left(x\right)=3x^2+x\)
Theo đề bài, ta có:
\(f'\left(x\right)>g'\left(x\right)\\ \Leftrightarrow6x^2-2x>3x^2+x\\ \Leftrightarrow3x^2-3x>0\\ \Leftrightarrow3x\left(x-1\right)>0\\ \Leftrightarrow\left[{}\begin{matrix}x>1\\x< 0\end{matrix}\right.\)
Vậy tập nghiệm của bất phương trình là \(\left(-\infty;0\right)\cup\left(1;+\infty\right)\)
Chọn D.