Cho x+\(\sqrt{3}=2\)\(.Tính\) giá trị biểu thức H= \(x^5-3x^4-3x^3+6x^2-20x+2024\)ta được
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
PM
1
NH
5 tháng 10 2019
Ta có: \(x=2-\sqrt{3}\)\(\Rightarrow2-x=\sqrt{3}\)\(\Rightarrow\left(2-x\right)^2=3\)\(\Rightarrow4-4x+x^2=3\)\(\Rightarrow x^2-4x+1=0\)
Lại có: \(B=x^5-3x^4-3x^3+6x^2-20x+2018\)
\(\Rightarrow B=x^5-4x^4+x^4+x^3-4x^3+5x^2+x^2+20x+5+2013\)
\(\Rightarrow B=\left(x^5-4x^4+x^3\right)+\left(x^4-4x^3+x^2\right)+\left(5x^2-20x+5\right)+2013\)
\(\Rightarrow B=x^3\left(x^2-4x+1\right)+x^2\left(x^2-4x+1\right)+5\left(x^2-4x+1\right)+2013\)
\(\Rightarrow B=x^3\cdot0+x^2\cdot0+5\cdot0+2013=2013\)
21 tháng 7 2018
b) Ta có: \(x+\sqrt{3}=2\Leftrightarrow x-2=-\sqrt{3}\Leftrightarrow\left(x-2\right)^2=3\Leftrightarrow x^2-4x+1=0\)
\(B=x^5-3x^4-3x^3+6x^2-20x+2021\)
\(B=\left(x^5-4x^4+x^3\right)+\left(x^4-4x^3+x^2\right)+\left(5x^2-20x+5\right)+2016\)
\(B=x^3\left(x^2-4x+1\right)+x^2\left(x^2-4x+1\right)+5\left(x^2-4x+1\right)+2016\)
Thế \(x^2-4x+1=0\)\(\Rightarrow B=2016.\)
6 tháng 11 2016
Theo đề ta có
\(x=2-\sqrt{3}\)
\(\Rightarrow\left(4-x\right)x=\left(2+\sqrt{3}\right)\left(2-\sqrt{3}\right)=1\)
Q = x5 - 3x4 - 3x3 + 6x2 - 20x + 2020
= (x5 - 4x4) + (x4 - 4x3) + (x3 - 4x2) + (10x2 - 40x) + 20x + 2020
= - x3 - x2 - x - 10 + 20x + 2020
= (- x3 + 4x2) + ( - 5x2 + 20x) - x + 2010
= x + 5 - x + 2010 = 2015
12 tháng 7 2023
\(x=\sqrt{\dfrac{2\sqrt{3}+2-6\sqrt{3}}{2\sqrt{3}\left(2\sqrt{3}+2\right)}}=\sqrt{\dfrac{2-4\sqrt{3}}{2\sqrt{3}\left(2\sqrt{3}+2\right)}}\) ko tồn tại vì 2-4căn 3<0
ND
0
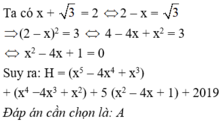
\(\Leftrightarrow x=2-\sqrt{3}\)
Dễ thấy x là nghiệm của PT \(x^2-4x+1\)
\(H=\left(x^5-4x^4+x^3\right)+\left(x^4-4x^3+x^2\right)+\left(5x^2-20x+5\right)+2019\\ H=\left(x^2-4x+1\right)\left(x^3+x^2+5\right)+2019\\ H=2019\)