Giúp mk phần B ạ 
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


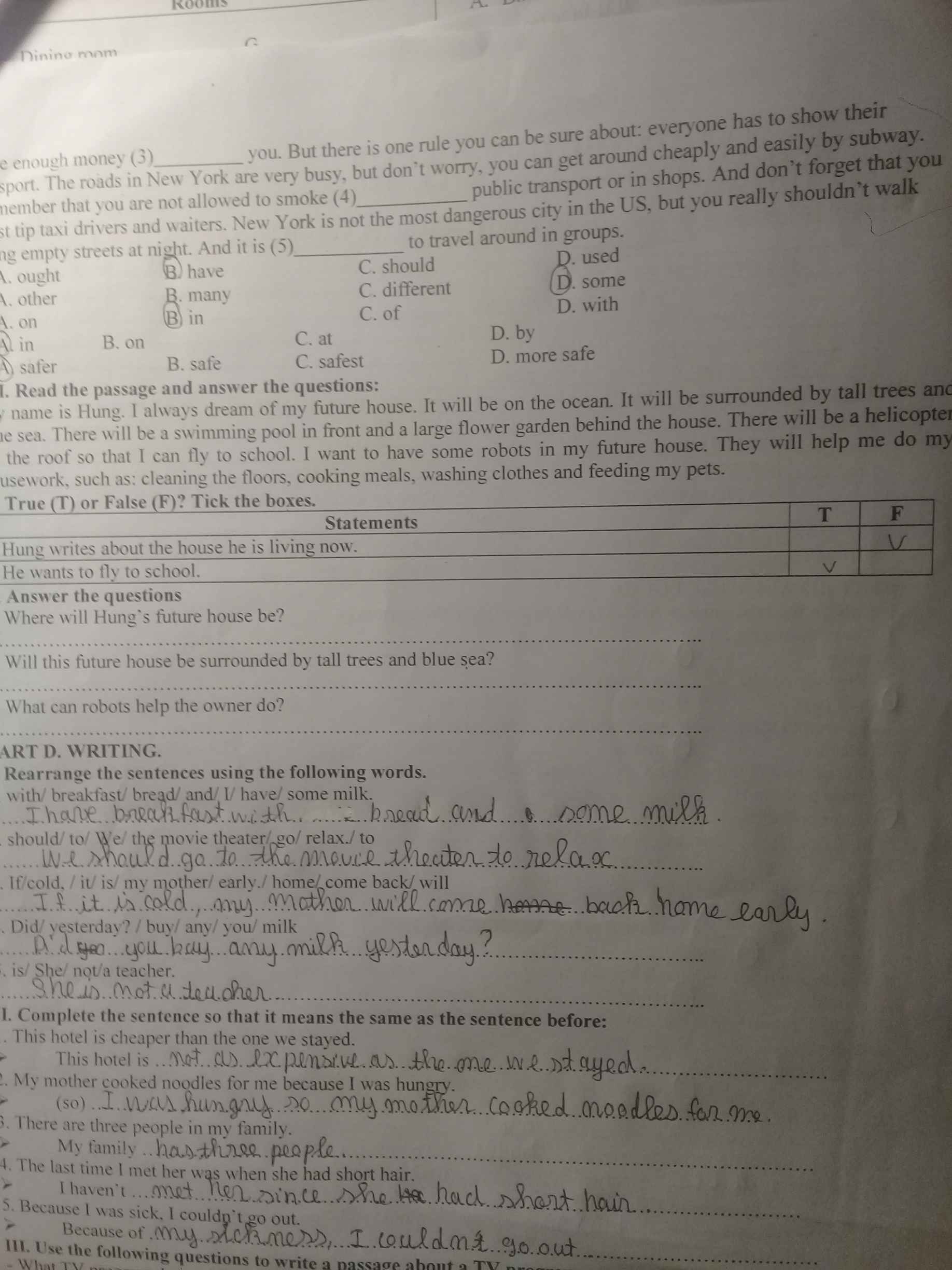
1. On the ocean
2. Yes
3. do house work , cleaning the floor , cooking meals , washing clothes , feeding his pets .

Bài 3:
b: \(B_1=-\left|2x-3\right|+2\le2\forall x\)
Dấu '=' xảy ra khi \(x=\dfrac{3}{2}\)
\(B_2=-\left|x+4\right|+5\le5\forall x\)
Dấu '=' xảy ra khi x=-4
Bài 3:
b) Xét số \(-B_3=6+\left|x+4\right|\ge6\Rightarrow B_3\le-6\)
Dấu '=' xảy ra \(\Leftrightarrow x=-4\)


Bài 4:
Nhóm 1: x;1/3x; 8x
Nhóm 2: \(x^2;5x^2;-3x^2\)


1.
a, \(\left(C\right)x^2+y^2-6x-2y+6=0\)
\(\Leftrightarrow\left(C\right)\left(x-3\right)^2+\left(y-1\right)^2=4\)
\(\Rightarrow\) Tâm \(I=\left(3;1\right)\), bán kính \(R=2\)
b, Tiếp tuyến đi qua A có dạng: \(\left(\Delta\right)ax+by-5a-7b=0\left(a^2+b^2\ne0\right)\)
Ta có: \(d\left(I;\Delta\right)=\dfrac{\left|3a+b-5a-7b\right|}{\sqrt{a^2+b^2}}=2\)
\(\Leftrightarrow\left|a+3b\right|=\sqrt{a^2+b^2}\)
\(\Leftrightarrow6ab+8b^2=0\)
\(\Leftrightarrow2b\left(3a+4b\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}b=0\\3a+4b=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\Delta_1:x=5\\\Delta_2:4x-3y+1=0\end{matrix}\right.\)
TH1: \(\Delta_1:x=5\)
Tiếp điểm có tọa độ là nghiệm hệ: \(\left\{{}\begin{matrix}x=5\\x^2+y^2-6x-2y+6=0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=5\\y^2-2y+1=0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=5\\y=1\end{matrix}\right.\Rightarrow\left(5;1\right)\)
TH2: \(\Delta_2:4x-3y+1=0\)
Tiếp điểm có tọa độ là nghiệm hệ: \(\left\{{}\begin{matrix}4x-3y+1=0\\x^2+y^2-6x-2y+6=0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{7}{5}\\y=\dfrac{11}{5}\end{matrix}\right.\Rightarrow\left(\dfrac{7}{5};\dfrac{11}{5}\right)\)
Kết luận: Phương trình tiếp tuyến: \(\left\{{}\begin{matrix}\Delta_1:x=5\\\Delta_2:4x-3y+1=0\end{matrix}\right.\)
Tọa độ tiếp điểm: \(\left\{{}\begin{matrix}\left(5;1\right)\\\left(\dfrac{7}{5};\dfrac{11}{5}\right)\end{matrix}\right.\)

5.
\(n_{Na}=a\left(mol\right)\)
\(Na+H_2O\rightarrow NaOH+\dfrac{1}{2}H_2\)
\(a...............a.....0.5a\)
\(m_{\text{dung dịch sau phản ứng}}=23a+500-0.5a\cdot2=22a+500\left(g\right)\)
\(m_{NaOH}=40a\left(g\right)\)
\(C\%_{NaOH}=\dfrac{40a}{22a+500}\cdot100\%=20\%\)
\(\Rightarrow a=2.8\)
\(b.\)
\(n_{H^+}=10^{-3}\cdot V\cdot\left(1+0.5\cdot2\right)=2\cdot10^{-3}V\left(mol\right)\)
\(\Rightarrow n_{NaOH}=2\cdot10^{-3}V=2.8\left(mol\right)\)
\(\Rightarrow V=1400\)

1. \(P=\dfrac{\sqrt{x}+4}{\sqrt{x}-1}:\left(\dfrac{3\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}-\dfrac{2}{\sqrt{x}+3}\right)\)
\(P=\dfrac{\sqrt{x}+4}{\sqrt{x}-1}:\left(\dfrac{3\sqrt{x}+1-2\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}\right)\)
\(P=\dfrac{\sqrt{x}+4}{\sqrt{x}-1}:\left(\dfrac{3\sqrt{x}+1-2\sqrt{x}+2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}\right)\)
\(P=\dfrac{\sqrt{x}+4}{\sqrt{x}-1}:\left(\dfrac{\sqrt{x}+3}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}\right)\)
\(P=\dfrac{\sqrt{x}+4}{\sqrt{x}-1}.\sqrt{x}-1\)
P=\(\sqrt{x}+4\)
b) \(P=\dfrac{x}{4}+5\)
⇔\(\sqrt{x}+4=\dfrac{x}{4}+5\)
⇔\(\dfrac{x}{4}-\sqrt{x}+1=0\)
⇔\(x-4\sqrt{x}+4=0\)
⇔\(\left(\sqrt{x}-2\right)^2=0\)
⇔\(\sqrt{x}-2=0\)
⇔\(\sqrt{x}=2\)
⇔\(x=4\)
Vậy x=4 thì P=\(\dfrac{x}{4}+5\)
Bài 1:
a) Ta có: \(P=\dfrac{\sqrt{x}+4}{\sqrt{x}-1}:\left(\dfrac{3\sqrt{x}+1}{x+2\sqrt{x}-3}-\dfrac{2}{\sqrt{x}+3}\right)\)
\(=\dfrac{\sqrt{x}+4}{\sqrt{x}-1}:\left(\dfrac{3\sqrt{x}+1}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-1\right)}-\dfrac{2\left(\sqrt{x}-1\right)}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-1\right)}\right)\)
\(=\dfrac{\sqrt{x}+4}{\sqrt{x}-1}:\dfrac{\sqrt{x}+3}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-1\right)}\)
\(=\sqrt{x}+4\)
b) Ta có: \(P=\dfrac{x}{4}+5\)
\(\Leftrightarrow\sqrt{x}+4=\dfrac{1}{4}x+5\)
\(\Leftrightarrow\dfrac{1}{4}x-\sqrt{x}+1=0\)
\(\Leftrightarrow x-4\sqrt{x}+4=0\)
\(\Leftrightarrow x=4\)
 Các bạn giúp mk mỗi bài 3 phần b với phần c hoi ạ><
Các bạn giúp mk mỗi bài 3 phần b với phần c hoi ạ>< giúp mk phần mk khoanh ạ . mk cảm ơn ạ
giúp mk phần mk khoanh ạ . mk cảm ơn ạ 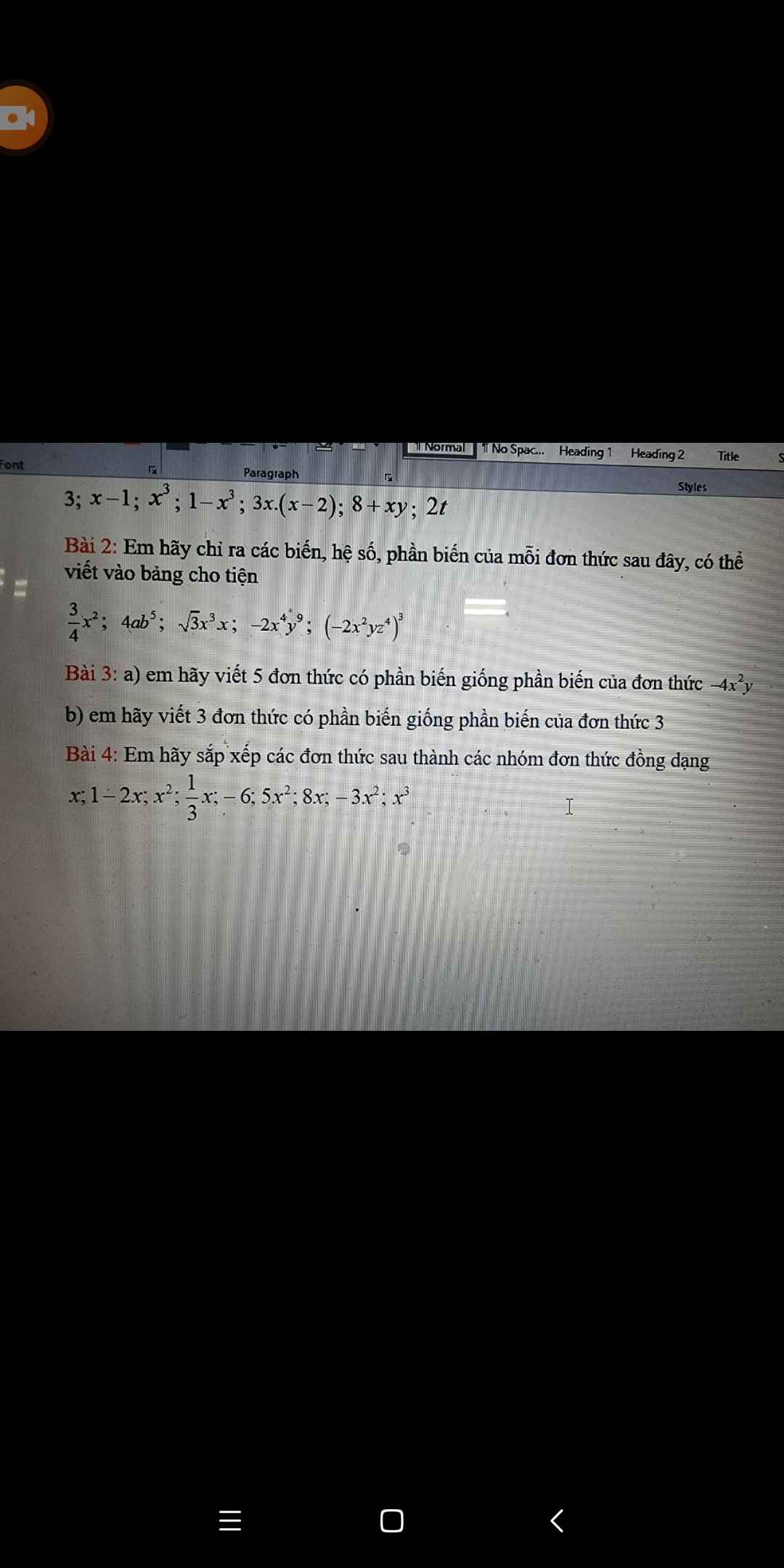




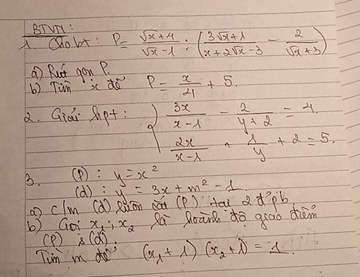
1 Yes, it can
2 It can cause heal problems such as eye-tiredness and obesity
3 No, I won't
4 We should use it for a limited time
Dạ cảm ơn ạ:>