Giải các bất phương trình sau:
a) b)
c) d)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

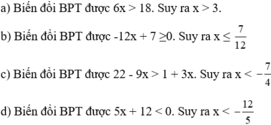

a, -2x>15 x>-15/2 c, th1 x+2>0 vs x+3 <0 suy ra x>-2 vs x<-3 . th2 x+2<0,x+3>0 suy ra x<-2 ,x>-3
b, 112-x2>0
x2<112 x<11
a) \(3x-8>5x+7\)
\(\Leftrightarrow-8>5x+7-3x\)
\(\Leftrightarrow-8>2x+7\)
\(\Leftrightarrow-8-7>2x\)
\(\Leftrightarrow-15>2x\)
\(\Leftrightarrow-\frac{15}{2}>x\)
\(\Rightarrow x< -\frac{15}{2}\)
b) \(\left(11-x\right)\left(11+x\right)>0\)
\(\Leftrightarrow x=\pm11\)
\(\Rightarrow-11< x< 11\)
c) \(\left(x+2\right)\left(x+3\right)< 0\)
\(\Leftrightarrow x=-2;-3\)
\(\Rightarrow-3< x< -2\)

\(a,\left(\dfrac{1}{3}\right)^{2x+1}\le9\\ \Leftrightarrow2x+1\ge-2\\ \Leftrightarrow2x\ge-3\\ \Leftrightarrow x\ge-\dfrac{3}{2}\)
\(b,4^x>2^{x-2}\\ \Leftrightarrow2^{2x}>2^{x-2}\\ \Leftrightarrow2x>x-2\\ \Leftrightarrow x>-2\)

a) Ta có \(a = 3 > 0\) và tam thức bậc hai \(f\left( x \right) = 3{x^2} - 2x + 4\) có \(\Delta ' = {1^2} - 3.4 = - 11 < 0\)
=> \(f\left( x \right) = 3{x^2} - 2x + 4\) vô nghiệm.
=> \(3{x^2} - 2x + 4 > 0\forall x \in \mathbb{R}\)
b) Ta có: \(a = - 1 < 0\) và \(\Delta ' = {3^2} - \left( { - 1} \right).\left( { - 9} \right) = 0\)
=> \(f\left( x \right) = - {x^2} + 6x - 9\) có nghiệm duy nhất \(x = 3\).
=> \( - {x^2} + 6x - 9 < 0\forall x \in \mathbb{R}\backslash \left\{ 3 \right\}\)

\(a,0,1^{2-x}>0,1^{4+2x}\\ \Leftrightarrow2-x>2x+4\\ \Leftrightarrow3x< -2\\ \Leftrightarrow x< -\dfrac{2}{3}\)
\(b,2\cdot5^{2x+1}\le3\\ \Leftrightarrow5^{2x+1}\le\dfrac{3}{2}\\ \Leftrightarrow2x+1\le log_5\left(\dfrac{3}{2}\right)\\ \Leftrightarrow2x\le log_5\left(\dfrac{3}{2}\right)-1\\ \Leftrightarrow x\le\dfrac{1}{2}log_5\left(\dfrac{3}{2}\right)-\dfrac{1}{2}\\ \Leftrightarrow x\le log_5\left(\dfrac{\sqrt{30}}{10}\right)\)
c, ĐK: \(x>-7\)
\(log_3\left(x+7\right)\ge-1\\ \Leftrightarrow x+7\ge\dfrac{1}{3}\\ \Leftrightarrow x\ge-\dfrac{20}{3}\)
Kết hợp với ĐKXĐ, ta có:\(x\ge-\dfrac{20}{3}\)
d, ĐK: \(x>\dfrac{1}{2}\)
\(log_{0,5}\left(x+7\right)\ge log_{0,5}\left(2x-1\right)\\ \Leftrightarrow x+7\le2x-1\\ \Leftrightarrow x\ge8\)
Kết hợp với ĐKXĐ, ta được: \(x\ge8\)

a) Xét tam thức \(f\left( x \right) = 7{x^2} - 19x - 6\) có \(\Delta = 529 > 0\), có hai nghiệm phân biệt \({x_1} = - \frac{2}{7},{x_2} = 3\) và có \(a = 7 > 0\)
Ta có bảng xét dấu như sau
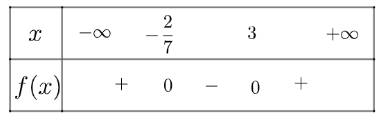
Vậy nghiệm của bất phương trình là đoạn \(\left[ { - \frac{2}{7};3} \right]\)
b) \( - 6{x^2} + 11x > 10 \Leftrightarrow - 6{x^2} + 11x - 10 > 0\)
Xét tam thức \(f\left( x \right) = - 6{x^2} + 11x - 10\) có \(\Delta = - 119 < 0\)và có \(a = - 6 < 0\)
Ta có bảng xét dấu như sau

Vậy bất phương trình vô nghiệm
c) \(3{x^2} - 4x + 7 > {x^2} + 2x + 1 \Leftrightarrow 2{x^2} - 6x + 6 > 0\)
Xét tam thức \(f\left( x \right) = 2{x^2} - 6x + 6\) có \(\Delta = - 12 < 0\)và có \(a = 2 > 0\)
Ta có bảng xét dấu như sau
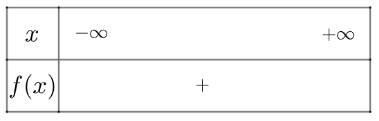
Vậy bất phương trình có vô số nghiệm
d) Xét tam thức \(f\left( x \right) = {x^2} - 10x + 25\) có \(\Delta = 0\), có nghiệm kép \({x_1} = {x_2} = 5\) và có \(a = 1 > 0\)
Ta có bảng xét dấu như sau
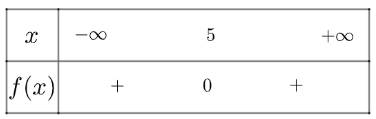
Vậy nghiệm của bất phương trình là \(x = 5\)

a) \({\log _{\frac{1}{7}}}\left( {x + 1} \right) > {\log _7}\left( {2 - x} \right)\) (ĐK: \(x + 1 > 0;2 - x > 0 \Leftrightarrow - 1 < x < 2\))
\(\begin{array}{l} \Leftrightarrow {\log _{{7^{ - 1}}}}\left( {x + 1} \right) > {\log _7}\left( {2 - x} \right)\\ \Leftrightarrow - {\log _7}\left( {x + 1} \right) > {\log _7}\left( {2 - x} \right)\\ \Leftrightarrow {\log _7}{\left( {x + 1} \right)^{ - 1}} > {\log _7}\left( {2 - x} \right)\\ \Leftrightarrow {\left( {x + 1} \right)^{ - 1}} > 2 - x\\ \Leftrightarrow \frac{1}{{x + 1}} - 2 + x > 0\\ \Leftrightarrow \frac{{1 + \left( {x - 2} \right)\left( {x + 1} \right)}}{{x + 1}} > 0\\ \Leftrightarrow \frac{{1 + {x^2} - x - 2}}{{x + 1}} > 0 \Leftrightarrow \frac{{{x^2} - x - 1}}{{x + 1}} > 0\end{array}\)
Mà – 1 < x < 2 nên x + 1 > 0
\( \Leftrightarrow {x^2} - x - 1 > 0 \Leftrightarrow \left[ \begin{array}{l}x < \frac{{1 - \sqrt 5 }}{2}\\x > \frac{{1 + \sqrt 5 }}{2}\end{array} \right.\)
KHĐK ta có \(\left[ \begin{array}{l} - 1 < x < \frac{{1 - \sqrt 5 }}{2}\\\frac{{1 + \sqrt 5 }}{2} < x < 2\end{array} \right.\)
b) \(2\log \left( {2x + 1} \right) > 3\) (ĐK: \(2x + 1 > 0 \Leftrightarrow x > \frac{{ - 1}}{2}\))
\(\begin{array}{l} \Leftrightarrow \log \left( {2x + 1} \right) > \frac{3}{2}\\ \Leftrightarrow 2x + 1 > {10^{\frac{3}{2}}} = 10\sqrt {10} \\ \Leftrightarrow x > \frac{{10\sqrt {10} - 1}}{2}\end{array}\)
KHĐK ta có \(x > \frac{{10\sqrt {10} - 1}}{2}\)

a.\(x^2-25=8\left(5-x\right)\)
\(\Leftrightarrow\left(x-5\right)\left(x+5\right)-8\left(5-x\right)=0\)
\(\Leftrightarrow\left(x-5\right)\left(x+5\right)+8\left(x-5\right)=0\)
\(\Leftrightarrow\left(x-5\right)\left(x+13\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=5\\x=-13\end{matrix}\right.\)
b.\(\dfrac{x-2}{x+2}-\dfrac{2\left(x-11\right)}{x^2-4}=\dfrac{3}{x-2}\) ; \(ĐK:x\ne\pm2\)
\(\Leftrightarrow\dfrac{\left(x-2\right)\left(x-2\right)-2\left(x-11\right)}{\left(x-2\right)\left(x+2\right)}=\dfrac{3\left(x+2\right)}{\left(x-2\right)\left(x+2\right)}\)
\(\Leftrightarrow\left(x-2\right)^2-2\left(x-11\right)=3\left(x+2\right)\)
\(\Leftrightarrow x^2-4x+4-2x+22=3x+6\)
\(\Leftrightarrow x^2-9x+20=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=5\left(tm\right)\\x=4\left(tm\right)\end{matrix}\right.\)