Cho hình chữ nhật ABCD có tâm o cạnh AB = 2a và AD = a. Tính / AB + AD / và / BC - OD /
có ai giúp mình làm bài này với
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Lời giải:
1.
$\overrightarrow{2AO}-\overrightarrow{BC}=\overrightarrow{AC}-\overrightarrow{BC}=\overrightarrow{AB}$
Độ dài: $|\overrightarrow{AB}|=a$
2.
Trên tia đối của $AC$ lấy $T$ sao cho $TA=OC$
Trên tia đối của $BA$ lấy $K$ sao cho $BA=BK$
$\overrightarrow{OC}+2\overrightarrow{AB}=\overrightarrow{TA}+\overrightarrow{AB}+\overrightarrow{AB}$
$=\overrightarrow{TB}+\overrightarrow{AB}$
$=\overrightarrow{TB}+\overrightarrow{BK}=\overrightarrow{TK}$
Ta có:
$TC=3OC=\frac{3}{2}AC=\frac{3}{2}\sqrt{(2a)^2+a^2}=\frac{3\sqrt{5}}{2}a$
$CK=\sqrt{BC^2+BK^2}=\sqrt{(2a)^2+a^2}=\sqrt{5}a$
$\cos \widehat{TCK}=\cos 2\widehat{TCB}=2\cos^2 \widehat{TCB}-1$
$=2(\frac{CB}{AC})^2-1=\frac{3}{5}$
Áp dụng định lý cos:
$TK^2=TC^2+CK^2-2TC.CK\cos \widehat{TCK}$
$=\frac{45}{4}a^2+5a^2-9a^2=\frac{29}{4}a^2$
$\Rightarrow TK=\frac{\sqrt{29}}{2}a$
3. Trên tia đối tia $CD$ lấy $M$ sao cho $CM=CD$
$3\overrightarrow{AB}+2\overrightarrow{OD}=3\overrightarrow{DC}+2\overrightarrow{OD}=2\overrightarrow{OC}+\overrightarrow{DC}$
$=\overrightarrow{AC}+\overrightarrow{DC}=\overrightarrow{AC}+\overrightarrow{CM}=\overrightarrow{AM}$
$AM=\sqrt{AD^2+DM^2}=\sqrt{(2a)^2+(2a)^2}=2\sqrt{2}a$

a) \(AC = BD = \sqrt {A{B^2} + A{D^2}} \\= \sqrt {{{\left( {2a} \right)}^2} + {a^2}} = a\sqrt 5 \)
\(\cos \left( {\overrightarrow {AB} ,\overrightarrow {AO} } \right) = \cos \widehat {OAB} =\\ \cos \widehat {CAB} = \frac{{AB}}{{AC}} = \frac{{2a}}{{a\sqrt 5 }} = \frac{{2\sqrt 5 }}{5}\)
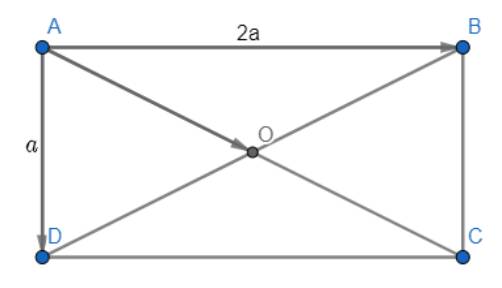
\(\begin{array}{l}\overrightarrow {AB} .\overrightarrow {AO} = \left| {\overrightarrow {AB} } \right|.\left| {\overrightarrow {AO} } \right|.\cos \left( {\overrightarrow {AB} ,\overrightarrow {AO} } \right) \\= AB.\frac{1}{2}AC.\cos \left( {\overrightarrow {AB} ,\overrightarrow {AO} } \right)\\ = 2a.\frac{1}{2}.a\sqrt 5 .\frac{{2\sqrt 5 }}{5} = 2{a^2}\end{array}\)
b) \(AB \bot AD \Rightarrow \left( {\overrightarrow {AB} ,\overrightarrow {AD} } \right) = 90^o \Rightarrow \cos \left( {\overrightarrow {AB} ,\overrightarrow {AD} } \right) =0 \Rightarrow \overrightarrow {AB} .\overrightarrow {AD} = 0\)

Đáp án C
Gọi O là trung điểm của SD. Ta có:
A D = D M = a 2 và A D = 2 a ⇒ A M ⊥ D M
Lại có D M ⊥ S A ⇒ D M ⊥ S A M ⇒ D M ⊥ S M
Vì tam giác SAD vuông tại A nên OS = OD = OA. Tương tự với tam giác SMD nên OS = OD = OM.
Vậy O là tâm mặt cầu ngoại tiếp hình chóp S.ADM. Khi đó R = S D 2 = S A 2 + D A 2 2 = a 6 2 .
\(\left|\overrightarrow{AB}+\overrightarrow{AD}\right|=a\sqrt{5}\)
\(\left|\overrightarrow{BC}-\overrightarrow{OD}\right|=\left|\overrightarrow{AD}+\overrightarrow{DO}\right|=AO=\dfrac{a\sqrt{5}}{2}\)