Giải phương trình:
3\(C^3_{n+1} \)- 3\(A^2_n\) = 52(\(n\) - 1)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
13 tháng 11 2021
Ta có:
\(2A_n^2=C_{n-1}^2+C_{n-1}^3\) \(\left(n\ge4\right)\)
\(\Rightarrow2\cdot\dfrac{n!}{\left(n-2\right)!}=\dfrac{\left(n-1\right)!}{2!\left(n-1-2\right)!}+\dfrac{\left(n-1\right)!}{3!\left(n-1-3\right)!}\)
\(\Rightarrow2\cdot n\left(n-1\right)=\dfrac{\left(n-1\right)\left(n-2\right)}{4}+\dfrac{\left(n-1\right)\left(n-2\right)\left(n-3\right)}{6}\)
\(\Rightarrow2n=\dfrac{n-2}{4}+\dfrac{\left(n-2\right)\left(n-3\right)}{6}\)
\(\Rightarrow n=14\) hoặc \(n=0\left(loại\right)\)
Với n=14 ta có khai triển:
\(\left(x^2-\dfrac{1}{x^2}\right)^{14}=\sum\limits^{14}_{k=0}\cdot C_{14}^k\cdot\left(x^2\right)^{14-k}\cdot\left(\dfrac{1}{x^2}\right)^k\)
\(=C_{14}^k\cdot x^{28-4k}\)
Số hạng không chứa x: \(\Rightarrow28-4k=0\Rightarrow k=7\)
Vậy số hạng không chứa x trong khai triển là:
\(C_{14}^7\cdot x^{28-4\cdot7}=C_{14}^7=3432\)
CY
0
NN
0
NV
Nguyễn Việt Lâm
Giáo viên
8 tháng 2 2020
Xét khai triển:
\(\left(3-x\right)^n=C_n^0.3^n+C_n^1.3^{n-1}.\left(-x\right)^1+...+C_n^n\left(-x\right)^n\)
Thế \(x=1\) vào ta được:
\(2^n=3^nC_n^0-3^{n-1}C_n^1+...+\left(-1\right)^nC_n^n\)
\(\Rightarrow2^n=2048=2^{11}\Rightarrow n=11\)
NV
Nguyễn Việt Lâm
Giáo viên
8 tháng 2 2020
\(\Leftrightarrow\left\{{}\begin{matrix}u_1-u_1q^2+u_1q^4=65\\u_1+u_1q^6=325\end{matrix}\right.\)
Chia vế cho vế ta được:
\(\frac{q^6+1}{q^4-q^2+1}=5\Leftrightarrow\frac{\left(q^2+1\right)\left(q^4-q^2+1\right)}{q^4-q^2+1}=5\)
\(\Leftrightarrow q^2=4\Rightarrow\left[{}\begin{matrix}q=2\\q=-2\end{matrix}\right.\)
\(\Rightarrow u_1=\frac{325}{q^6+1}=5\)
26 tháng 9 2020
Phạm Dương Ngọc Nhi thế thì bạn học pp này đi. Cái pp này giúp cm nhiều bài một cách dễ dàng
G
12 tháng 8 2023
ĐK của pt là \(n\ge2\)
\(\left(1+x\right)^n=C_n^0+x.C_n^1+x^2.C_n^2+x^3.C^3_n+x^4.C_n^4+...+x^n.C_n^n\)
\(\Rightarrow n\left(1+x\right)^{n-1}=C_n^1+2x.C_n^2+3x^2.C^3_n+4x^3.C_n^4...+n.x^{n-1}.C^n_n\) ( đạo hàm hai vế )
\(\Rightarrow n\left(n-1\right)\left(x+1\right)^{n-2}=2.C_n^2+2.3.x.C_n^3+3.4.x^2.C_n^4+...+\left(n-1\right)n.x^{n-2}.C_n^n\) ( đạo hàm hai vế )
Thay x=1 ta được: \(n\left(n-1\right).2^{n-2}=2.C_n^2+2.3.C^3_n+3.4.C_n^4+...+\left(n-1\right).n.C^n_n\)
\(\Leftrightarrow n\left(n-1\right).2^{n-2}=64n.\left(n-1\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}n\left(n-1\right)=0\\2^{n-2}=64\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}n=0;n=1\left(ktm\right)\\n=8\left(tm\right)\end{matrix}\right.\)
Vậy \(n=8\)
CM
2 tháng 7 2017
a) 3 x 2 − 7 x − 10 ⋅ 2 x 2 + ( 1 − 5 ) x + 5 − 3 = 0
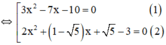
+ Giải (1):
3 x 2 – 7 x – 10 = 0
Có a = 3; b = -7; c = -10
⇒ a – b + c = 0
⇒ (1) có hai nghiệm x 1 = - 1 v à x 2 = - c / a = 10 / 3 .
+ Giải (2):
2 x 2 + ( 1 - √ 5 ) x + √ 5 - 3 = 0
Có a = 2; b = 1 - √5; c = √5 - 3
⇒ a + b + c = 0
⇒ (2) có hai nghiệm:
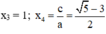
Vậy phương trình có tập nghiệm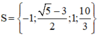 
b)
x 3 + 3 x 2 - 2 x - 6 = 0 ⇔ x 3 + 3 x 2 - ( 2 x + 6 ) = 0 ⇔ x 2 ( x + 3 ) - 2 ( x + 3 ) = 0 ⇔ x 2 - 2 ( x + 3 ) = 0
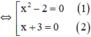
+ Giải (1): x 2 – 2 = 0 ⇔ x 2 = 2 ⇔ x = √2 hoặc x = -√2.
+ Giải (2): x + 3 = 0 ⇔ x = -3.
Vậy phương trình có tập nghiệm S = {-3; -√2; √2}
c)
x 2 − 1 ( 0 , 6 x + 1 ) = 0 , 6 x 2 + x ⇔ x 2 − 1 ( 0 , 6 x + 1 ) = x ⋅ ( 0 , 6 x + 1 ) ⇔ x 2 − 1 ( 0 , 6 x + 1 ) − x ( 0 , 6 x + 1 ) = 0 ⇔ ( 0 , 6 x + 1 ) x 2 − 1 − x = 0
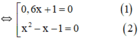
+ Giải (1): 0,6x + 1 = 0 ⇔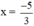 
+ Giải (2):
x 2 – x – 1 = 0
Có a = 1; b = -1; c = -1
⇒ Δ = ( - 1 ) 2 – 4 . 1 . ( - 1 ) = 5 > 0
⇒ (2) có hai nghiệm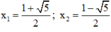 
Vậy phương trình có tập nghiệm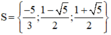 
d)
x 2 + 2 x − 5 2 = x 2 − x + 5 2 ⇔ x 2 + 2 x − 5 2 − x 2 − x + 5 2 = 0 ⇔ x 2 + 2 x − 5 − x 2 − x + 5 ⋅ x 2 + 2 x − 5 + x 2 − x + 5 = 0 ⇔ ( 3 x − 10 ) 2 x 2 + x = 0
⇔ (3x-10).x.(2x+1)=0
+ Giải (1): 3x – 10 = 0 ⇔ 
+ Giải (2):
\(n\ge2\)
\(\frac{3.\left(n+1\right)!}{3!.\left(n-2\right)!}-\frac{3.n!}{\left(n-2\right)!}=52\left(n-1\right)\)
\(\Leftrightarrow\frac{\left(n+1\right)n\left(n-1\right)}{2}-3n\left(n-1\right)=52\left(n-1\right)\)
\(\Leftrightarrow n\left(n+1\right)-6n=104\)
\(\Leftrightarrow n^2-5n-104=0\Rightarrow\left[{}\begin{matrix}n=13\\n=-8\left(l\right)\end{matrix}\right.\)