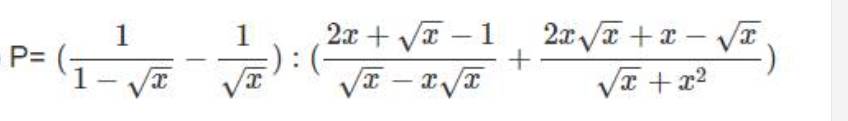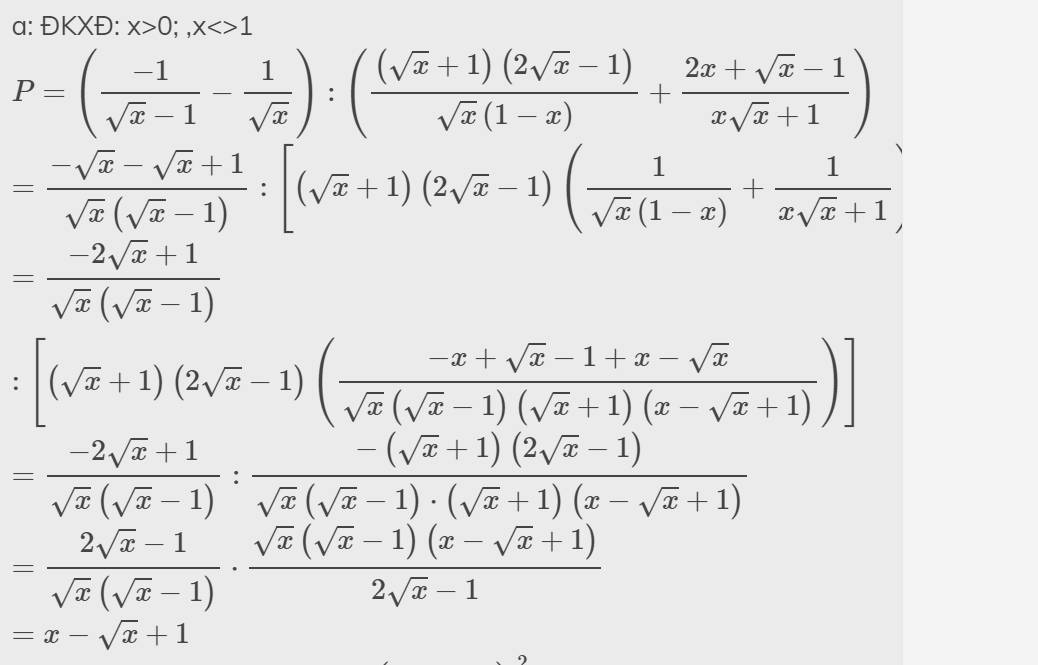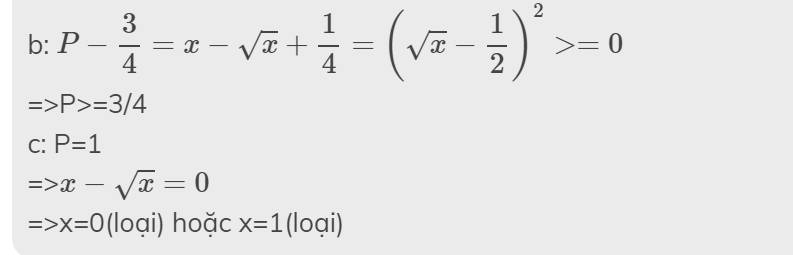Tìm max: \(\dfrac{1}{\sqrt{x}-1}-\dfrac{1}{\sqrt{x}+1}+\dfrac{2x^2+4}{1-x^3}\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
DT
0
AH
Akai Haruma
Giáo viên
4 tháng 7 2021
$A=2x-\sqrt{x}=2(x-\frac{1}{2}\sqrt{x}+\frac{1}{4^2})-\frac{1}{8}$
$=2(\sqrt{x}-\frac{1}{4})^2-\frac{1}{8}$
$\geq \frac{-1}{8}$
Vậy $A_{\min}=-\frac{1}{8}$. Giá trị này đạt tại $x=\frac{1}{16}$
AH
Akai Haruma
Giáo viên
4 tháng 7 2021
$B=x+\sqrt{x}$
Vì $x\geq 0$ nên $B\geq 0+\sqrt{0}=0$
Vậy $B_{\min}=0$. Giá trị này đạt tại $x=0$
28 tháng 8 2023
1: \(\Leftrightarrow\dfrac{3x-1}{x+2}=4\)
=>4x+8=3x-1
=>x=-9
2: \(\Leftrightarrow\dfrac{5x-7}{2x-1}=4\)
=>8x-4=5x-7
=>3x=-3
=>x=-1
3: ĐKXD: x>=0
\(PT\Leftrightarrow\left(\sqrt{x}-2\right)\left(\sqrt{x}+3\right)=\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)\)
=>\(x+\sqrt{x}-6=x-1\)
=>căn x=-1+6=5
=>x=25
4: ĐKXĐ: x>=0
PT =>\(\left(\sqrt{x}-3\right)\left(\sqrt{x}+1\right)=\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)\)
=>x-2*căn x-3=x-4
=>-2căn x-3=-4
=>2căn x+3=4
=>2căn x=1
=>căn x=1/2
=>x=1/4
DT
0
HP
27 tháng 8 2021
a, \(x+1\ge0\Leftrightarrow x\ge-1\)
b, \(1-2x\ge0\Leftrightarrow x\le\dfrac{1}{2}\)
c, \(\left\{{}\begin{matrix}x+1\ge0\\x-2\ge0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\ge-1\\x\ge2\end{matrix}\right.\Leftrightarrow x\ge2\)
HP
27 tháng 8 2021
d, \(\left\{{}\begin{matrix}2-3x\ge0\\1-2x\ge0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\le\dfrac{2}{3}\\x\le\dfrac{1}{2}\end{matrix}\right.\Leftrightarrow x\le\dfrac{1}{2}\)
e, \(\left\{{}\begin{matrix}\sqrt{3}-2x\ge0\\x-1\ne0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\le\dfrac{\sqrt{3}}{2}\\x\ne1\end{matrix}\right.\Leftrightarrow x\le\dfrac{\sqrt{3}}{2}\)
XO
27 tháng 12 2021
a) ĐKXĐ : \(3\le x\le7\)
Ta có \(A=1.\sqrt{x-3}+1.\sqrt{7-x}\)
\(\le\sqrt{\left(1+1\right)\left(x-3+7-x\right)}=\sqrt{8}\)(BĐT Bunyacovski)
Dấu "=" xảy ra <=> \(\dfrac{1}{\sqrt{x-3}}=\dfrac{1}{\sqrt{7-x}}\Leftrightarrow x=5\)
AD
15 tháng 8 2023
\(a,\dfrac{-5}{x+6}\ge0\\ mà\left(-5< 0\right)\\ \Rightarrow x+6< 0\\ \Rightarrow x< -6\\ b,\dfrac{2}{6-x}\ge0\\ mà\left(2>0\right)\\ \Rightarrow6-x>0\\ \Rightarrow x< 6\\ c,\dfrac{-x+3}{-6}\ge0\\ mà-6< 0\\ \Rightarrow-x+3< 0\\ \Rightarrow x>3\\\)
\(d,\dfrac{7x-1}{-9}\ge0\\mà-9< 0\\ \Rightarrow 7x-1\le0\\ \Rightarrow x\le\dfrac{1}{7}\\ e,\dfrac{x+2}{x^2+2x+1}\ge0\\ mà\left(x^2+2x+1\right)>0\forall x\\ \Rightarrow x+2\ge0\\ \Rightarrow x\ge-2\\ f,\dfrac{x-2}{x^2-2x+4}\ge0\\ mà\left(x^2-2x+4\right)>0\forall x\\ \Rightarrow x-2\ge0\\ \Rightarrow x\ge2\)
Chứng minh : \(x^2-2x+4>0\\ x^2-2x+1+3=\left(x-1\right)^2+3\ge3>0\)
15 tháng 8 2023
a: ĐKXĐ: \(\dfrac{-5}{x+6}>=0\)
=>x+6<0
=>x<-6
b: ĐKXĐ: (-2)/(6-x)>=0
=>6-x<0
=>x>6
c: ĐKXĐ: (-x+3)/(-6)>=0
=>-x+3<=0
=>-x<=-3
=>x>=3
d: ĐKXĐ: (7x-1)/-9>=0
=>7x-1<=0
=>x<=1/7
e: ĐKXĐ: (x+2)/(x^2+2x+1)>=0
=>x+2>=0
=>x>=-1
f: ĐKXĐ: (x-2)/(x^2-2x+4)>=0
=>x-2>=0
=>x>=2
25 tháng 9 2021
1) ĐKXĐ: \(x^2+2x-3\ge0\Leftrightarrow\left(x+1\right)^2\ge4\)
\(\Leftrightarrow\left[{}\begin{matrix}x+1\ge2\\x+1\le-2\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x\ge1\\x\le-3\end{matrix}\right.\)
2) ĐKXĐ: \(2x^2+5x+3\ge0\Leftrightarrow2\left(x+\dfrac{5}{4}\right)^2\ge\dfrac{1}{8}\Leftrightarrow\left(x+\dfrac{5}{4}\right)^2\ge\dfrac{1}{16}\)
\(\Leftrightarrow\left[{}\begin{matrix}x+\dfrac{5}{4}\ge\dfrac{1}{4}\\x+\dfrac{5}{4}\le-\dfrac{1}{4}\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x\ge-1\\x\le-\dfrac{3}{2}\end{matrix}\right.\)
3) ĐKXĐ: \(x-1>0\Leftrightarrow x>1\)
4) ĐKXĐ: \(x-3< 0\Leftrightarrow x< 3\)
5) ĐKXĐ: \(x+2< 0\Leftrightarrow x< -2\)
6) ĐKXĐ: \(2a-1>0\Leftrightarrow a>\dfrac{1}{2}\)