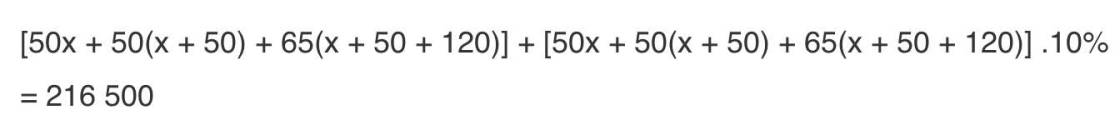Giải giúp mình pt lượng giác này với ạ: tan2x – tanx/cot3x = 2
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(tan2x=tanx\)
\(\Rightarrow2x=x+k\pi\)
\(\Rightarrow x=k\pi\)

\(tan\left(\dfrac{x}{2}\right)=\sqrt{3}\)
\(\Leftrightarrow\dfrac{x}{2}=\dfrac{\pi}{3}+k\pi\)
\(\Leftrightarrow x=\dfrac{2\pi}{3}+k2\pi\) (\(k\in Z\))

ĐKXĐ: ...
\(\Leftrightarrow\frac{sinx}{cosx}+\frac{sin2x}{cos2x}=sin3x.cosx\)
\(\Leftrightarrow\frac{sinx.cos2x+cosx.sin2x}{cosx.cos2x}=sin3x.cosx\)
\(\Leftrightarrow\frac{sin3x}{cosx.cos2x}=sin3x.cosx\)
\(\Leftrightarrow\left[{}\begin{matrix}sin3x=0\Rightarrow x=\frac{k\pi}{3}\\\frac{1}{cosx.cos2x}=cosx\left(1\right)\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow cos^2x.cos2x=1\)
\(\Leftrightarrow\left(\frac{1+cos2x}{2}\right)cos2x=1\)
\(\Leftrightarrow cos^22x+cos2x-2=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos2x=1\\cos2x=2\left(l\right)\end{matrix}\right.\) \(\Rightarrow x=k\pi\)


c/
\(\Leftrightarrow\sqrt{3}tan\left(\frac{\pi}{9}-2x\right)=-3\)
\(\Leftrightarrow tan\left(\frac{\pi}{9}-2x\right)=-\sqrt{3}\)
\(\Rightarrow\frac{\pi}{9}-2x=-\frac{\pi}{3}+k\pi\)
\(\Rightarrow x=\frac{2\pi}{9}+\frac{k\pi}{2}\)
d/
\(\Leftrightarrow\left[{}\begin{matrix}tanx=5\\tan2x=tan4\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=arctan\left(5\right)+k\pi\\2x=4+k\pi\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=arctan\left(5\right)+k\pi\\x=2+\frac{k\pi}{2}\end{matrix}\right.\)
a/
ĐKXĐ: ...
\(\Leftrightarrow tanx-8\sqrt{3}=3tanx-6\sqrt{3}\)
\(\Leftrightarrow2tanx=-2\sqrt{3}\)
\(\Rightarrow tanx=-\sqrt{3}\Rightarrow x=-\frac{\pi}{3}+k\pi\)
b/
\(\Leftrightarrow tan2x=-cot\left(\frac{5\pi}{8}\right)\)
\(\Leftrightarrow tan2x=tan\left(\frac{\pi}{2}+\frac{5\pi}{8}\right)\)
\(\Leftrightarrow tan2x=tan\left(\frac{9\pi}{8}\right)\)
\(\Rightarrow2x=\frac{9\pi}{8}+k\pi\Rightarrow x=\frac{9\pi}{16}+\frac{k\pi}{2}\)

\(tanx=-tan\dfrac{\pi}{5}\)
\(\Leftrightarrow tanx=tan\left(-\dfrac{\pi}{5}\right)\)
\(\Leftrightarrow x=-\dfrac{\pi}{5}+k\pi\)
Mình quên mất, nó nằm trong khoảng (π/2; π) nha, mình xin lỗi

=>(50x+50x+250+65x+11050)*1,1=216500
=>165x+11300=196818,1818
=>165x=185518,1818
=>\(x\simeq124.353\)