giải phương trình |x+10|5 +|x-10|5=200000
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
ND
0
KH
28 tháng 2 2018
a, \(\frac{x+9}{10}+\frac{x+10}{9}=\frac{9}{x+10}+\frac{10}{x+9}\)(1)
ĐKXĐ: \(\hept{\begin{cases}x+9\ne0\\x+10\ne0\end{cases}\Leftrightarrow\hept{\begin{cases}x\ne-9\\x\ne-10\end{cases}}}\)
(1)\(\Leftrightarrow\frac{9.\left(x+9\right)}{90}+\frac{10.\left(x+10\right)}{90}=\frac{9.\left(x+9\right)}{\left(x+9\right)\left(x+10\right)}+\frac{10.\left(x+10\right)}{\left(x+9\right)\left(x+10\right)}\)
\(\Leftrightarrow9.\left(x+9\right)+10.\left(x+10\right)=9.\left(x+9\right)+10.\left(x+10\right)\)
\(\Leftrightarrow9x+81+10x+100=9x+81+10x+100\)
\(\Leftrightarrow9x+10x-9x-10x=81+100-81-100\)
\(\Leftrightarrow0x=0\)
\(\Rightarrow x\in R\)trừ -9 và -10
ML
2 tháng 8 2016
\(pt\Leftrightarrow\left[\left(x-4\right)\left(x-10\right)\right]\left[\left(x-5\right)\left(x-8\right)\right]=72x^2\)
\(\Leftrightarrow\left(x^2+40-14x\right)\left(x^2+40-13x\right)=72x^2\)
\(x=0\) không phải là nghiệm của phương trình trên
Xét \(x\ne0\)
\(pt\Leftrightarrow\frac{x^2+40-14x}{x}.\frac{x^2+40-13x}{x}=72\)
\(\Leftrightarrow\left(x+\frac{40}{x}-14\right)\left(x+\frac{40}{x}-13\right)=72\)
Đặt \(x+\frac{40}{x}-14=a\)
\(pt\rightarrow a\left(a+1\right)=72\Leftrightarrow a^2+a-72=0\Leftrightarrow\orbr{\begin{cases}a=8\\a=-9\end{cases}}\)
TH1: a = 8 \(\Rightarrow x+\frac{40}{x}-14=8\Leftrightarrow\frac{x^2-22x+40}{x}=0\Leftrightarrow\orbr{\begin{cases}x=2\\x=20\end{cases}}\)
TH2: a = -9 \(\Rightarrow x+\frac{40}{x}-14=-9\Leftrightarrow\frac{x^2-5x+40}{x}=0\text{ }\left(\text{vô nghiệm }\right)\)
22 tháng 7 2021
1) Ta có: \(4x+8=3x-1\)
\(\Leftrightarrow4x-3x=-1-8\)
\(\Leftrightarrow x=-9\)
2) Ta có: \(10-5\left(x+3\right)>3\left(x-1\right)\)
\(\Leftrightarrow10-5x-15-3x+3>0\)
\(\Leftrightarrow-8x>2\)
hay \(x< \dfrac{-1}{4}\)
15 tháng 12 2023
(m+5)x+2m-10=0
=>(m+5)x=-2m+10
TH1: m<>-5
=>Phương trình có nghiệm duy nhất là \(x=\dfrac{-2m+10}{m+5}\)
TH2: m=-5
Phương trình sẽ trở thành:
\(0x=-2\cdot\left(-5\right)+10=10+10=20\)
=>\(x\in\varnothing\)
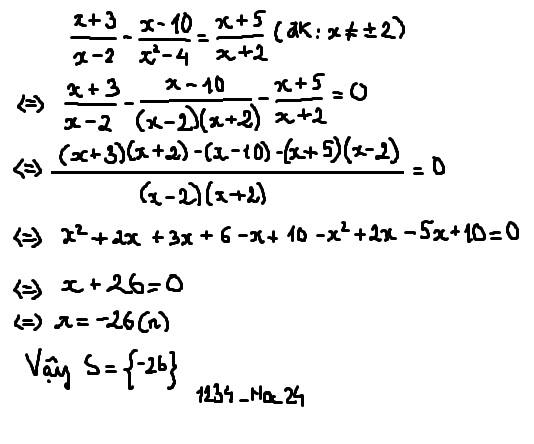
Làm nhầm làm lại nhé
Ta có:
|x + 10|5 + |x - 10|5
= |x + 10|5 + |10 - x|5
= |x5 + 50x4 + 1000x3 + 10000x2 + 50000x + 100000| + |-x5 + 50x4 - 1000x3 + 10000x2 - 50000x + 100000|
\(\ge\)|100x4 + 20000x2 + 200000|
\(\ge\)|200000| = 200000
Dấu = xảy ra khi x = 0
Ta có:
|x + 10|5 + |x - 10|5
= |x + 10|5 + |10 - x|5
= |x5 + 50x4 + 1000x3 + 10000x2 + 50000x + 1000000| + |-x5 + 50x4 - 1000x3 + 10000x2 - 50000x + 1000000|
\(\ge\)|100x4 + 20000x2 + 2000000|
\(\ge\)|2000000| = 2000000
Dấu = xảy ra khi x = 0