4x-2 + 4x+1 = 1040
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


4x + 3 + 4x = 1040
4x . 43 + 4x . 1 = 1040
4x ( 43 + 1 ) = 1040
4x . 65 = 1040
4x = 1040 : 65 = 16 = 42
Vậy x = 2
\(4^{x+3}+4^x=1040\)
\(\Leftrightarrow4^x\left(1+4^3\right)=1040\)
\(\Leftrightarrow4^x.65=1040\)
\(\Leftrightarrow4^x=16\)
\(\Leftrightarrow x=2\)


1) (4x−7)2−5×|7−4x|=0
Có (4x-7)2 \(\ge0\) với mọi x
|7−4x| \(\ge0\) với mọi x
<=> 5|7−4x| \(\ge0\) với mọi x
Để (4x−7)2−5×|7−4x|=0 thì \(\left\{{}\begin{matrix}\left(4x-7\right)^2=0\\5|7-4x|=0\end{matrix}\right.\)<=>\(\left\{{}\begin{matrix}4x-7=0\\7-4x=0\end{matrix}\right.\)<=>\(\left\{{}\begin{matrix}4x=7\\4x=7\end{matrix}\right.\)<=>\(x=\dfrac{7}{4}\)
Vậy \(x=\dfrac{7}{4}\)
2) \(4^{x-2}+4^{x+1}=1040\)
<=> \(4^{x+1}.4^{-3}+4^{x+1}=1040\)
<=> \(4^{x+1}\left(4^{-3}+1\right)=1040\)
<=> \(4^{x+1}.\dfrac{65}{64}=1040\)
<=> \(4^{x+1}=1024=4^5\)
=> x+1=5 <=> x=4
Vậy x=4

Ta có: \(\sqrt{4x^2-4x+1}-\sqrt{4x^2+4x+1}\)
\(=\sqrt{\left(2x-1\right)^2}-\sqrt{\left(2x+1\right)^2}\)
\(=\left|2x-1\right|-\left|2x+1\right|\)

\(4x^2-4x+1=\left(2x\right)^2-2.2x.1+1^2=\left(2x-1\right)^2\\ ---\\ 4x^2-4x-3\\ =4x^2-4x+1-4\\ =\left(2x-1\right)^2-2^2=\left(2x-1-2\right)\left(2x-1+2\right)\\ =\left(2x-3\right)\left(2x+1\right)\)
1: =(2x)^2-2*2x*1+1^2
=(2x-1)^2
2: =4x^2-6x+2x-3
=2x(2x-3)+(2x-3)
=(2x-3)(2x+1)

1) ( 4x + 1 )2 + ( 4x - 1 )2 - 2( 4x + 1 ).( 4x - 1 )
= ( 4x + 1 - 4x - 1 )2
= 22
= 4
2) 4x2 - 9 + ( 2x + 3 )
= ( 2x )2 - 32 + ( 2x + 3 )
= ( 2x + 3 ).( 2x - 3 ) + ( 2x + 3 )
= ( 2x + 3 ). ( 2x - 3 + 1 )
= ( 2x + 3 ) .( 2x - 2 )
= 2.( 2x + 3 ) .( x - 1 )
1, (4x+1)^2 + (4x-1)^2 - 2(4x+1)(4x-1)
=[(4x+1)-(4x-1)]^2
=(4x+1-4x+1)^2
=2^2
=4
2, 4x^2 - 9 +(2x+3)
=(4x^2 - 9)+(2x+3)
=(2x+3)(2x-3)+(2x+3)
=(2x+3)(2x-3+1)
=(2x+3)(2x-2)
=2(x-1)(2x+3)
=.= hok tốt!!


\(y=\sqrt{\left(2x-1\right)^2}+\sqrt{\left(x-2\right)^2}=\left|2x-1\right|+\left|x-2\right|\)
\(y=\left[{}\begin{matrix}3x-3\left(\text{với }x\ge2\right)\\3-3x\left(\text{với }x\le\dfrac{1}{2}\right)\\x+1\left(\text{với }\dfrac{1}{2}\le x\le2\right)\end{matrix}\right.\)
Từ đó ta có đồ thị hàm số như sau:
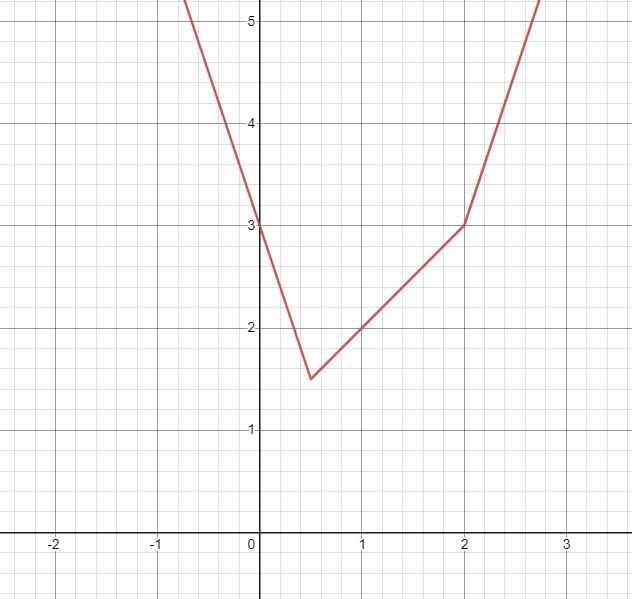
Từ đồ thị ta thấy phương trình \(\sqrt{4x^2-4x+1}+\sqrt{x^2-4x+4}=m\):
- Có đúng 1 nghiệm khi \(m=\dfrac{3}{2}\)
- Có 2 nghiệm phân biệt khi \(m>\dfrac{3}{2}\)
- Vô nghiệm khi \(m< \dfrac{3}{2}\)
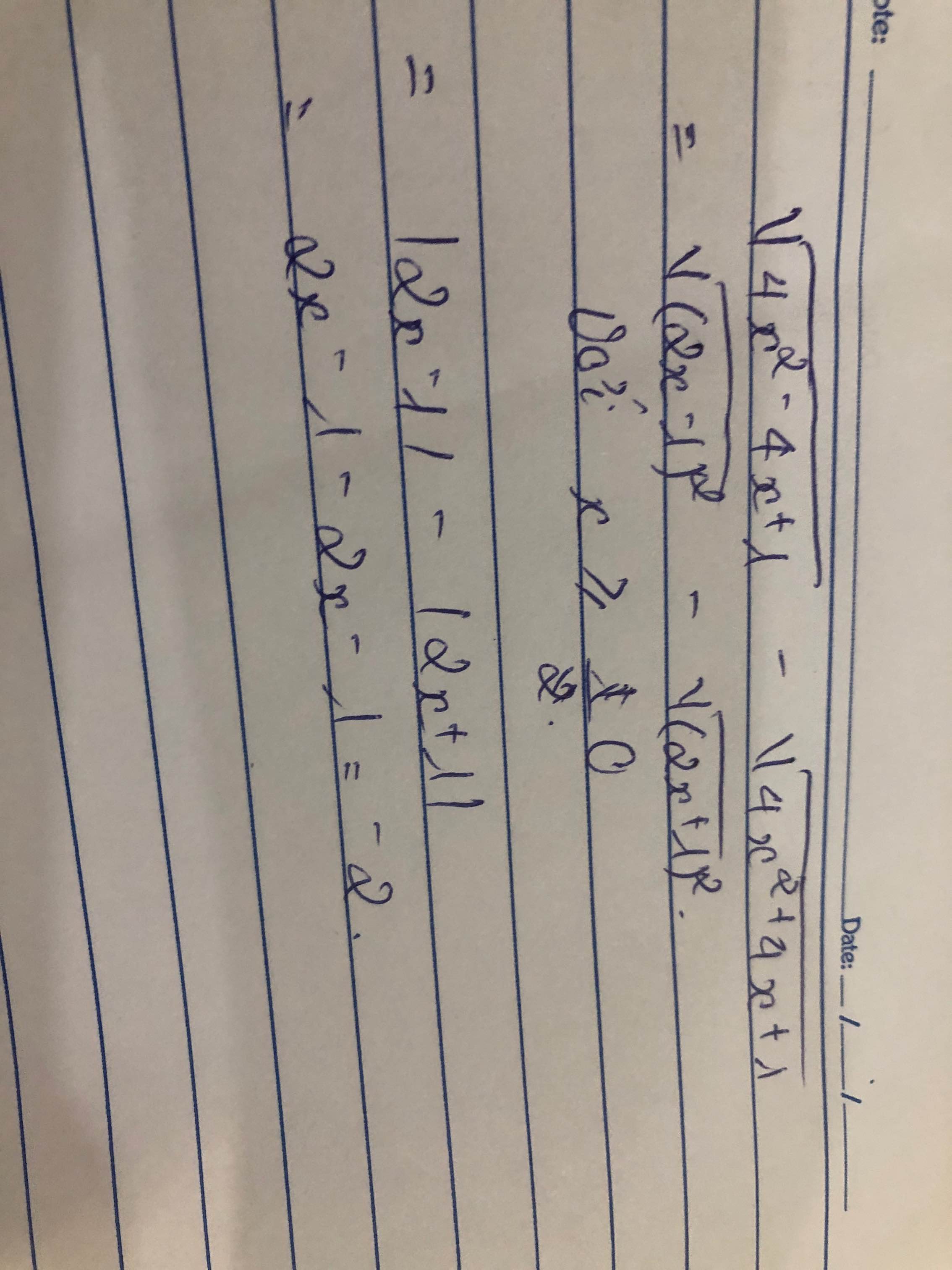
4x-2 +4x+1 =1040
4x:42+4x.4 =1040
4x:2+4x.4 =1040
4x.1/2+4x.4=1040
4x.(1/2+4) =1040
4x.9/2 =1040
4x =1040:9/2
4x =2080/9