Giúp mình bài này với được không ạ😓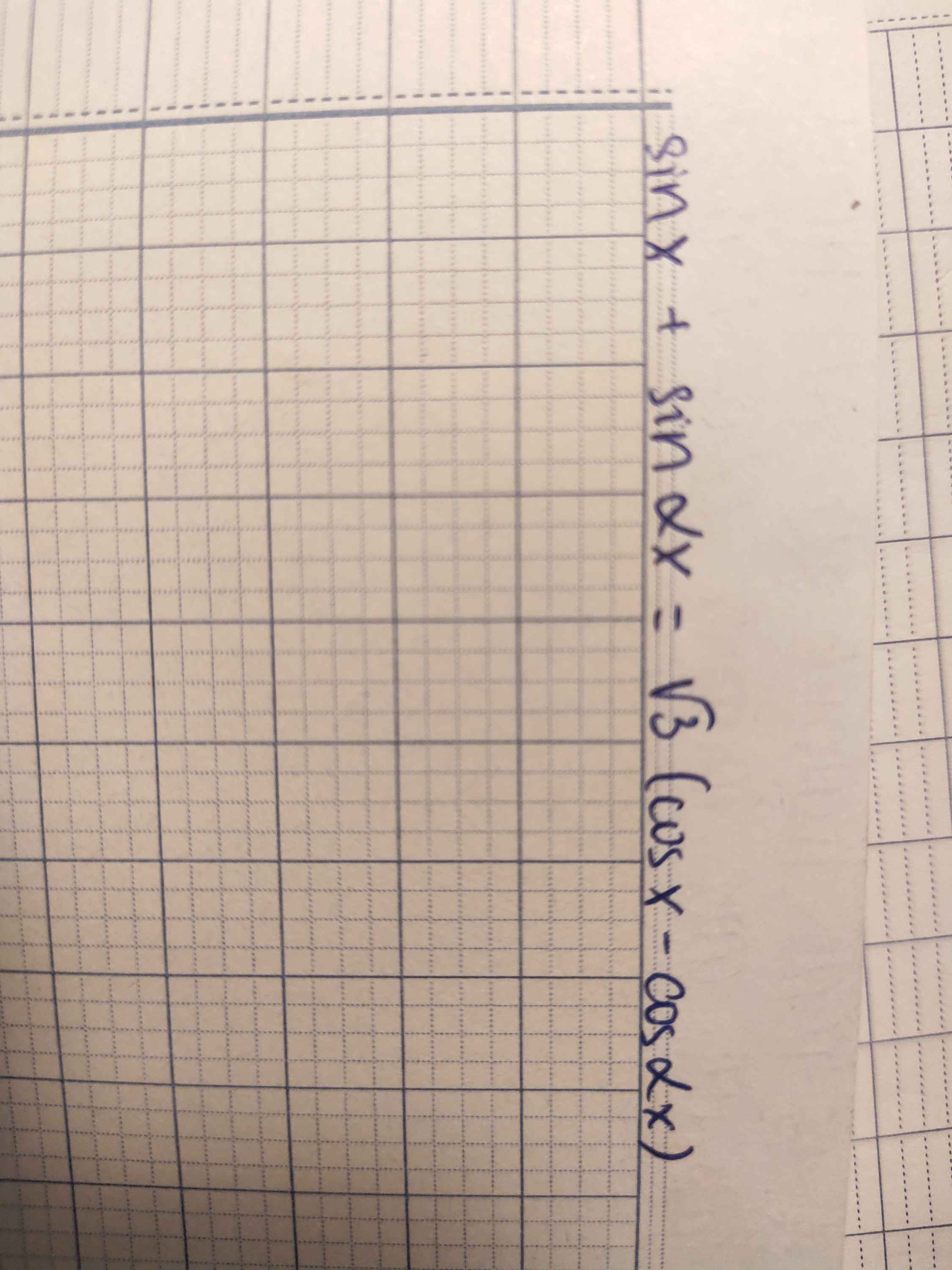
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NV
Nguyễn Việt Lâm
Giáo viên
2 tháng 1 2022
Phương trình hoành độ giao điểm (d) và (P):
\(x^2-2x-3=ax-a-3\)
\(\Leftrightarrow x^2-\left(a+2\right)x+a=0\)
\(\Delta=\left(a+2\right)^2-4a=a^2+4>0;\forall a\Rightarrow\) (d) luôn cắt (P) tại 2 điểm pb
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_A+x_B=a+2\\x_Ax_B=a\end{matrix}\right.\)
Mặt khác do A, B thuộc (d) nên: \(\left\{{}\begin{matrix}y_A=ax_A-a-3\\y_B=ax_B-a-3\end{matrix}\right.\)
\(y_A+y_B=0\)
\(\Leftrightarrow a\left(x_A+x_B\right)-2a-6=0\)
\(\Leftrightarrow a\left(a+2\right)-2a-6=0\)
\(\Leftrightarrow a^2-6=0\)
\(\Leftrightarrow a=\pm\sqrt{6}\)
NV
Nguyễn Việt Lâm
Giáo viên
1 tháng 3 2023
27.
Bán kính mặt cầu ngoại tiếp tứ diện vuông được tính bằng:
\(R=\sqrt{\dfrac{OA^2+OB^2+OC^2}{4}}=\sqrt{\dfrac{1^2+2^2+3^2}{4}}=\dfrac{\sqrt{14}}{2}\)
28.
Từ giả thiết suy ra \(A\left(2;2;2\right)\)
Gọi điểm thuộc mặt Oxz có tọa độ dạng \(D\left(x;0;z\right)\)
\(\Rightarrow\overrightarrow{AD}=\left(x-2;-2;z-2\right)\)
\(\overrightarrow{BD}=\left(x+2;-2;z\right)\) ; \(\overrightarrow{CD}=\left(x-4;-1;z+1\right)\)
D cách đều A, B, C \(\Rightarrow\left\{{}\begin{matrix}AD=BD\\AD=CD\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}\left(x-2\right)^2+4+\left(z-2\right)^2=\left(x+2\right)^2+4+z^2\\\left(x-2\right)^2+4+\left(z-2\right)^2=\left(x-4\right)^2+1+\left(z+1\right)^2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}2x+z=1\\2x-3z=3\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}z=-\dfrac{1}{2}\\x=\dfrac{3}{4}\end{matrix}\right.\)
\(\Rightarrow P\left(\dfrac{3}{4};0;-\dfrac{1}{2}\right)\)
NV
Nguyễn Việt Lâm
Giáo viên
1 tháng 3 2023
29.
Do tâm I mặt cầu thuộc Oz nên tọa độ có dạng: \(I\left(0;0;z\right)\)
\(\Rightarrow\left\{{}\begin{matrix}\overrightarrow{AI}=\left(-3;1;z-2\right)\\\overrightarrow{BI}=\left(-1;-1;z+2\right)\end{matrix}\right.\)
Mặt cầu qua A, B nên \(AI=BI\)
\(\Leftrightarrow3^2+1^2+\left(z-2\right)^2=1^2+1^2+\left(z+2\right)^2\)
\(\Leftrightarrow8z=8\Rightarrow z=1\)
\(\Rightarrow I\left(0;0;1\right)\Rightarrow R=IB=\sqrt{1^2+1^1+3^2}=\sqrt{11}\)
Phương trình mặt cầu:
\(x^2+y^2+\left(z-1\right)^2=11\)
NV
Nguyễn Việt Lâm
Giáo viên
21 tháng 12 2022
42.
ĐKXĐ: \(x>0\)
\(log_3^2x-5log_3x+6\le0\)
\(\Leftrightarrow\left(log_3x-2\right)\left(log_3x-3\right)\le0\)
\(\Rightarrow2\le log_3x\le3\)
\(\Rightarrow9\le x\le27\)
\(\Rightarrow2a-b=9.2-27=\)
43.
\(r=\dfrac{1}{2};h=1\)
\(\Rightarrow V=\pi r^2h=\dfrac{\pi}{4}\)
44.
ĐKXĐ: \(a>0\)
\(log_2a+log_23=log_2\left(2a+2\right)\)
\(\Leftrightarrow log_2\left(3a\right)=log_2\left(2a+2\right)\)
\(\Rightarrow3a=2a+2\)
\(\Rightarrow a=2\)
NV
Nguyễn Việt Lâm
Giáo viên
21 tháng 12 2022
45.
\(V=\dfrac{1}{3}.6a.20a^2=40a^3\)
46.
Pt hoành độ giao điểm:
\(-x^2+2=x^3+2\Leftrightarrow x^3+x^2=0\)
\(\Leftrightarrow x^2\left(x+1\right)=0\Rightarrow\left[{}\begin{matrix}x=0\\x=-1\end{matrix}\right.\)
\(\Rightarrow\) Hai đồ thị có 2 giao điểm
47.
\(y'=x^2-5x+6=0\Rightarrow\left[{}\begin{matrix}x=2\\x=3\end{matrix}\right.\)
\(f\left(1\right)=\dfrac{29}{6}\) ; \(f\left(2\right)=\dfrac{17}{3}\) ; \(f\left(3\right)=\dfrac{11}{2}\)
\(\Rightarrow\) Hàm đạt min tại \(x=1\) và đạt max tại \(x=2\)
\(\Rightarrow x_1+x_2=3\)
48.
\(y'=-4x^3=0\Rightarrow x=0\)
Do \(a=-1< 0\Rightarrow\)hàm đồng biến trên \(\left(-\infty;0\right)\)
49.
\(y'=3ax^2+2bx+c\Rightarrow\left\{{}\begin{matrix}c=0\\12a+4b+c=0\end{matrix}\right.\)
\(x=0;y=d\Rightarrow d=2\)
\(x=2;y=-2\Rightarrow8a+4b+2c+d=-2\)
\(\Rightarrow8a+4b+2=-2\Rightarrow\left\{{}\begin{matrix}12a+4b=0\\8a+4b=-4\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}a=1\\b=-3\end{matrix}\right.\)
\(\Rightarrow y=x^3-3x^2+2\)
\(\Rightarrow y\left(-2\right)=-18\)
DD
Đoàn Đức Hà
Giáo viên
26 tháng 12 2022
Câu 3:
Phương trình hoành độ giao điểm: \(-x=x^3\Leftrightarrow x^3+x=0\Leftrightarrow x\left(x^2+1\right)=0\Leftrightarrow x=0\).
Phương trình có \(1\) nghiệm do đó chọn C.
DD
Đoàn Đức Hà
Giáo viên
26 tháng 12 2022
Câu 4:
\(2^{2x^2-7x+5}=32\)
\(\Leftrightarrow2^{2x^2-7x+5}=2^5\)
\(\Leftrightarrow2x^2-7x+5=5\)
\(\Leftrightarrow2x^2-7x=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=\dfrac{7}{2}\end{matrix}\right.\)
Suy ra \(x_1=0,x_2=\dfrac{7}{2}\).
\(x_1+4x_2=14\).
Chọn A.
NV
Nguyễn Việt Lâm
Giáo viên
20 tháng 12 2022
24.
\(log\left(a^3b^2\right)=loga^3+logb^2=3loga+2logb=3x+2y\)
25.
\(f'\left(x\right)=0\) có 2 nghiệm bội lẻ \(x=\left\{-\dfrac{3}{2};1\right\}\) nên hàm có 2 cực trị
26.
\(\lim\limits_{x\rightarrow\infty}\dfrac{x^2+3x-4}{x^2-16}=\lim\limits_{x\rightarrow\pm\infty}\dfrac{1+\dfrac{3}{x}-\dfrac{4}{x^2}}{1-\dfrac{16}{x^2}}=1\)
\(\Rightarrow y=1\) là TCN của đồ thị hàm số
\(\lim\limits_{x\rightarrow-4}\dfrac{x^2+3x-4}{x^2-16}=\lim\limits_{x\rightarrow-4}\dfrac{\left(x-1\right)\left(x+4\right)}{\left(x-4\right)\left(x+4\right)}=\lim\limits_{x\rightarrow-4}\dfrac{x-1}{x-4}=\dfrac{5}{8}\) hữu hạn
\(\Rightarrow x=-4\) không phải tiệm cận đứng
\(\lim\limits_{x\rightarrow4^+}\dfrac{x^2+3x-4}{x^2-16}=+\infty\Rightarrow x=4\) là 1 TCĐ
Vậy đồ thị hàm số có 2 tiệm cận
NV
Nguyễn Việt Lâm
Giáo viên
20 tháng 12 2022
27.
\(y'=x^2-2x+2\)
\(y'\left(1\right)=1\)
\(y\left(1\right)=\dfrac{7}{3}\)
Phương trình tiếp tuyến có dạng:
\(y=1\left(x-1\right)+\dfrac{7}{3}\Leftrightarrow y=x+\dfrac{4}{3}\)
28.
ĐKXĐ: \(x>0\)
\(\log x\le1\)
\(\Rightarrow x\le10\)
Kết hợp ĐKXĐ ta được \(x\in(0;10]\)
29.
Gọi H là trung điểm AB \(\Rightarrow SH\perp\left(ABCD\right)\)
Do SAB vuông cân tại S \(\Rightarrow SH=\dfrac{1}{2}AB=\dfrac{a}{2}\)
\(\Rightarrow V=\dfrac{1}{3}SH.a^2=\dfrac{a^3}{6}\)
21 tháng 1 2022
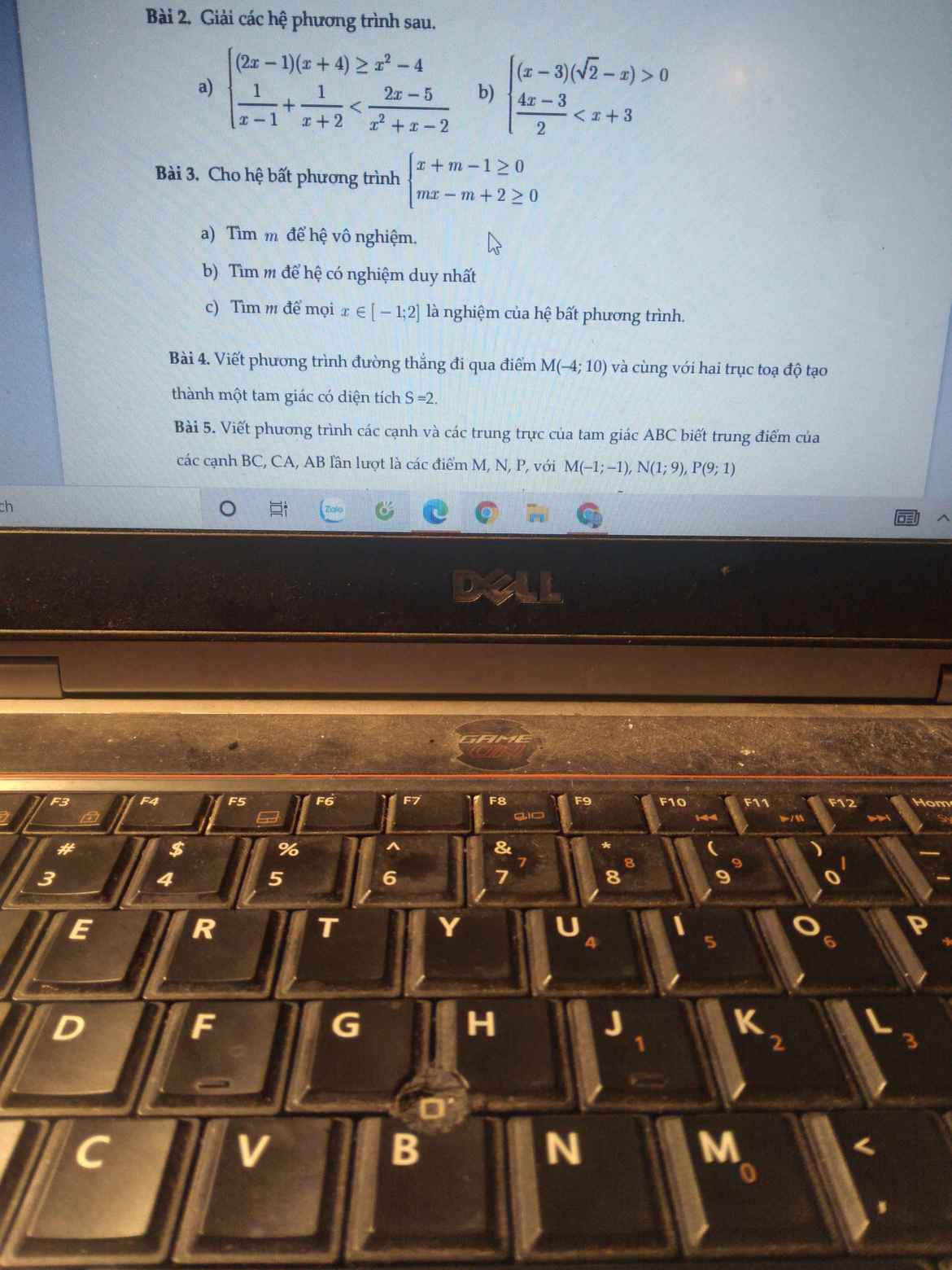
C3: Hệ bpt trở thành: \(\left\{{}\begin{matrix}x\ge1-m\\mx\ge2-m\end{matrix}\right.\)
a, Để hệ phương trình vô nghiệm thì \(m=0\)
b, Để hệ có nghiệm duy nhất thì \(\left\{{}\begin{matrix}m\ne0\\\dfrac{m-2}{m}=1-m\end{matrix}\right.\)
\(\Leftrightarrow\) \(\left\{{}\begin{matrix}m\ne0\\m=\pm\sqrt{2}\end{matrix}\right.\) \(\Leftrightarrow\) \(m=\pm\sqrt{2}\)
c, \(x\in\left[-1;2\right]\) \(\Leftrightarrow\) \(-1\le x\le2\)
Để mọi \(x\in\left[-1;2\right]\) là nghiệm của hệ bpt trên thì
\(\left\{{}\begin{matrix}-1\le1-m\le2\\-1\le\dfrac{2-m}{m}\le2\end{matrix}\right.\) với \(m\ne0\)
\(\Leftrightarrow\) \(\left\{{}\begin{matrix}2\ge m\ge-1\\m\ge\dfrac{2}{3}\end{matrix}\right.\) \(\left(m\ne0\right)\)
\(\Leftrightarrow\) \(2\ge m\ge\dfrac{2}{3}\)
Vậy \(m\in\left[\dfrac{2}{3};2\right]\) thì mọi \(x\in\left[-1;2\right]\) là nghiệm của hệ bpt
Chúc bn học tốt!
\(\Leftrightarrow sinx+sinax=\sqrt{3}cosx-\sqrt{3}cosax\)
\(\Leftrightarrow sinax+\sqrt{3}cosax=\sqrt{3}cosx-sinx\)
\(\Leftrightarrow\dfrac{\sqrt{3}}{2}cosax+\dfrac{1}{2}sinax=\dfrac{\sqrt{3}}{2}cosx-\dfrac{1}{2}sinx\)
\(\Leftrightarrow cos\left(ax-\dfrac{\pi}{6}\right)=cos\left(x+\dfrac{\pi}{6}\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}ax-\dfrac{\pi}{6}=x+\dfrac{\pi}{6}+k2\pi\\ax-\dfrac{\pi}{6}=-x-\dfrac{\pi}{6}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\left(a-1\right)x=\dfrac{\pi}{3}+k2\pi\\\left(a+1\right)x=k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{3\left(a-1\right)}+\dfrac{k2\pi}{a-1}\left(a\ne1\right)\\x=\dfrac{k2\pi}{a+1}\left(a\ne-1\right)\end{matrix}\right.\)