Cho các số thực dương \(a;b;c\) và thỏa mãn: \(a+b+c=1\). Chứng minh rằng :
\(\dfrac{a}{a+2.\sqrt{a+bc}}+\dfrac{b}{b+2.\sqrt{b+ac}}+\dfrac{c}{c+2.\sqrt{c+ab}}\le\dfrac{3}{5}\)
P/s: Em nhờ quý thầy cô và các bạn hỗ trợ và giúp đỡ em với ạ!
Em cám ơn nhiều lắm ạ!

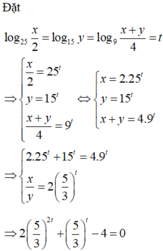
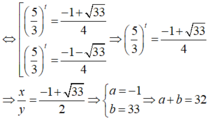
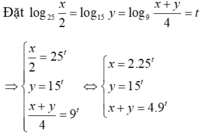
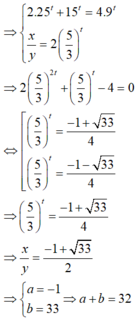
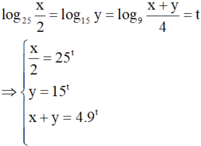
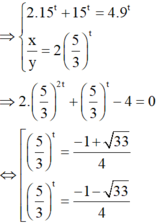
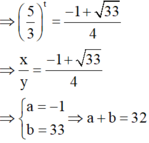
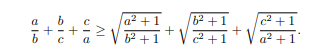
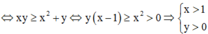
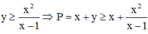
\(\dfrac{a}{a+2\sqrt{\left(a+bc\right)}}=\dfrac{a}{a+2\sqrt{a\left(a+b+c\right)+bc}}=\dfrac{a}{a+2\sqrt{\left(a+b\right)\left(a+c\right)}}\)
\(=\dfrac{a}{a+\dfrac{\sqrt{\left(a+b\right)\left(a+c\right)}}{2}+\dfrac{\sqrt{\left(a+b\right)\left(a+c\right)}}{2}+\dfrac{\sqrt{\left(a+b\right)\left(a+c\right)}}{2}+\dfrac{\sqrt{\left(a+b\right)\left(a+c\right)}}{2}}\)
\(\le\dfrac{a}{5^2}\left(\dfrac{1}{a}+\dfrac{1}{\dfrac{\sqrt{\left(a+b\right)\left(a+c\right)}}{2}}+\dfrac{1}{\dfrac{\sqrt{\left(a+b\right)\left(a+c\right)}}{2}}+\dfrac{1}{\dfrac{\sqrt{\left(a+b\right)\left(a+c\right)}}{2}}+\dfrac{1}{\dfrac{\sqrt{\left(a+b\right)\left(a+c\right)}}{2}}\right)\)
\(=\dfrac{a}{25}\left(\dfrac{1}{a}+\dfrac{8}{\sqrt{\left(a+b\right)\left(a+c\right)}}\right)=\dfrac{1}{25}+\dfrac{8}{25}.\dfrac{a}{\sqrt{\left(a+b\right)\left(a+c\right)}}\)
\(\le\dfrac{1}{25}+\dfrac{4}{25}\left(\dfrac{a}{a+b}+\dfrac{a}{a+c}\right)\)
Tương tự:
\(\dfrac{b}{b+2\sqrt{b+ac}}\le\dfrac{1}{25}+\dfrac{4}{25}\left(\dfrac{b}{a+b}+\dfrac{b}{b+c}\right)\)
\(\dfrac{c}{c+2\sqrt{c+ab}}\le\dfrac{1}{25}+\dfrac{4}{25}\left(\dfrac{c}{a+c}+\dfrac{c}{b+c}\right)\)
Cộng vế:
\(P\le\dfrac{3}{25}+\dfrac{4}{25}\left(\dfrac{a+b}{a+b}+\dfrac{b+c}{b+c}+\dfrac{c+a}{c+a}\right)=\dfrac{15}{25}=\dfrac{3}{5}\)
Dấu "=" xảy ra khi \(a=b=c=\dfrac{1}{3}\)