Question

[Phát]

1. Cho \(A=-\dfrac{3}{4}x^2yz;B=\dfrac{1}{3}xy^2;C=-\dfrac{8}{7}xy^2\)

Tính \(A.\left(B+C\right)\) ( Các bạn tính cách này cũng được miễn đúng được rồi )

2. Viết đơn thức \(3x^3y^2\) thành:

a) Tổng của 2 đơn thức \(A+B\), trong đó \(A=\dfrac{3}{5}x^3y^2\)

b) Hiệu của 2 đơn thức \(C-D\), trong đó \(D=-\dfrac{1}{4}x^3y^2\)

c) Tích của 2 đơn thức E và F với \(E=-\dfrac{5}{7}x^2y\)

3. Cho đơn thức \(A=5x^{n+2}y^{m-3}\)

Viết đơn thức A thành tích của 2 đơn thức mà trong 2 đơn thức ấy có một đơn thức là \(-\dfrac{3}{2}x^ny^3\)

Answer 1

[Lê Thị Ngọc Duyên]

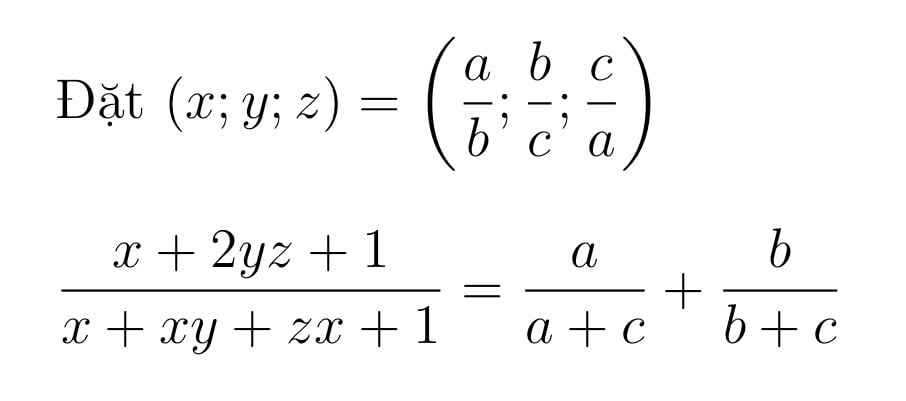
1.\(A=-\dfrac{3}{4}x^2yz;B=\dfrac{1}{3}xy^2;C=-\dfrac{8}{7}xy^2\)

\(A.\left(B+C\right)=-\dfrac{3}{4}x^2yz\left[\dfrac{1}{3}xy^2+\left(-\dfrac{8}{7}xy^2\right)\right]\)

\(=-\dfrac{3}{4}x^2yz\left(\dfrac{1}{3}xy^2-\dfrac{8}{7}xy^2\right)\)

\(=\left(-\dfrac{3}{4}x^2yz\right)\dfrac{1}{3}xy^2-\left(-\dfrac{3}{4}x^2yz\right)\dfrac{8}{7}xy^2\)

\(=-\dfrac{1}{4}x^3y^3z+\dfrac{6}{7}x^3y^3z\)

Answer 2

[Ngô Thanh Sang]

1. Ta có: \(-\dfrac{3}{4}x^2yz;B=\dfrac{1}{3}xy^2;C=-\dfrac{8}{7}xy^2\)

\(B+C=\dfrac{1}{3}xy^2-\dfrac{8}{7}xy^2=-\dfrac{17}{21}xy^2\)

\(A.\left(B+C\right)=\left(-\dfrac{3}{4}x^2yz\right).\left(-\dfrac{17}{21}xy^2\right)\)

\(\Rightarrow A.\left(B+C\right)=\dfrac{17}{28}x^3y^3z\)