Gọi là nghiệm của phương trình . Không giải phương trình, tính giá trị của biểu thức
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

-1/7 . 7/3 ; -4 ; -43/10
=-7/3 ; -4 ; -43/10
nhớ tính lại từ đây nha=-7/3 ; -4/1 ; -43/10
= -7/3 . -1/4 ; -43 /10
= 8/12; -43/10
=8/12 . -10/43
= -80/516

Lời giải:
Vì $x,y$ là 2 đại lượng tỉ lệ nghịch nên tích $xy=k$ không đổi với $k$ là số thực, hay còn được gọi là hệ số tỉ lệ.
Có:
$x_1y_1=x_2y_2=k$
$\Rightarrow 6y_1=-9y_2$
$\Rightarrow \frac{y_1}{-9}=\frac{y_2}{6}$
Áp dụng TCDTSBN: $\frac{y_1}{-9}=\frac{y_2}{6}=\frac{y_1-y_2}{-9-6}=\frac{10}{-15}=\frac{-2}{3}$
$\Rightarrow y_1=\frac{-2}{3}.(-9)=6; y_2=\frac{-2}{3}.6=-4$

Chọn C
Điều kiện
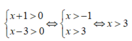
Ta có: log5(x+1) + log5( x-3) = 1
Tương đương : log5[(x+1)( x-3)] = 1 hay ( x+1) (x-3) = 5
=> x2- 3x+ x- 3= 5 nên x2- 2x-8= 0
Do đó; x= -2 hoặc x= 4
Mà x= -2 loại do đó đáp án đúng là C .

Bài 1
1)
a) x²(x - 2y) - 3xy(x - 2y)
= x(x - 2y)(x - 3y)
b) x² + 2xy + y² - 9z²
= (x² + 2xy + y²) - 9z²
= (x + y)² - (3z)²
= (x + y + 3z)(x + y - 3z)
2) 5x(x - 3) - x + 3 = 0
5x(x - 3) - (x - 3) = 0
(x - 3)(5x - 1) = 0
x - 3 = 0 hoặc 5x - 1 = 0
*) x - 3 = 0
x = 0 + 3
x = 3
*) 5x - 1 = 0
5x = 1
x = 1/5
Vậy x = 1/5; x = 3

a)
+) Ta có: a=3128 suy ra \(x = 3130\).
\(\left| {a - x} \right| = \left| {3128 - 3130} \right| = \left| { - 2} \right| = 2 \le 5\)
Vậy \(\left| {a - x} \right| \le 5\).
+) Ta có:
\(\begin{array}{l}x - 5 = 3128 - 5 = 3123\\x + 5 = 3128 + 5 = 3133\end{array}\)
Nên \(x - 5 \le a \le x + 5\)
b) Do y là số làm tròn đến hàng phần trăm của \(\frac{1}{3}\) nên \(y = 0,33\).
Ta có: \(\left| {\frac{1}{3} - y} \right| = \left| {\frac{1}{3} - 0,33} \right| = \left| {\frac{1}{{300}}} \right| = \frac{1}{{300}} = 0,00\left( 3 \right) \le 0,005\).
Nên \(\left| {\frac{1}{3} - y} \right| \le 0,005\).
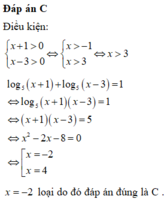


Phương trình: − x 2 − 4x + 6 = 0 có = ( − 4 ) 2 – 4.(− 1).6 = 40 > 0 nên phương trình có hai nghiệm x 1 ; x 2
Theo hệ thức Vi-ét ta có x 1 + x 2 = − b a x 1 . x 2 = c a ⇔ x 1 + x 2 = − 4 x 1 . x 2 = − 6
Ta có
N = 1 x 1 + 2 + 1 x 2 + 2 = x 1 + x 2 + 4 x 1 x 2 + 2 x 1 + x 2 + 4 = − 4 + 4 − 6 + 2. − 4 + 4
Đáp án: C