Chứng minh: \(\dfrac{3n+\left(-1\right)^n}{4n-\left(-1\right)^n}\) \(\le\)\(\dfrac{3n+4}{4n-1}\)Với mọi n thuộc tập hợp N*
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Câu 3:
\(u_1=\dfrac{2\cdot1+1}{1+2}=\dfrac{3}{3}=1\)
\(u_4=\dfrac{2\cdot4+1}{4+2}=\dfrac{9}{6}=\dfrac{3}{2}\)
\(u_5=\dfrac{2\cdot5+1}{5+2}=\dfrac{11}{7}\)
Câu 2:
\(u_n=u_1+\left(n-1\right)\cdot d\)
=>\(-3\left(n-1\right)+4=-41\)
=>-3(n-1)=-45
=>n-1=15
=>n=16
Câu 1:
Tổng của 50 số hạng đầu là 5150
=>\(\dfrac{n\cdot\left[2\cdot u_1+\left(n-1\right)\cdot d\right]}{2}=5150\)
=>\(\dfrac{50\left(2\cdot5+\left(50-1\right)\cdot d\right)}{2}=5150\)
=>\(25\left(10+49d\right)=5150\)
=>49d+10=206
=>49d=196
=>d=4
\(u_{10}=u_1+9d=5+9\cdot4=5+36=41\)

Câu 1:
-2;x;-18;y là cấp số nhân
=>\(\left\{{}\begin{matrix}x^2=\left(-2\right)\cdot\left(-18\right)\\\left(-18\right)^2=x\cdot y\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x^2=36\\xy=324\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}\left\{{}\begin{matrix}x=6\\y=\dfrac{324}{6}=54\end{matrix}\right.\\\left\{{}\begin{matrix}x=-6\\y=\dfrac{324}{-6}=-54\end{matrix}\right.\end{matrix}\right.\)
=>Chọn C
Câu 2:
\(u_4=u_2\cdot q^2\)
=>\(4q^2=9\)
=>\(q^2=\dfrac{9}{4}=\left(\dfrac{3}{2}\right)^2\)
=>\(\left[{}\begin{matrix}q=\dfrac{3}{2}\\q=-\dfrac{3}{2}\end{matrix}\right.\)
TH1: q=3/2
\(u_2=q\cdot u_1\)
=>\(u_1=\dfrac{u_2}{q}=4:\dfrac{3}{2}=4\cdot\dfrac{2}{3}=\dfrac{8}{3}\)
\(u_5=u_1\cdot q^4=\dfrac{8}{3}\cdot\left(\dfrac{3}{2}\right)^4=\dfrac{8}{3}\cdot\dfrac{81}{16}=\dfrac{27}{2}\)
\(u_8=u_1\cdot q^7=\dfrac{8}{3}\cdot\left(\dfrac{3}{2}\right)^7=\dfrac{2^3}{3}\cdot\dfrac{3^7}{2^7}=\dfrac{3^6}{2^4}=\dfrac{729}{16}\)
TH2: q=-3/2
\(u_1=\dfrac{u_2}{q}=4:\dfrac{-3}{2}=4\cdot\dfrac{-2}{3}=-\dfrac{8}{3}\)
\(u_5=u_1\cdot q^4=-\dfrac{8}{3}\cdot\left(-\dfrac{3}{2}\right)^4=-\dfrac{8}{3}\cdot\dfrac{81}{16}=\dfrac{-27}{2}\)
\(u_8=u_1\cdot q^7=\dfrac{-8}{3}\cdot\left(-\dfrac{3}{2}\right)^7=\dfrac{-2^3}{3}\cdot\dfrac{\left(-3\right)^7}{2^7}=\dfrac{2^3}{3}\cdot\dfrac{3^7}{2^7}=\dfrac{3^4}{2^4}=\dfrac{81}{16}\)
Câu 3:
\(\left\{{}\begin{matrix}u_1+u_5=51\\u_2+u_6=102\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}u_1+u_1\cdot q^4=51\\u_1\cdot q+u_1\cdot q^5=102\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}u_1+u_1\cdot q^4=51\\q\left(u_1+u_1\cdot q^4\right)=102\end{matrix}\right.\Leftrightarrow q=2\)
\(u_1+u_5=51\)
=>\(u_1\left(1+q^4\right)=51\)
=>\(u_1=\dfrac{51}{2^4+1}=\dfrac{51}{17}=3\)
\(u_4=u_1\cdot q^3=3\cdot2^3=24\)
\(u_{12}=u_1\cdot q^{11}=3\cdot2^{11}=6144\)

Câu 1: \(u_4=u_1+3k\)
=>\(3k=\dfrac{3}{8}-3=\dfrac{3}{8}-\dfrac{24}{8}=-\dfrac{21}{8}\)
=>\(k=-\dfrac{7}{8}\)
\(u_7=u_1+6k=3+6\cdot\dfrac{-7}{8}=3-\dfrac{42}{8}=\dfrac{24-42}{8}=-\dfrac{18}{8}=-\dfrac{9}{4}\)
Câu 2:
\(\dfrac{u_5}{u_8}=8\)
=>\(\dfrac{u_1\cdot q^4}{u_1\cdot q^7}=8\)
=>\(\dfrac{1}{q^3}=8\)
=>\(q=\dfrac{1}{2}\)
\(u_{12}=u_1\cdot q^{11}=12\cdot\left(\dfrac{1}{2}\right)^{11}=\dfrac{12}{2^{11}}=\dfrac{3}{2^9}\)
Câu 3:
Tổng của 5 số hạng đầu là:
\(S_5=\dfrac{u_1\cdot\left(1-q^5\right)}{1-q}=\dfrac{2\cdot\left(1-4^5\right)}{1-4}=682\)
=>Chọn D

Câu 25:
\(0< \alpha< \dfrac{\Omega}{2}\)
=>\(0< sin\alpha< 1;0< cos\alpha< 1\)
\(\sqrt{\dfrac{1+sin\alpha}{1-sin\alpha}}+\sqrt{\dfrac{1-sin\alpha}{1+sin\alpha}}\)
\(=\sqrt{\dfrac{\left(1+sin\alpha\right)^2}{\left(1-sin\alpha\right)\left(1+sin\alpha\right)}}+\sqrt{\dfrac{\left(1-sin\alpha\right)^2}{\left(1-sin\alpha\right)\left(1+sin\alpha\right)}}\)
\(=\sqrt{\dfrac{\left(1+sin\alpha\right)^2}{cos^2\alpha}}+\sqrt{\dfrac{\left(1-sin\alpha\right)^2}{cos^2\alpha}}\)
\(=\dfrac{1+sin\alpha+1-sin\alpha}{cos\alpha}=\dfrac{2}{cos\alpha}\)
Câu 28:
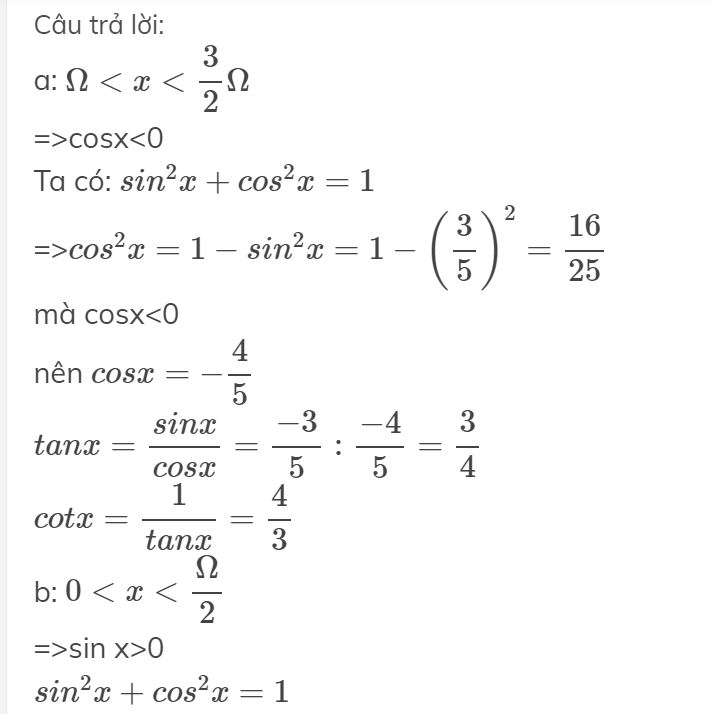
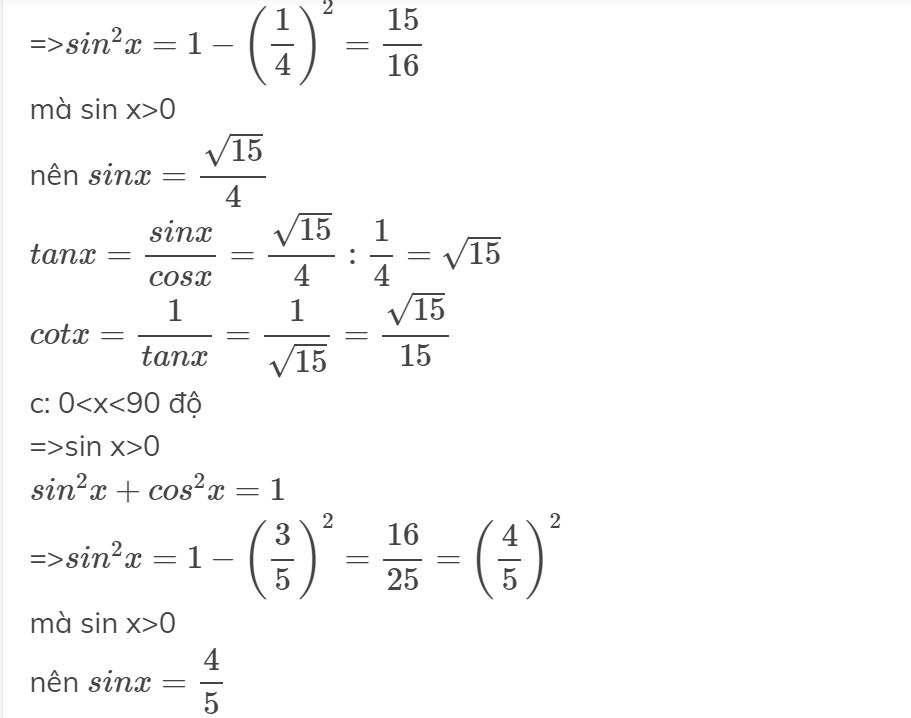


a, \(sin^2x+cos^2x=1\Leftrightarrow cos^2x=1-\dfrac{9}{25}=\dfrac{16}{25}\Leftrightarrow cosx=\dfrac{4}{5}\)
\(tanx=\dfrac{sinx}{cosx}=-\dfrac{3}{5}:\left(\dfrac{4}{5}\right)=-\dfrac{3}{4}\)
\(cotx=-\dfrac{4}{3}\)
c, \(sin^2x+cos^2x=1\Leftrightarrow sin^2x=1-\dfrac{9}{25}=\dfrac{16}{25}\Leftrightarrow sinx=\dfrac{4}{5}\)
\(tanx=\dfrac{sinx}{cosx}=\dfrac{4}{5}:\dfrac{3}{5}=\dfrac{4}{3}\)
\(cotx=\dfrac{3}{4}\)
b, \(cos^2x+sin^2x=1\Leftrightarrow sin^2x=1-\dfrac{1}{16}=\dfrac{15}{16}\Leftrightarrow sinx=\dfrac{\sqrt{15}}{4}\)
\(tanx=\dfrac{\sqrt{15}}{4}:\dfrac{1}{4}=\sqrt{15}\)
\(cotx=\dfrac{1}{\sqrt{15}}\)
d, \(sin^2x+cos^2x=1\Leftrightarrow sin^2x=1-\dfrac{25}{169}=\dfrac{144}{169}\Leftrightarrow sinx=\dfrac{12}{13}\)
\(tanx=\dfrac{12}{13}:\left(-\dfrac{5}{13}\right)=-\dfrac{12}{5}\)
\(cotx=-\dfrac{5}{12}\)
a: \(\Omega< x< \dfrac{3}{2}\Omega\)
=>cosx<0
Ta có: \(sin^2x+cos^2x=1\)
=>\(cos^2x=1-sin^2x=1-\left(\dfrac{3}{5}\right)^2=\dfrac{16}{25}\)
mà cosx<0
nên \(cosx=-\dfrac{4}{5}\)
\(tanx=\dfrac{sinx}{cosx}=\dfrac{-3}{5}:\dfrac{-4}{5}=\dfrac{3}{4}\)
\(cotx=\dfrac{1}{tanx}=\dfrac{4}{3}\)
b: \(0< x< \dfrac{\Omega}{2}\)
=>sin x>0
\(sin^2x+cos^2x=1\)
=>\(sin^2x=1-\left(\dfrac{1}{4}\right)^2=\dfrac{15}{16}\)
mà sin x>0
nên \(sinx=\dfrac{\sqrt{15}}{4}\)
\(tanx=\dfrac{sinx}{cosx}=\dfrac{\sqrt{15}}{4}:\dfrac{1}{4}=\sqrt{15}\)
\(cotx=\dfrac{1}{tanx}=\dfrac{1}{\sqrt{15}}=\dfrac{\sqrt{15}}{15}\)
c: 0<x<90 độ
=>sin x>0
\(sin^2x+cos^2x=1\)
=>\(sin^2x=1-\left(\dfrac{3}{5}\right)^2=\dfrac{16}{25}=\left(\dfrac{4}{5}\right)^2\)
mà sin x>0
nên \(sinx=\dfrac{4}{5}\)
\(tanx=\dfrac{sinx}{cosx}=\dfrac{4}{5}:\dfrac{3}{5}=\dfrac{4}{3}\)
\(cotx=1:\dfrac{4}{3}=\dfrac{3}{4}\)
d: \(180^0< x< 270^0\)
=>sin x<0
\(sin^2x+cos^2x=1\)
=>\(sin^2x=1-\left(-\dfrac{5}{13}\right)^2=1-\dfrac{25}{169}=\dfrac{144}{169}\)
mà sin x<0
nên \(sinx=-\dfrac{12}{13}\)
\(tanx=\dfrac{sinx}{cosx}=\dfrac{-12}{13}:\dfrac{-5}{13}=\dfrac{12}{5}\)
\(cotx=\dfrac{1}{tanx}=\dfrac{5}{12}\)

\(A=2\cdot cos\left(\dfrac{\Omega}{2}+x\right)+sin\left(5\Omega-x\right)+sin\left(\dfrac{3\Omega}{2}+x\right)+cos\left(\dfrac{\Omega}{2}+x\right)\)
\(=3\cdot cos\left(\dfrac{\Omega}{2}+x\right)+sin\left(\Omega-x\right)+sin\left(\dfrac{\Omega}{2}+\Omega+x\right)\)
\(=-3\cdot sinx+sinx+cos\left(\Omega+x\right)\)
\(=-2\cdot sinx-cosx\)
\(B=sin\left(\Omega+x\right)-cos\left(\dfrac{\Omega}{2}+x\right)+cot\left(2\Omega-x\right)+tan\left(\dfrac{2\Omega}{2}+x\right)\)
\(=-sinx+sinx+cot\left(-x\right)+tan\left(x\right)\)
\(=tanx-cotx=tanx-\dfrac{1}{tanx}=\dfrac{tan^2x-1}{tanx}\)

Câu 1:
\(2\sin x-\sqrt{3}=0\\ \Leftrightarrow\sin x=\dfrac{\sqrt{3}}{2}=\sin\dfrac{\pi}{3}\\ \Leftrightarrow\left[{}\begin{matrix}x_1=\dfrac{\pi}{3}+k_12\pi\\x_2=\pi-\dfrac{\pi}{3}+k_22\pi=\dfrac{2\pi}{3}+k_22\pi\end{matrix}\right.\left(k_1,k_2\inℤ\right)\)
Mà: \(x\in\left[0;2\pi\right]\) do đó nên: \(k_1=0,k_2=0\)
Vậy tập nghiệm pt là: \(S=\left\{\dfrac{\pi}{3};\dfrac{2\pi}{3}\right\}\) (2 nghiệm => D)
Câu 2:
Vì: \(-1\le\cos x\le1\forall x\)
\(\Rightarrow-1\le m+1\le1\\ \Leftrightarrow-2\le m\le0\)
Mà: \(m\inℤ\Rightarrow m\in\left\{-2;-1;0\right\}\) (C)
Câu 1: \(2\cdot sinx-\sqrt{3}=0\)
=>\(sinx=\dfrac{\sqrt{3}}{2}\)
=>\(\left[{}\begin{matrix}x=\dfrac{\Omega}{3}+k2\Omega\\x=\Omega-\dfrac{\Omega}{3}+k2\Omega=\dfrac{2}{3}\Omega+k2\Omega\end{matrix}\right.\)
Để \(x\in\left[0;2\Omega\right]\) thì \(\left[{}\begin{matrix}\dfrac{\Omega}{3}+k2\Omega\in\left[0;2\Omega\right]\\\dfrac{2}{3}\Omega+k2\Omega\in\left[0;2\Omega\right]\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}2k+\dfrac{1}{3}\in\left[0;2\right]\\2k+\dfrac{2}{3}\in\left[0;2\right]\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}2k\in\left[-\dfrac{1}{3};\dfrac{5}{3}\right]\\2k\in\left[-\dfrac{2}{3};\dfrac{4}{3}\right]\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}k\in\left[-\dfrac{1}{6};\dfrac{5}{6}\right]\\k\in\left[-\dfrac{1}{3};\dfrac{2}{3}\right]\end{matrix}\right.\Leftrightarrow k=0\)
=>Chọn B
Câu 2:
Để phương trình cosx =m+1 có nghiệm thì -1<=m+1<=1
=>-2<=m<=0
mà m nguyên
nên \(m\in\left\{-2;-1;0\right\}\)
=>Chọn C
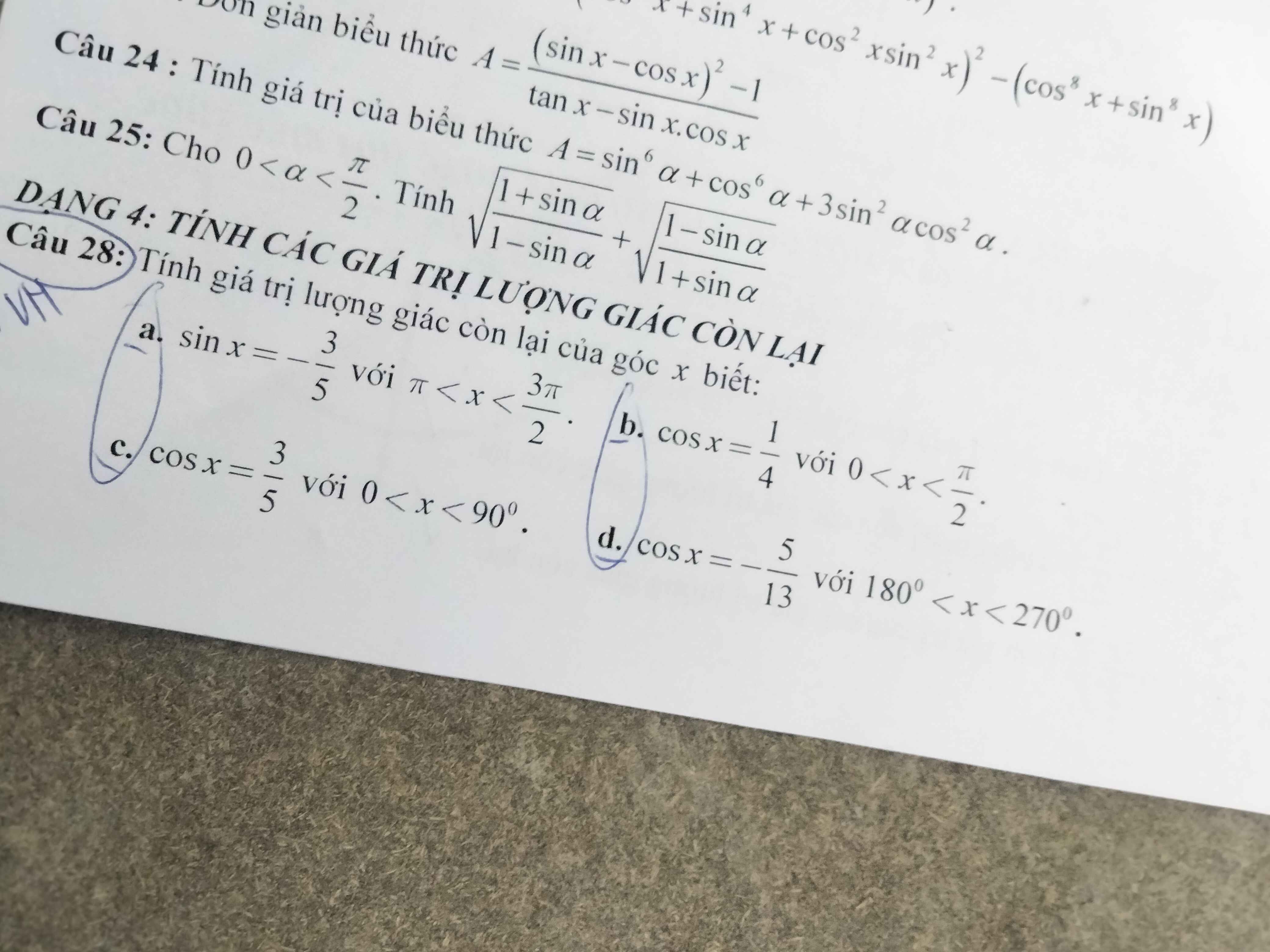
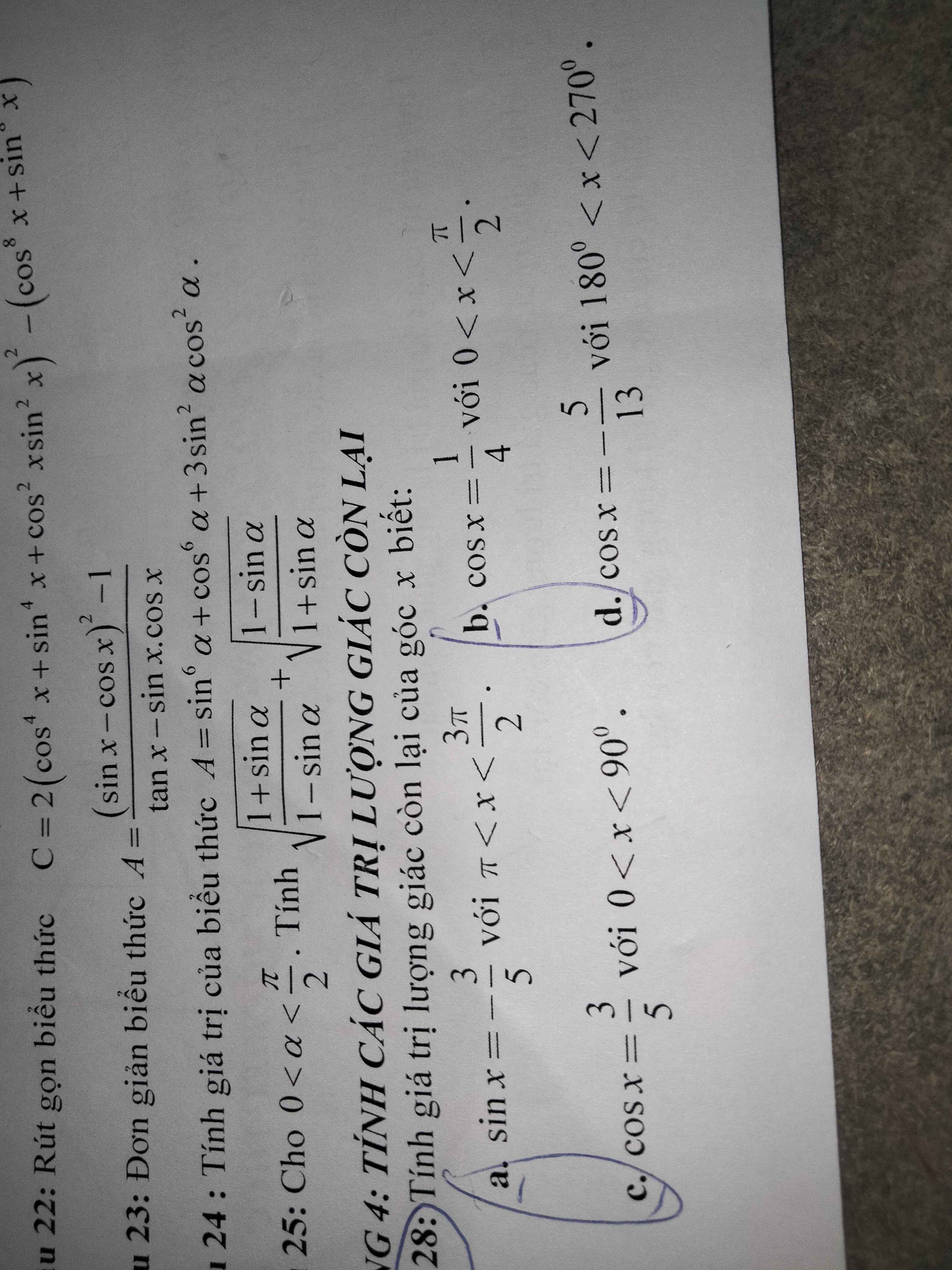
Nếu \(n\) chẵn thì đpcm trở thành \(\dfrac{3n+1}{4n-1}\le\dfrac{3n+4}{4n-1}\) \(\Leftrightarrow3n+1\le3n+4\) \(\Leftrightarrow1\le4\), luôn đúng.
Nếu \(n\) lẻ thì đpcm thành \(\dfrac{3n-1}{4n+1}\le\dfrac{3n+4}{4n-1}\)
\(\Leftrightarrow\left(3n-1\right)\left(4n-1\right)\le\left(4n+1\right)\left(3n+4\right)\)
\(\Leftrightarrow12n^2-3n-4n+1\le12n^2+16n+3n+4\)
\(\Leftrightarrow26n+3\ge0\) (luôn đúng)
Vậy với mọi \(n\inℕ^∗\) thì \(\dfrac{3n+\left(-1\right)^n}{4n-\left(-1\right)^n}\le\dfrac{3n+4}{4n-1}\)