Ở một tế bào sinh tinh, xét một cặp gen dị hợp đều dài 4080A. Alen A có 580 Ađênin, alen a có 650 Ađênin. Trong kì sau của giảm phân I cặp nhiễm sắc thể chứa gen nói trên không phân li, giảm phân II diễn ra bình thường. Xác định số nuclêôtit từng loại trong các giao tử được tạo thành.
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
10 tháng 6 2023
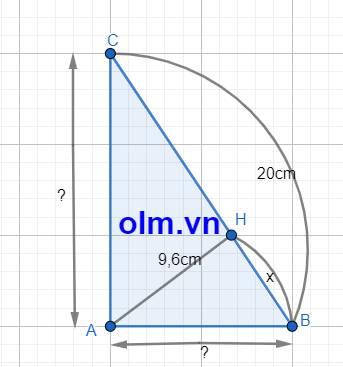
Gọi độ dài đoạn BH là: \(x\) ( cm) ; \(x\) > 0; AC > AB nên \(x\) < CH
Xét tam giác vuông HAB vuông tại H theo pytago ta có:
AB2 = HA2 + HB2 = 9,62 + \(x^2\) = 92,16 + \(x^2\)
Xét tam giác vuông AHC vuông tại H theo pytago ta có:
AC2 = HA2 + HC2 = 9,62 + (\(20-x\))2 = 92,16 + 400 - 40\(x\) + \(x^2\)
AC2 = 492,16 - 40\(x\) + \(x^2\)
Xét tam giác vuông ABC vuông tại A theo pytago ta có:
AC2 + AB2 = BC2
492,16 - 40\(x\) + \(x^2\) + 92,16 + \(x^2\) = 202
(\(x^2\) + \(x^2\)) - 40\(x\) + (492,16 + 92,16) - 400 = 0
2\(x^2\) - 40\(x\) + 584,32 - 400 = 0
2\(x^2\)- 40\(x\) + 184,32 =0
\(x^2\) - 20\(x\) + 92,16 = 0
△' = 102 - 92,16 = 7,84 > 0
\(x\)1 = -(-10) + \(\sqrt{7,84}\) = 12,8 ⇒ CH = 20 - 12,8 = 7,2 < BH (loại )
\(x_2\) = -(-10) - \(\sqrt{7,84}\) = 7,2 ⇒ CH = 20 - 7,2 = 12,8 (thỏa mãn)
Thay \(x_2\) = 7,2 vào biểu thức: AB2 = 92,16 + \(x^2\) = 92,16 + 7,22 = 144
⇒AB = \(\sqrt{144}\) = 12
Thay \(x_2\) = 7,2 vào biểu thức: AC2 = 492,16 - 40\(x\) + \(x^2\)
AC2 = 492,16 - 40\(\times\) 7,2 + 7,22 = 256
AC = \(\sqrt{256}\) = 16
Kết luận AB = 12 cm; AC = 16 cm
NA
1
Y
9 tháng 6 2023
\(\dfrac{1}{2\sqrt{3}-3\sqrt{2}}+\dfrac{1}{2\sqrt{3}+3\sqrt{2}}\)
\(=\dfrac{2\sqrt{3}+3\sqrt{2}+2\sqrt{3}-3\sqrt{2}}{\left(2\sqrt{3}-3\sqrt{2}\right)\left(2\sqrt{3}+3\sqrt{2}\right)}\)
\(=\dfrac{\left(2\sqrt{3}+2\sqrt{3}\right)+\left(3\sqrt{2}-3\sqrt{2}\right)}{\left(2\sqrt{3}\right)^2-\left(3\sqrt{2}\right)^2}\)
\(=\dfrac{4\sqrt{3}+0}{4.3-9.2}\)
\(=\dfrac{4\sqrt{3}}{12-18}\)
\(=\dfrac{4\sqrt{3}}{-6}\)
\(=-\dfrac{2\sqrt{3}}{3}\)
8 tháng 6 2023
\(\dfrac{1}{x}+\dfrac{2}{y}\le1\Rightarrow\dfrac{2}{y}\le1-\dfrac{1}{x}\Rightarrow y\ge\dfrac{2x}{x-1}=2+\dfrac{2}{x-1}\)
\(x+\dfrac{2}{z}\le3\Rightarrow x< 3;\dfrac{2}{z}\le3-x\Rightarrow z\ge\dfrac{2}{3-x}\Rightarrow y+z\ge2+\dfrac{2}{x-1}+\dfrac{2}{3-x}\)
Lúc này ta sẽ áp dụng bất đẳng thức Bunhiacopski
Ta có:
\(6^2\le\left(y+z\right)^2=\left(\sqrt{2}\dfrac{y}{\sqrt{2}}Z\right)^2\le3\left(\dfrac{y^2}{2}+z^2\right)=\dfrac{3}{2}\left(y^2+2z^2\right)\)
\(\Rightarrow P\ge24\). Dấu đẳng thức xảy ra khi và chỉ khi \(y=4,z=2\)
Vậy giá trị nhỏ nhật của P là 24
CD
2
8 tháng 6 2023
Có vẻ bạn bị sai đề bài ở chỗ 4088403 nếu là 4088483 sẽ giải được
\(A=\dfrac{1}{3}+\dfrac{1}{3.5}+\dfrac{1}{7.5}+\dfrac{1}{7.9}+...+\dfrac{1}{2021.2023}\)
\(=1-\dfrac{1}{3}+\dfrac{1}{3}-\dfrac{1}{5}+\dfrac{1}{5}-\dfrac{1}{7}+\dfrac{1}{7}-\dfrac{1}{9}+\dfrac{1}{9}+...+\dfrac{1}{2021}-\dfrac{1}{2023}\)
\(=1-\dfrac{1}{2023}\)
\(=\dfrac{2022}{2023}\)
8 tháng 6 2023
Ta có \(p=x^2+y^2\ge\dfrac{\left(x+y\right)^2}{2}=2\). Ta đi tìm GTNN của \(B=p+\dfrac{1}{p}\).
Do \(B=\dfrac{p}{4}+\dfrac{1}{p}+\dfrac{3p}{4}\) \(\ge2\sqrt{\dfrac{p}{4}.\dfrac{1}{p}}+\dfrac{3.2}{4}\) \(=\dfrac{5}{2}\). ĐTXR \(\Leftrightarrow\left\{{}\begin{matrix}x=y\\p=2\end{matrix}\right.\) \(\Leftrightarrow x=y=1\).
Vậy GTNN của B là \(\dfrac{5}{2}\) khi \(x=y=1\)
A
1
8 tháng 6 2023
pt đã cho
\(\Leftrightarrow2x^2-5x+2-\left(x-2\right)\sqrt{x^2-x+1}=0\)
\(\Leftrightarrow\left(x-2\right)\left(2x-1-\sqrt{x^2-x+1}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=2\\2x-1-\sqrt{x^2-x+1}=0\end{matrix}\right.\)
(*) \(2x-1-\sqrt{x^2-x+1}=0\) (đk: \(x\ge\dfrac{2+\sqrt{3}}{4}\))
Ta thấy \(2x-1+\sqrt{x^2-x+1}\ne0\) với mọi \(x\ge\dfrac{2+\sqrt{3}}{4}\), (*) tương đương:
\(\dfrac{\left(2x-1\right)^2-\left(x^2-x+1\right)}{2x-1+\sqrt{x^2-x+1}}=0\)
\(\Leftrightarrow\dfrac{3x\left(x-1\right)}{2x-1+\sqrt{x^2-x+1}}=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\left(nhận\right)\\\dfrac{3}{2x-1+\sqrt{x^2-x+1}}=0\left(vôlí\right)\end{matrix}\right.\)
Vậy pt đã cho có tập nghiệm \(S=\left\{1;2\right\}\)