HT
2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
CM
31 tháng 10 2017
Đáp án đúng: C
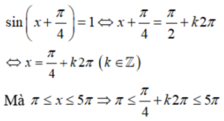
⇔ 3 4 ≤ k ≤ 19 8 ⇒ k ∈ 1 ; 2
Vậy phương trình có 2 nghiệm trong π ; 5 π
PT
1
CM
3 tháng 10 2018
sin π/6 = 1/2; cos π/6 = √3/2
sin π/4 = √2/2; cos π/4 = √2/2
sin 1,5 = 0,9975; cos 1,5 = 0,0707
sin 2 = 0,9093; cos 2 = -0,4161
sin 3,1 = 0,0416; cos 3,1 = -0,9991
sin 4,25 = -0,8950; cos 4,25 = -0,4461
sin 5 = -0,9589; cos 5 = 0,2837
Y
1
NV
Nguyễn Việt Lâm
Giáo viên
19 tháng 9 2021
\(\Leftrightarrow2cosx+2cos3x=cosx-\sqrt{3}sinx\)
\(\Leftrightarrow\dfrac{1}{2}cosx+\dfrac{\sqrt{3}}{2}sinx=-cos3x\)
\(\Leftrightarrow cos\left(x-\dfrac{\pi}{3}\right)=cos\left(\pi-3x\right)\)
\(\Leftrightarrow...\)
1 tháng 9 2021
y = \(\dfrac{sin^2x}{cosx\left(sinx-cosx\right)}+\dfrac{1}{4}\)
y = \(\dfrac{sin^2x}{sinx.cosx-cos^2x}+\dfrac{1}{4}=\dfrac{\dfrac{sin^2x}{cos^2x}}{\dfrac{sinx.cosx}{cos^2x}-1}+\dfrac{1}{4}\)
y = \(\dfrac{tan^2x}{tanx-1}+\dfrac{1}{4}\)
y = \(\dfrac{4tan^2x+tanx-1}{4tanx-4}\). Đặt t = tanx. Do x ∈ \(\left(\dfrac{\pi}{4};\dfrac{\pi}{2}\right)\) nên t ∈ (1 ; +\(\infty\))\
Ta đươc hàm số f(t) = \(\dfrac{4t^2+t-1}{4t-4}\)
⇒ ymin = \(\dfrac{17}{4}\) khi t = 2. hay x = arctan(2) + kπ
QV
Có bao nhiêu giá trị nguyên của m để pt sin2 x - sinx - 3 -m = 0 có nghiệm duy nhất thuộc [-π/2;π/2]
0
NV
Nguyễn Việt Lâm
Giáo viên
31 tháng 10 2020
\(\Leftrightarrow\left[{}\begin{matrix}cosx=1\\sin^2x+sinx+m=0\end{matrix}\right.\)
Pt \(cosx=1\Leftrightarrow x=k2\pi\) có 2 nghiệm trên đoạn đã cho
\(\Rightarrow sin^2x+sinx+m=0\) (1) có 4 nghiệm trên đoạn đã cho
Đặt \(sinx=t\Rightarrow t^2+t=-m\)
Trên \(\left[0;2\pi\right]\) ứng với mỗi \(sinx=t\) có tối đa 2 giá trị x
Pt \(t^2+t=-m\) cũng có tối đa 2 nghiệm \(t\)
Do đó để (1) có 4 nghiệm \(\Leftrightarrow\left\{{}\begin{matrix}sinx=t\\t^2+t=-m\end{matrix}\right.\) đều có 2 nghiệm pb
\(\Leftrightarrow\left\{{}\begin{matrix}-1< t< 1\\t^2+t=-m\end{matrix}\right.\)
Xét \(f\left(t\right)=t^2+t\) trên \(\left(-1;1\right)\)
\(-\frac{b}{2a}=-\frac{1}{2}\) ; \(f\left(-\frac{1}{2}\right)=-\frac{1}{4}\) ; \(f\left(1\right)=2\) ; \(f\left(-1\right)=0\)
\(\Rightarrow y=-m\) cắt \(y=f\left(t\right)\) tại 2 điểm pb \(\Leftrightarrow-\frac{1}{4}< -m< 0\)
\(\Leftrightarrow0< m< \frac{1}{4}\)
LV
2
AH
Akai Haruma
Giáo viên
21 tháng 8 2023
Lời giải:
$m^2=(\sin x+\cos x)^2=\sin ^2x+\cos ^2x+2\sin x\cos x=1+2\sin x\cos x$
$\Rightarrow \sin x\cos x=\frac{m^2-1}{2}$
Ta có:
$|\sin ^3x-\cos ^3x|=|\sin x-\cos x||\sin ^2x+\sin x\cos x+\cos ^2x|$
$=\sqrt{(\sin x-\cos x)^2}|1+\sin x\cos x|$
$=\sqrt{1-2\sin x\cos x}.|1+\sin x\cos x|$
$=\sqrt{1-(m^2-1)}.|1+\frac{m^2-1}{2}|$
$=\sqrt{2-m^2}.\frac{m^2+1}{2}$
H
21 tháng 8 2023
\(sinx+cosx=m\\ \Rightarrow sin^2x+cos^2x+2sinx.cosx=m^2\\ \Rightarrow sinx.cosx=\dfrac{1-m^2}{2}\)
Mặt khác:
\(sinx-cosx=\left(sinx+cosx\right)-2cosx=m-2cosx\)
Có:
\(\left|sin^3x-cos^3x\right|=\left|\left(sinx-cosx\right)\left(sin^2x+sinx.cosx+cos^2x\right)\right|\\ =\left|\left(m-2cosx\right)\left(1+\dfrac{1-m^2}{2}\right)\right|\\ =\left|\left(m-2cosx\right)\left(\dfrac{3-m^2}{2}\right)\right|\)
NV
Nguyễn Việt Lâm
Giáo viên
14 tháng 9 2020
a/ \(y=sin2x+\left(\sqrt{3}+1\right)cos2x+sin^2x-cos^2x-1\)
\(=sin2x+\sqrt{3}cos2x-1=2sin\left(2x+\frac{\pi}{3}\right)-1\)
Do \(-1\le sin\left(2x+\frac{\pi}{3}\right)\le1\Rightarrow-3\le y\le1\)
b/ \(y=2sin^2x-2cos^2x-3sinx.cosx-1\)
\(=-2cos2x-\frac{3}{2}sin2x-1=-\frac{5}{2}\left(\frac{3}{5}sinx+\frac{4}{5}cosx\right)-1\)
\(=-\frac{5}{2}sin\left(x+a\right)-1\Rightarrow-\frac{7}{2}\le y\le\frac{3}{2}\)
c/ \(y=1-sin2x+2cos2x+\frac{3}{2}sin2x=\frac{1}{2}sin2x+2cos2x+1\)
\(=\frac{\sqrt{17}}{2}\left(\frac{1}{\sqrt{17}}sin2x+\frac{4}{\sqrt{17}}cos2x\right)+1=\frac{\sqrt{17}}{2}sin\left(2x+a\right)+1\)
\(\Rightarrow-\frac{\sqrt{17}}{2}+1\le y\le\frac{\sqrt{17}}{2}+1\)
\(x\in\left(\dfrac{5}{2}\Omega;3\Omega\right)\)
=>\(x\in\left(\dfrac{\Omega}{2};\Omega\right)\)
=>\(cosx< 0\)
=>\(cosx=-\sqrt{1-\left(\dfrac{5}{8}\right)^2}=-\sqrt{1-\dfrac{25}{64}}=-\sqrt{\dfrac{39}{64}}=-\dfrac{\sqrt{39}}{8}\)
\(sin\left(x+\dfrac{\Omega}{3}\right)\)
\(=sinx\cdot cos\left(\dfrac{\Omega}{3}\right)+cosx\cdot sin\left(\dfrac{\Omega}{3}\right)\)
\(=\dfrac{5}{8}\cdot\dfrac{1}{2}+\dfrac{-\sqrt{39}}{8}\cdot\dfrac{\sqrt{3}}{2}=\dfrac{5-\sqrt{117}}{16}\)
Sinx =0,01827602763