Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Với m = 1 ta có phương trình:
\(x^2-2x+1=0\)
Sử dụng đen ta ta có: \(\Delta=\left(-2\right)^2-4.1.1=0\)
nên phương trình có nghiệm kép \(x_1=x_2=\frac{2}{2}=1\)
Vậy phương trình trên có nghiệm x = 1
b) Đặt phương trình \(x^2-\left(3m-1\right)x+2m^2-m=0\left(1\right)\) \(\Rightarrow\Delta>0\)
\(\Leftrightarrow\left[-\left(3m-1\right)\right]^2-4.1.\left(2m^2-m\right)>0\)
\(\Leftrightarrow\left(3m-1\right)^2-4\left(2m^2-m\right)>0\)
\(\Leftrightarrow9m^2-6m+1-8m^2+4m>0\)
\(\Leftrightarrow m^2-2m+1>0\)
\(\Leftrightarrow\left(m-1\right)^2>0\Leftrightarrow m-1\ne0\Leftrightarrow m\ne1\)
\(\left|x_1-x_2\right|-2=0\Leftrightarrow\left|x_1-x_2\right|=2\)
\(\Leftrightarrow\left(x_1-x_2\right)^2=4\Leftrightarrow\left(x_1+x_2\right)^2-4x_1x_2=4\)\(\left(2\right)\)
Áp dụng hệ thức Vi-ét cho phương trình ( 1 ) ta có:
\(\hept{\begin{cases}x_1+x_2=3m-1\\x_1x_2=2m^2-m\end{cases}}\)
từ ( 2 ) suy ra \(\left(3m-1\right)^2-4\left(2m^2-m\right)=4\)
\(\Leftrightarrow9m^2-6m+1-8m^2+4m=4\)
\(\Leftrightarrow m^2-2m+1-4=0\)
\(\Leftrightarrow m^2-2m-3=0\Leftrightarrow\)\(\left(m+1\right)\left(m-3\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}m+1=0\\m-3=0\end{cases}\Leftrightarrow\orbr{\begin{cases}m=-1\left(tmđk\right)\\m=3\left(tmđk\right)\end{cases}}}\)
Vậy \(m=-1;m=3\)thỏa mãn yêu cầu đề bài đã cho

Vì PT(1) có nghiệm bằng 3 nên thay x=3 vào PT ,ta có :
\(\left(1\right)\Leftrightarrow3^2-3\left(m+1\right)+2m-3=0\)
\(\Leftrightarrow3-m=0\)
\(\Leftrightarrow m=3\)
Vậy m=3 thì P)T(1) có nghiệm x=3
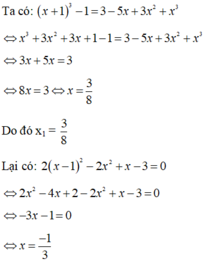
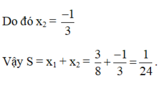
Lời giải:
Ta có:
\(\Delta=(2m-1)^2-4m(m-1)=1>0\) nên pt có hai nghiệm phân biệt với mọi \(m\in\mathbb{R}\)
Khi đó áp dụng định lý Viete cho pt bậc 2, ta có:
\(\left\{\begin{matrix} x_1+x_2=2m-1\\ x_1x_2=m(m-1)\end{matrix}\right.(*)\)
Dựa vào (*):
\(3x_1+2x_2=1\Leftrightarrow x_1+2(x_1+x_2)=1\)
\(\Leftrightarrow x_1+2(2m-1)=1\Leftrightarrow x_1=3-4m\)
\(\Rightarrow x_2=2m-1-x_1=2m-1-(3-4m)=6m-4\)
Suy ra: \(x_1x_2=(3-4m)(6m-4)\)
\(\Leftrightarrow m(m-1)=18m-12-24m^2+16m\)
\(\Leftrightarrow 25m^2-35m+12=0\)
\(\Leftrightarrow (5m-4)(5m-3)=0 \Rightarrow \left[\begin{matrix} m=\frac{4}{5}\\ m=\frac{3}{5}\end{matrix}\right.\) (đều thỏa mãn)
Vậy..........