Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

ĐKXĐ:\(x\ge0;y\ge1;z\ge2\)
\(\sqrt{x}+\sqrt{y-1}+\sqrt{z-2}=\frac{x+y+z}{2}\)
\(\Leftrightarrow2\sqrt{x}+2\sqrt{y-1}+2\sqrt{z-2}=x+y+z\)
\(\Leftrightarrow\left(x-2\sqrt{x}+1\right)+\left(y-1+2\sqrt{y-1}+1\right)+\left(z-2+2\sqrt{z-2}+1\right)=0\)
\(\Leftrightarrow\left(\sqrt{x}-1\right)^2+\left(\sqrt{y-1}-1\right)^2+\left(\sqrt{z-2}-2\right)^2=0\)
Mà \(\left\{\begin{matrix}\left(\sqrt{x-1}-1\right)^2\ge0\\\left(\sqrt{y-1}-1\right)^2\ge0\\\left(\sqrt{z-2}-2\right)^2\ge0\end{matrix}\right.\)\(\forall x;y;z\)
\(\Rightarrow\left\{\begin{matrix}\left(\sqrt{x-1}-1\right)^2=0\\\left(\sqrt{y-1}-1\right)^2=0\\\left(\sqrt{z-2}-2\right)^2=0\end{matrix}\right.\)\(\Leftrightarrow\left\{\begin{matrix}\sqrt{x-1}-1=0\\\sqrt{y-1}-1=0\\\sqrt{z-2}-2=0\end{matrix}\right.\)
\(\Leftrightarrow\left\{\begin{matrix}\sqrt{x-1}=1\\\sqrt{y-1}=1\\\sqrt{z-2}=2\end{matrix}\right.\)\(\Leftrightarrow\left\{\begin{matrix}x-1=1\\y-1=1\\z-2=4\end{matrix}\right.\)\(\Leftrightarrow\left\{\begin{matrix}x=2\\y=2\\z=6\end{matrix}\right.\)
=> x02 + y02 + z02 = 22 + 22 + 62 = 44


a) \(16x^2-\left(4x-5\right)^2=15\) \(\Leftrightarrow\) \(16x^2-\left(16x^2-40x+25\right)=15\)
\(\Leftrightarrow\) \(16x^2-16x^2+40x-25=15\) \(\Leftrightarrow\) \(40x-25=15\)
\(\Leftrightarrow\) \(40x=40\) \(\Leftrightarrow\) \(x=1\) vậy \(x=1\)
b) \(\left(2x+3\right)^2-4\left(x-1\right)\left(x+1\right)=49\)
\(\Leftrightarrow\) \(4x^2+12x+9-4\left(x^2-1\right)=49\)
\(\Leftrightarrow\) \(4x^2+12x+9-4x^2+4=49\)
\(\Leftrightarrow\) \(12x+13=49\) \(\Leftrightarrow\) \(12x=36\) \(\Leftrightarrow\) \(x=\dfrac{36}{12}=3\)vậy \(x=3\)
c) \(\left(2x+1\right)\left(2x-1\right)+\left(1-2x\right)^2=18\)
\(\Leftrightarrow\) \(4x^2-1+1-4x+4x^2=18\)\(\Leftrightarrow\) \(8x^2-4x=18\)
\(\Leftrightarrow\) \(8x^2-4x-18=0\)
\(\Delta'=\left(-2\right)^2-8.\left(-18\right)=4+144=148>0\)
\(\Rightarrow\) phương trình có 2 nghiệm phân biệt
\(x_1=\dfrac{2+\sqrt{148}}{8}=\dfrac{1+\sqrt{37}}{4}\)
\(x_2=\dfrac{2-\sqrt{148}}{8}=\dfrac{1-\sqrt{37}}{4}\)
vậy \(x=\dfrac{1+\sqrt{37}}{4};x=\dfrac{1-\sqrt{37}}{4}\)
Giải:
a) \(16x^2-\left(4x-5\right)^2=15\)
\(\Leftrightarrow16x^2-16x^2-40x+25=15\)
\(\Leftrightarrow-40x+25=15\)
\(\Leftrightarrow-40x=15-25=-10\)
\(\Leftrightarrow x=-\dfrac{10}{-40}=\dfrac{1}{4}\)
Vậy \(x=\dfrac{1}{4}\)
b) \(\left(2x+3\right)^2-4\left(x-1\right)\left(x+1\right)=49\)
\(\Leftrightarrow4x^2+12x+9-4\left(x^2-1^2\right)=49\)
\(\Leftrightarrow4x^2+12x+9-4x^2+4=49\)
\(\Leftrightarrow12x+9+4=49\)
\(\Leftrightarrow12x=49-9-4\)
\(\Leftrightarrow12x=36\)
\(\Leftrightarrow x=\dfrac{36}{12}=3\)
Vậy \(x=3\)
c) \(\left(2x+1\right)\left(2x-1\right)+\left(1-2x\right)^2=18\)
\(\Leftrightarrow4x^2-1+1-4x+4x^2=18\)
\(\Leftrightarrow8x^2-4x=18\)
Mình chỉ làm được đến đây thôi, hình như là đề bị sai bạn nhé!
Chúc bạn học tốt!

Bài 1:
\(P=3^x\left(3+3^2+3^3+3^4\right)+...+3^{x+96}\left(3+3^2+3^3+3^4\right)\)
\(=120\left(3^x+...+3^{x+96}\right)⋮120\)

giao điểm (d1) ;và (d2) thỏa he :\(\left\{{}\begin{matrix}2x+my+m+1=0\\\left(m+1\right)x+y+2m=0\end{matrix}\right.\)(I)
\(\Rightarrow\)(I) có nghiệm khi \(m^2+m-2\ne0\Leftrightarrow m\ne1;m\ne-2\)(\(\circledast\))
nghiệm của(I) \(\left\{{}\begin{matrix}x=\dfrac{2m+1}{m+2}=2-\dfrac{3}{m+2}\left(1\right)\\y=\dfrac{m-1}{m+2}=1-\dfrac{3}{m+2}\left(2\right)\end{matrix}\right.\)
lấy về trừ theo về cửa (1) chờ (2) tá dược: x-y = 1
vậy giao điểm của d1 va d2 luôn di động trên đường thẳng : x -y -1 = 0
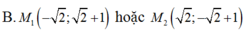
Đáp án A
- Do M thuộc d suy ra M( t; -1-t).
Nếu 2 tiếp tuyến vuông góc với nhau thì MAIB là hình vuông
(A; B là 2 tiếp điểm).
Do đó:
- Ta có :
- Do đó : 2t2+ 8= 12