
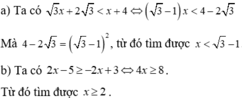
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

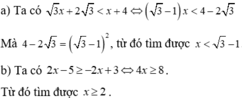

a) \(\frac{x^2+2}{5}\ge0\)
\(\Rightarrow x^2+2\ge0\)( đúng với mọi x )
Vậy \(S=\left\{ℝ\right\}\)
b) \(\frac{x+2}{x-3}< 0\)( ĐKXĐ : \(x\ne3\))
Xét hai trường hợp :
1. \(\hept{\begin{cases}x+2< 0\\x-3>0\end{cases}}\Rightarrow\hept{\begin{cases}x< -2\\x>3\end{cases}}\)( loại )
2. \(\hept{\begin{cases}x+2>0\\x-3< 0\end{cases}}\Rightarrow\hept{\begin{cases}x>-2\\x< 3\end{cases}\Rightarrow}-2< x< 3\)
Vậy nghiệm của bất phương trình là -2 < x < 3
c) \(\frac{x-1}{x-3}>1\)( ĐKXĐ : \(x\ne3\))
\(\Leftrightarrow\frac{x-3+2}{x-3}>1\)
\(\Leftrightarrow1+\frac{2}{x-3}>1\)
\(\Leftrightarrow\frac{2}{x-3}>0\)
\(\Leftrightarrow x-3>0\)
\(\Leftrightarrow x>3\)
Vậy nghiệm của bất phương trình là x > 3
Nhờ bạn khác vẽ trục số nhé vì mình mới lên lớp 8

\(\frac{x+4}{5}+\frac{3x+2}{10}< \frac{x-1}{3}\)
\(\Leftrightarrow\frac{6\left(x+4\right)}{30}+\frac{3\left(3x+2\right)}{30}< \frac{10\left(x-1\right)}{30}\)
\(\Leftrightarrow6x+24+9x+6< 10x-10\)
\(\Leftrightarrow5x+40< 0\)
\(\Leftrightarrow x< -8\)
Tự biểu diễn nha bạn
\(\frac{x+4}{5}+\frac{3x+2}{10}< \frac{x-1}{3}\)
\(\Rightarrow\frac{6\left(x+4\right)}{30}+\frac{3\left(3x+2\right)}{30}< \frac{10\left(x-1\right)}{30}\)
\(\Rightarrow6x+24+9x+6< 10x-10\)
\(5x< -40\)
\(\Rightarrow x< -8\)

\(1-2\left(x+1\right)\ge5\left(x-2\right)+2\)
\(\Leftrightarrow1-2x-2\ge5x-10+2\)
\(\Leftrightarrow-2x-5x\ge-10+2-1+2\)
\(\Leftrightarrow-7x\ge-7\)
\(\Leftrightarrow x\le1\)
\(\frac{3x+3}{3x-2}< 1\)
\(\Leftrightarrow\frac{3x-2+5}{3x-2}< 1\)
\(\Leftrightarrow1+\frac{5}{3x-2}< 1\)
\(\Leftrightarrow\frac{5}{3x-2}< 0\)
\(\Leftrightarrow3x-2< 0\)
\(\Leftrightarrow3x< 2\)
\(\Leftrightarrow x< \frac{2}{3}\)

Câu 1a : tự kết luận nhé
\(2\left(x+3\right)=5x-4\Leftrightarrow2x+6=5x-4\Leftrightarrow-3x=-10\Leftrightarrow x=\frac{10}{3}\)
Câu 1b : \(\frac{1}{x-3}-\frac{2}{x+3}=\frac{5-2x}{x^2-9}\)ĐK : \(x\ne\pm3\)
\(\Leftrightarrow x+3-2x+6=5-2x\Leftrightarrow-x+9=5-2x\Leftrightarrow x=-4\)
c, \(\frac{x+1}{2}\ge\frac{2x-2}{3}\Leftrightarrow\frac{x+1}{2}-\frac{2x-2}{3}\ge0\)
\(\Leftrightarrow\frac{3x+3-4x+8}{6}\ge0\Rightarrow-x+11\ge0\Leftrightarrow x\le11\)vì 6 >= 0
1) 2(x + 3) = 5x - 4
<=> 2x + 6 = 5x - 4
<=> 3x = 10
<=> x = 10/3
Vậy x = 10/3 là nghiệm phương trình
b) ĐKXĐ : \(x\ne\pm3\)
\(\frac{1}{x-3}-\frac{2}{x+3}=\frac{5-2x}{x^2-9}\)
=> \(\frac{x+3-2\left(x-3\right)}{\left(x-3\right)\left(x+3\right)}=\frac{5-2x}{\left(x-3\right)\left(x+3\right)}\)
=> x + 3 - 2(x - 3) = 5 - 2x
<=> -x + 9 = 5 - 2x
<=> x = -4 (tm)
Vậy x = -4 là nghiệm phương trình
c) \(\frac{x+1}{2}\ge\frac{2x-2}{3}\)
<=> \(6.\frac{x+1}{2}\ge6.\frac{2x-2}{3}\)
<=> 3(x + 1) \(\ge\)2(2x - 2)
<=> 3x + 3 \(\ge\)4x - 4
<=> 7 \(\ge\)x
<=> x \(\le7\)
Vậy x \(\le\)7 là nghiệm của bất phương trình
Biểu diễn
-----------------------|-----------]|-/-/-/-/-/-/>
0 7

nhiều thế
a) \(\frac{5x-2}{2}\ge\frac{3-x}{3}\Leftrightarrow\frac{3\left(5x-2\right)}{6}\ge\frac{2\left(3-x\right)}{6}\Leftrightarrow15x-6\ge6-2x\Leftrightarrow x\ge\frac{12}{17}\)
0 [ 12/17

a) Tập hợp nghiệm: S = {x/x < 4}
b) Tập hợp nghiệm: S = {x/x ≤ -2}
c) Tập hợp nghiệm: S = {x/x > -3}
d) Tập hợp nghiệm: S = {x/x ≥ 1}


\(x^2-4x+3\ge0\)
\(\left(x-1\right)\left(x-3\right)\ge0\)
TH1; X-1>=0 VA X-3>=0
TH2: X-1=<0 VA X-3<=0
Vay x>=3 hoac x<=1