Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a) Ta có: \(\frac{\left(2x+1\right)^2}{5}-\frac{\left(x-1\right)^2}{3}=\frac{7x^2-14x-5}{15}\)
\(\Leftrightarrow\frac{\left(2x+1\right)^2\cdot3}{15}-\frac{5\left(x-1\right)^2}{15}-\frac{7x^2-14x-5}{15}=0\)
\(\Leftrightarrow3\left(4x^2+4x+1\right)-5\left(x^2-2x+1\right)-7x^2+14x+5=0\)
\(\Leftrightarrow12x^2+12x+3-5x^2+10x-5-7x^2+14x+5=0\)
\(\Leftrightarrow36x+3=0\)
\(\Leftrightarrow36x=-3\)
\(\Leftrightarrow x=\frac{-3}{36}\)
Vậy: \(x=\frac{-3}{36}\)
b) Ta có: \(\frac{201-x}{99}+\frac{203-x}{97}=\frac{205-x}{95}+3=0\)
\(\Leftrightarrow\frac{201-x}{99}+\frac{203-x}{97}-\frac{205-x}{95}-3=0\)
\(\Leftrightarrow\left(\frac{201-x}{99}+1\right)+\left(\frac{203-x}{97}+1\right)+\left(\frac{205-x}{95}+1\right)=0\)
\(\Leftrightarrow\frac{201-x+99}{99}+\frac{203-x+97}{97}+\frac{205-x+95}{95}=0\)
\(\Leftrightarrow\frac{300-x}{99}+\frac{300-x}{97}+\frac{300-x}{95}=0\)
\(\Leftrightarrow\left(300-x\right)\left(\frac{1}{99}+\frac{1}{97}+\frac{1}{95}\right)=0\)
Vì \(\frac{1}{99}+\frac{1}{97}+\frac{1}{95}\ne0\)
nên 300-x=0
\(\Leftrightarrow x=300\)
Vậy: x=300
c) Ta có: \(x^3+x^2+x+1=0\)
\(\Leftrightarrow x^2\left(x+1\right)+\left(x+1\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(x^2+1\right)=0\)(1)
Ta có: \(x^2\ge0\forall x\)
\(\Rightarrow x^2+1\ge1\ne0\forall x\)(2)
Từ (1) và (2) suy ra x+1=0
hay x=-1
Vậy: x=-1
d) Ta có: \(\left(x-1\right)x\left(x+1\right)\left(x+2\right)=24\)
\(\Leftrightarrow\left(x^2+x\right)\left(x^2+x-2\right)=24\)
Đặt \(x^2+x-1=t\)
\(\Leftrightarrow\left(t+1\right)\left(t-1\right)=24\)
\(\Leftrightarrow t^2-1-24=0\)
\(\Leftrightarrow t^2-25=0\)
\(\Leftrightarrow\left(t-5\right)\left(t+5\right)=0\)
\(\Leftrightarrow\left(x^2+x-1-5\right)\left(x^2+x-1+5\right)=0\)
\(\Leftrightarrow\left(x^2+x-6\right)\left(x^2+x+4\right)=0\)
\(\Leftrightarrow\left(x^2+3x-2x-6\right)\left(x^2+2\cdot x\cdot\frac{1}{2}+\frac{1}{4}+\frac{15}{4}\right)=0\)
\(\Leftrightarrow\left(x+3\right)\left(x-2\right)\left[\left(x+\frac{1}{2}\right)^2+\frac{15}{4}=0\right]\)(3)
Ta có: \(\left(x+\frac{1}{2}\right)^2\ge0\forall x\)
\(\Rightarrow\left(x+\frac{1}{2}\right)^2+\frac{15}{4}\ge\frac{15}{4}\ne0\forall x\)(4)
Từ (3) và (4) suy ra
\(\left[{}\begin{matrix}x+3=0\\x-2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=2\end{matrix}\right.\)
Vậy: \(x\in\left\{-3;2\right\}\)
e) Ta có: \(\left(5x-3\right)-\left(4x-7\right)=0\)
\(\Leftrightarrow5x-3-4x+7=0\)
\(\Leftrightarrow x+4=0\)
\(\Leftrightarrow x=-4\)
Vậy: x=-4
f) Ta có: \(3x^2+2x-1=0\)
\(\Leftrightarrow3x^2+3x-x-1=0\)
\(\Leftrightarrow3x\left(x+1\right)-\left(x+1\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(3x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+1=0\\3x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-1\\3x=1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=\frac{1}{3}\end{matrix}\right.\)
Vậy: \(x\in\left\{-1;\frac{1}{3}\right\}\)
g) Ta có: \(x^2+6x-16=0\)
\(\Leftrightarrow x^2-2x+8x-16=0\)
\(\Leftrightarrow x\left(x-2\right)+8\left(x-2\right)=0\)
\(\Leftrightarrow\left(x-2\right)\left(x+8\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-2=0\\x+8=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-8\end{matrix}\right.\)
Vậy: \(x\in\left\{2;-8\right\}\)
h) Ta có: \(x^2+3x-10=0\)
\(\Leftrightarrow x^2+5x-2x-10=0\)
\(\Leftrightarrow x\left(x+5\right)-2\left(x+5\right)=0\)
\(\Leftrightarrow\left(x+5\right)\left(x-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+5=0\\x-2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-5\\x=2\end{matrix}\right.\)
Vậy: \(x\in\left\{-5;2\right\}\)
i) Ta có: \(x^2+x-2=0\)
\(\Leftrightarrow x^2-x+2x-2=0\)
\(\Leftrightarrow x\left(x-1\right)+2\left(x-1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(x+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\x+2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-2\end{matrix}\right.\)
Vậy: \(x\in\left\{1;-2\right\}\)
k) Ta có: \(3x^2+7x+2=0\)
\(\Leftrightarrow3x^2+6x+x+2=0\)
\(\Leftrightarrow3x\left(x+2\right)+\left(x+2\right)=0\)
\(\Leftrightarrow\left(x+2\right)\left(3x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+2=0\\3x+1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-2\\3x=-1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-2\\x=\frac{-1}{3}\end{matrix}\right.\)
Vậy: \(x\in\left\{-2;\frac{-1}{3}\right\}\)
l) Ta có: \(4x^2-12x+5=0\)
\(\Leftrightarrow4x^2-2x-10x+5=0\)
\(\Leftrightarrow2x\left(2x-1\right)-5\left(2x-1\right)=0\)
\(\Leftrightarrow\left(2x-1\right)\left(2x-5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}2x-1=0\\2x-5=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}2x=1\\2x=5\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\frac{1}{2}\\x=\frac{5}{2}\end{matrix}\right.\)
Vậy: \(x\in\left\{\frac{1}{2};\frac{5}{2}\right\}\)

\(a,\left(2x^2+1\right)+4x>2x\left(x-2\right)\)
\(\Leftrightarrow2x^2+1+4x>2x^2-4x\)
\(\Leftrightarrow4x+4x>-1\)
\(\Leftrightarrow8x>-1\)
\(\Leftrightarrow x>-\frac{1}{8}\)
\(b,\left(4x+3\right)\left(x-1\right)< 6x^2-x+1\)
\(\Leftrightarrow4x^2-4x+3x-3< 6x^2-x+1\)
\(\Leftrightarrow4x^2-x-3< 6x^2-x+1\)
\(\Leftrightarrow4x^2-6x^2< 1+3\)
\(\Leftrightarrow-2x^2< 4\)
\(\Leftrightarrow x^2>2\)
\(\Leftrightarrow x>\pm\sqrt{2}\)

\(\left(3x-2\right)\left(4x+5\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}3x-2=0\\4x+5=0\end{cases}\Leftrightarrow}\orbr{\begin{cases}x=\frac{2}{3}\\x=-\frac{5}{4}\end{cases}}\)
ĐKXĐ: x khác -4;-5;-6;-7
\(\frac{1}{x^2+9x+20}+\frac{1}{x^2+11x+30}+\frac{1}{x^2+13x+42}=\frac{1}{18}\)
\(\Rightarrow\frac{1}{\left(x+4\right).\left(x+5\right)}+\frac{1}{\left(x+5\right).\left(x+6\right)}+\frac{1}{\left(x+6\right).\left(x+7\right)}=\frac{1}{18}\)
\(\Rightarrow\frac{1}{x+4}-\frac{1}{x+5}+\frac{1}{x+5}-\frac{1}{x+6}+\frac{1}{x+6}-\frac{1}{x+7}=\frac{1}{18}\)
\(\Rightarrow\frac{1}{x+4}-\frac{1}{x+7}=\frac{1}{18}\)
\(\Rightarrow\frac{x+7-x-4}{\left(x+4\right).\left(x+7\right)}=\frac{1}{18}\Rightarrow3.18=x^2+11x+28\)
\(\Rightarrow x^2+11x-26=0\)
\(\Rightarrow\left(x-2\right).\left(x+13\right)=0\)
\(\Rightarrow\orbr{\begin{cases}x=2\\x=-13\end{cases}\left(tm\right)}\)
Vậy...

Bài làm
~ Bạn Thủy bên dưới có vẻ bị Lag mạnh, bài dễ như này mà cũng dùng denta với đen tiếc. Đéo biết làm thì đừng làm chứ đéo phải làm cái kiểu mà lớp 8 chưa học nhé bạn >.<, câu c dòng thứ hai với dòng thứ 3 không phải là thừa sao? đã vậy câu c làm sai đề nữa, bên trên là 1 - 5x. bên dưới là 1 + 5x . câu cuối cũng sai hằng đẳng thức, phải là +16x chứ hông phỉa -16x.~
a) 2x + 5 = 20 - 3x
<=> 2x + 3x = 20 + 5
<=> 5x = 25
<=> x = 5
Vậy x = 5 là nghiệm phương trình.
b) 4x2 + 5x = 0
<=> x( 4x + 5 ) = 0
<=> \(\orbr{\begin{cases}x=0\\4x+5=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=0\\x=-\frac{5}{4}\end{cases}}}\)
Vậy S = { 0; -5/4 }
c) \(\left(x-2\right)^2=1-5x\)
<=> \(x^2-4x+4=1-5x\)
<=> x2 - 4x + 5x - 1 + 4 = 0
<=> x2 + x + 3 = 0
<=> \(x^2+x.2.\frac{1}{2}+\frac{1}{4}+\frac{11}{4}=0\)
<=> \(\left(x^2+x+\frac{1}{4}\right)=-\frac{11}{4}\)
\(\Leftrightarrow\left(x+\frac{1}{2}\right)^2=-\frac{11}{4}\)( vô lí )
Vậy phương trình vô nghiệm.
d) x2 + 5x + 6 = 0
<=> x2 + 2x + 3x + 6 = 0
<=> x( x + 2 ) + 3( x + 2 ) = 0
<=> ( x + 3 )( x + 2 ) = 0
<=> \(\orbr{\begin{cases}x+3=0\\x+2=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=-3\\x=-2\end{cases}}}\)
Vậy tập nghiệm phương trình S = { -3; -2 }
e) x4 - 5x2 + 4 = 0
<=> x4 - x2 - 4x2 + 4 = 0
<=> x2( x2 - 1 ) - 4( x2 - 1 ) = 0
<=> ( x2 - 1 )( x2 - 4 ) = 0
<=> ( x - 1 )( x + 1 )( x - 2 )( x + 2 ) = 0
<=> \(\orbr{\begin{cases}x-1=0\\x+1=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=1\\x=-1\end{cases}}}\)
\(\orbr{\begin{cases}x-2=0\\x+2=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=2\\x=-2\end{cases}}}\)
Vậy tập nghiệm phương trình S = { 1; -1; 2; -2 }
f) 5( x2 - 3x ) = ( 4x + 2 )2 + 1
<=> 5x2 - 15x = 16x2 + 16x + 4 + 1
<=> 5x2 - 16x2 - 15x - 16x - 4 - 1 = 0
<=> -11x2 - 31x - 5 = 0
<=> -( 11x2 + 31x + 5 ) = 0
Ta có:( 11x2 + 31x + 5 ) > 0 V x
=> -( 11x2 + 31x + 5 ) < 0 V x
=> -( 11x2 + 31x + 5 ) = 0 ( vô lí )
Vậy phương trình vô nghiệm.
a, \(2x+5=20-3x\)
\(2x+5-20+3x=0\)
\(5x-15=0\Leftrightarrow5x=15\Leftrightarrow x=3\)
b, \(4x^2+5x=0\)
\(x\left(4x+5\right)=0\)
\(x=0\)
\(4x+5=0\Leftrightarrow4x=-5\Leftrightarrow x=-\frac{5}{4}\)
c, \(\left(x-2\right)^2=1-5x\)
\(\left(x-2\right)=\pm\sqrt{1-5x}\)
\(x-2=\sqrt{1+5x}\)
\(x^2-4x+4=1+5x\)
\(x^2-4x+4-1-5x=0\)
\(x^2-9x+3=0\)
\(\Delta=b^2-4ac=\left(-9\right)^2-4.3.1=81-12=69>0\)
Nên pt có 2 nghiệm phân biệt
\(x_1=\frac{9-\sqrt{69}}{2.1}=\frac{9-\sqrt{69}}{2}\)
\(x_2=\frac{9+\sqrt{69}}{2.1}=\frac{9+\sqrt{69}}{2}\)

Bạn đăng từng câu một thì sẽ có người giúp bạn đấy!
Tick cho mình nhé!

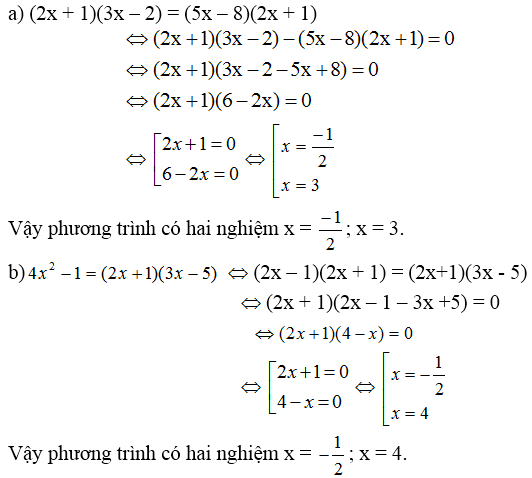
a)(2x+1)(3x-2)=(5x-8)(2x+1)
⇔(2x+1)(3x-2)-(5x-8)(2x+1)=0
⇔(2x+1)(3x-2-5x+8)=0
⇔(2x+1)(-2x+6)=0
⇔2x+1=0 hoặc -2x+6=0
1.2x+1=0⇔2x=-1⇔x=-1/2
2.-2x+6=0⇔-2x=-6⇔x=3
phương trình có 2 nghiệm x=-1/2 và x=3

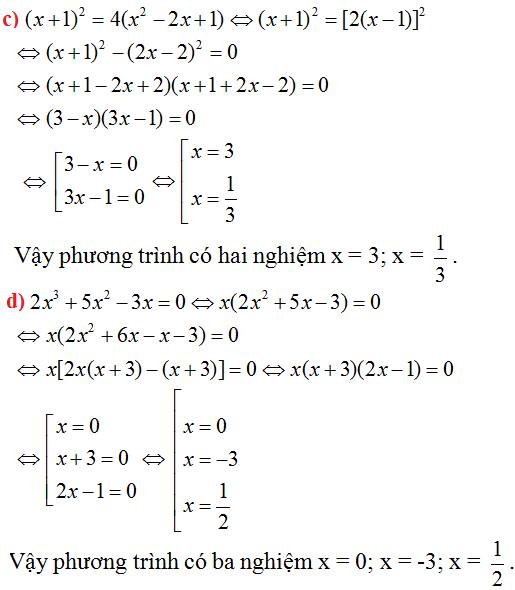
a: =>(x-2)(2x+5)=0
=>x=2 hoặc x=-5/2
c: \(\dfrac{2x}{x-1}-\dfrac{x}{x+1}=1\)
=>\(\dfrac{2x^2+2x-x^2+x}{x^2-1}=1\)
=>x^2+3x=x^2-1
=>3x=-1
=>x=-1/3