Câu 2.Cho biểu thức:

a) Rút gọn biểu thức P.
b) Tính giá trị của P khi 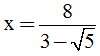 .
.
c) Tìm x để P có giá trị là số tự nhiên.
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Để biến một thanh thép thành một nam châm vĩnh cửu ta đặt thanh vào trong lòng ống dây, rồi cho dòng điện một chiều chạy qua.
=>Chọn A
Chọn câu C
vì Đặt thanh thép vào trong lòng ống dây dẫn có dòrng điện một chiều chạy q

a, Thay m = 7 ta được y = x + 7 - 1 = x + 6
Hoành độ giao điểm tm pt
\(x^2-x-6=0\)
\(\Delta=1-4\left(-6\right)=1+24=25>0\)
Vậy pt có 2 nghiệm pb
hay (P) cắt (D) tại 2 điểm pb

Với mọi số thực \(a_i\) , ta có:
\(\left(a_1-a_2\right)^2+\left(a_2-a_3\right)^2+...+\left(a_n-a_1\right)^2\ge0\)
\(\Leftrightarrow2\left(a_1^2+a_2^2+a_3^2+...+a_n^2\right)\ge2\left(a_1a_2+a_2a_3+...+a_na_1\right)\)
\(\Leftrightarrow a_1^2+a_2^2+...+a_n^2\ge a_1a_2+a_2a_3+...+a_na_1\) (đpcm)
ừa ae
(a1 - a2)2 + (a2 - a3)2 + ...+(ar - a1) \(\ge\) 0
\( \Leftrightarrow \) 2 (a12 + a22 + ...+ an2 ) \(\ge\) 2 ( a1 a2 + a2 a3 +...+ an a1 )
\( \Leftrightarrow\) a12 + a22+...+ an2 \(\ge\) a1 a2 + a2 a3 +...+ an a1 (ĐPCM)

Với mọi a;b;c;d;e ta có:
\(\left(a-2b\right)^2+\left(a-2c\right)^2+\left(a-2d\right)^2+\left(a-2e\right)^2\ge0\)
\(\Leftrightarrow4a^2+4b^2+4c^2+4d^2+4e^2\ge4ab+4ac+4ad+4ae\)
\(\Leftrightarrow a^2+b^2+c^2+d^2+e^2\ge a\left(b+c+d+e\right)\) (đpcm)
Dấu "=" xảy ra khi \(\dfrac{a}{2}=b=c=d=e\)
BĐT
\(\Leftrightarrow4a^2+4b^2+4c^2+4d^2+4e^2\ge4a\left(b+c+d+e\right)\)
\(\Leftrightarrow4a^2+4b^2+4c^2+4d^2+4e^2\ge4ab+4ac+4ad+4ae\)
\(\Leftrightarrow4a^2+4b^2+4c^2+4d^2+4e^2-\left(4ab+4ac+4ad+4ae\right)\ge0\)
\(\Leftrightarrow a^2-4ab+4b^2+a^2-4ac+4c^2+a^2-4ad+4d^2+a^2-4ae+4e^2\ge0\)
\(\Leftrightarrow\left(a-2b\right)^2+\left(a-2c\right)^2+\left(a-2d\right)^2+\left(a-2e\right)^2\ge0\), luôn đúng với \(\forall a,b,c,d,e\in R\)
Dấu "=" xảy ra khi và chỉ khi \(a=2b=2c=2d=2e\)

a, Với x >= 0 ; x khác 16
\(A=\left(\frac{x+5\sqrt{x}-27+\left(3-\sqrt{x}\right)\left(\sqrt{x}+4\right)}{x-16}\right):\frac{1}{\sqrt{x}+4}\)
\(=\left(\frac{x+5\sqrt{x}-27+3\sqrt{x}+12-x-4\sqrt{x}}{x-16}\right):\frac{1}{\sqrt{x}+4}\)
\(=\left(\frac{4\sqrt{x}-15}{x-16}\right):\frac{1}{\sqrt{x}+4}=\frac{4\sqrt{x}-15}{\sqrt{x}-4}\)
b, Ta có \(B=-2A\Rightarrow\sqrt{x}-4=-\frac{8\sqrt{x}-30}{\sqrt{x}-4}\)
\(\Leftrightarrow x-8\sqrt{x}+16=-8\sqrt{x}+30\Leftrightarrow x-14=0\Leftrightarrow x=14\left(tm\right)\)

`(1+\frac{1}{x})^3.(1+x)^3=16`
`<=>(2+x+\frac{1}{x})^3=16`
`<=>2+x+\frac{1}{x}=\root{3}{16}`
`<=>x+\frac{1}{x}-(\root{3}{16}-2)=0`
`=>x^2-(\root{3}{16}-2)+1=0`
`<=>x^2-2.x.\frac{\root{3}{16}-2}{2}+\frac{\root{\frac{3}{2}}{16}-2}{4}+(1-\frac{\root{\frac{3}{2}}{16}-2}{4})=0`
`<=>(x-\frac{\root{3}{16}-2}{2})^2+(1-\frac{\root{\frac{3}{2}}{16}-2}{4})>0` (vô nghiệm)
Vậy phương trình vô nghiệm
ĐKXĐ: ...
\(\dfrac{\left(1+x\right)^3\left(1+x\right)\left(x^2-x+1\right)}{x^3}=16\)
\(\Leftrightarrow\left(\dfrac{\left(1+x\right)^2}{x}\right)^2\left(\dfrac{x^2-x+1}{x}\right)=16\)
\(\Leftrightarrow\left(x+\dfrac{1}{x}+2\right)^2\left(x+\dfrac{1}{x}-1\right)=16\)
Đặt \(x+\dfrac{1}{x}+2=t\)
\(\Rightarrow t^2\left(t-3\right)=16\Rightarrow t^3-3t^2-16=0\)
\(\Leftrightarrow\left(t-4\right)\left(t^2+t+4\right)=0\)
\(\Leftrightarrow t=4\Rightarrow x+\dfrac{1}{x}+2=4\)
\(\Rightarrow x^2-2x+1=0\)
\(\Rightarrow x=1\)
ĐK: \(x\ge0,x\ne1,x\ne4\).
a) \(P=\left(\frac{2x+1}{\sqrt{x^3}-1}-\frac{\sqrt{x}}{x+\sqrt{x}+1}\right)\div\left(\frac{3}{\sqrt{x}-1}+\frac{2\sqrt{x}+5}{1-x}\right)\)
\(=\left(\frac{2x+1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}-\frac{\sqrt{x}\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\right)\div\left(\frac{3\left(\sqrt{x}+1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}-\frac{2\sqrt{x}+5}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\right)\)
\(=\frac{2x+1-x+\sqrt{x}}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\div\frac{3\sqrt{x}+3-2\sqrt{x}-5}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(=\frac{x+\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}.\frac{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{\sqrt{x}-2}\)
\(=\frac{\sqrt{x}+1}{\sqrt{x}-2}\)
\(x=\frac{8}{3-\sqrt{5}}=\frac{16}{6-2\sqrt{5}}=\frac{16}{5-2\sqrt{5}+1}=\left(\frac{4}{\sqrt{5}-1}\right)^2\)
\(\Rightarrow x=\sqrt{\left(\frac{4}{\sqrt{5}-1}\right)^2}=\left|\frac{4}{\sqrt{5}-1}\right|=\frac{4}{\sqrt{5}-1}\)
\(P=\frac{\sqrt{x}+1}{\sqrt{x}-2}=\frac{\frac{4}{\sqrt{5}-1}+1}{\frac{4}{\sqrt{5}-1}-2}=\frac{4+\sqrt{5}-1}{4-2\sqrt{5}+2}=\frac{3+\sqrt{5}}{6-2\sqrt{5}}=\frac{7+3\sqrt{5}}{4}\)
\(P=\frac{\sqrt{x}+1}{\sqrt{x}-2}=\frac{\sqrt{x}-2+3}{\sqrt{x}-2}=1+\frac{3}{\sqrt{x}-2}\)
Có \(-2\le\sqrt{x}-2< 0\)thì \(\frac{3}{\sqrt{x}-2}\le-\frac{3}{2}\)nên \(P\)không là số tự nhiên.
Suy ra \(\sqrt{x}-2>0\Leftrightarrow x>4\)
\(P\)là số tự nhiên thì \(\frac{3}{\sqrt{x}-2}\)là số tự nhiên suy ra \(\sqrt{x}-2=\frac{3}{n}\)(với \(n\)là số tự nhiên)
\(\Leftrightarrow x=\left(\frac{3}{n}+2\right)^2\).