\(0,5.\sqrt[]{100}-\sqrt[]{\dfrac{1}{2}+6,5}\)

\(=0,5.10-\sqrt[]{\dfrac{1}{2}+\dfrac{13}{2}}\)

\(=5-\sqrt[]{7}\)

21/2

Chiều dài hơn chiều rộng là: 10 cm 

Ta có sơ đồ: 

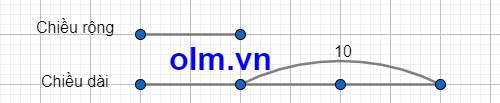
Theo sơ đồ ta có:

Chiều rộng là: 10: (3-2) \(\times\)  = 5 (cm)

Chiều dài là: 5 + 10 = 15  (cm)

Diện tích hình chữ nhật ban đầu là: 15 \(\times\) 5 = 75 (cm²)

Đs...

\(\left(2x-14\right):12=26\)

\(2x-14=26.12\)

\(2x-14=312\)

\(2x=312+14\)

\(2x=326\)

\(x=326:2\)

\(x=163\)

2x-14=26*12

2x-14=312 

2x=312-14

2x=298

x= 298:2

x=149

\(3^{30}:3^{27}=3^{30-27}=3^3=27\)

Vậy x=27

27

\(\left(x:32+15\right):4=24\)

\(x:32+15=24.4\)

\(x:32+15=96\)

\(x:32=96-15\)

\(x:32=81\)

\(x=81.32\)

\(x=2592\)

Sửa bài

\(\left(x:32+15\right):4=24\)

\(x:32+15=24.4\)

\(x:32+15=6\)

\(x:32=6-15\)

\(x:32=-9\)

\(x=\left(-9\right).32\)

\(x=-288\)

\(x+2y+xy=2\)

\(\Rightarrow x\left(1+y\right)+2y+2-2=2\)

\(\Rightarrow x\left(1+y\right)+2\left(y+1\right)=4\)

\(\Rightarrow\left(y+1\right)\left(x+2\right)=4\)

\(\Rightarrow\left(x+2\right);\left(y+1\right)\in\left\{-1;1;-2;2;-4;4\right\}\)

\(\Rightarrow\left(x;y\right)\in\left\{\left(-3;-5\right);\left(-1;3\right);\left(-4;-3\right);\left(0;1\right);\left(-6;-2\right);\left(2;0\right)\right\}\)

Số viên bi của Hùng bằng nhau Đức là :

\(780:2=390\left(viên\right)\)

Số viên bi của Hùng là :

\(390+\dfrac{1}{6}x390=455\left(viên\right)\)

Số viên bi của Đức là :

\(780-455=325\left(viên\right)\)

giải nhanh giúp mik nhé 5h nộp rồi 

\(\dfrac{1}{2}-\left|2-3x\right|=\sqrt{\dfrac{19}{16}}-\sqrt{\left(-0,75\right)^2}\\ \Rightarrow\dfrac{1}{2}-\left|2-3x\right|=\dfrac{\sqrt{19}}{4}-\dfrac{3}{4}\\ \Rightarrow\left|2-3x\right|=\dfrac{1}{2}-\dfrac{\sqrt{19}-3}{4}\)

\(\Rightarrow\left|2-3x\right|=\dfrac{5-\sqrt{19}}{4}\)

\(TH_1:x\le\dfrac{2}{3}\\ 2-3x=\dfrac{5-\sqrt{19}}{4}\\ \Rightarrow3x=\dfrac{3+\sqrt{19}}{4}\\ \Rightarrow x=\dfrac{3+\sqrt{19}}{12}\left(tm\right)\)

\(TH_2:x>\dfrac{2}{3}\\ 3x-2=\dfrac{5-\sqrt{19}}{4}\\ \Rightarrow3x=\dfrac{13-\sqrt{19}}{4}\\ \Rightarrow x=\dfrac{13-\sqrt{19}}{12}\left(tm\right)\)

Vậy \(x\in\left\{\dfrac{3+\sqrt{19}}{12};\dfrac{13-\sqrt{19}}{12}\right\}\)

\(\dfrac{1}{2}-\left|2-3x\right|=\sqrt[]{\dfrac{19}{16}}-\sqrt[]{\left(-0,75\right)^2}\)

\(\Rightarrow\dfrac{1}{2}-\left|2-3x\right|=\dfrac{\sqrt[]{19}}{4}-0,75\)

\(\Rightarrow\dfrac{1}{2}-\left|2-3x\right|=\dfrac{\sqrt[]{19}}{4}-\dfrac{3}{4}\)

\(\Rightarrow\left|2-3x\right|=\dfrac{1}{2}-\dfrac{\sqrt[]{19}}{4}+\dfrac{3}{4}\)

\(\Rightarrow\left|2-3x\right|=\dfrac{5-\sqrt[]{19}}{4}\)

\(\Rightarrow\left[{}\begin{matrix}2-3x=\dfrac{5-\sqrt[]{19}}{4}\\2-3x=\dfrac{-5+\sqrt[]{19}}{4}\end{matrix}\right.\)

\(\Rightarrow\left[{}\begin{matrix}3x=2-\dfrac{5-\sqrt[]{19}}{4}\\3x=2-\dfrac{\sqrt[]{19}-5}{4}\end{matrix}\right.\)

\(\Rightarrow\left[{}\begin{matrix}3x=\dfrac{3+\sqrt[]{19}}{4}\\3x=\dfrac{13-\sqrt[]{19}}{4}\end{matrix}\right.\)

\(\Rightarrow\left[{}\begin{matrix}x=\dfrac{3+\sqrt[]{19}}{12}\\x=\dfrac{13-\sqrt[]{19}}{12}\end{matrix}\right.\)

a. A B 4 cm b. M N a 8 cm

Chúc bạn học tốt

Xét hình thang ABCD ta có :

\(\widehat{A}+\widehat{B}+\widehat{C}+\widehat{D}=360^o\)

mà \(\left\{{}\begin{matrix}\widehat{B}+\widehat{D}=180^o\left(đề.bài\right)\\\widehat{B}+\widehat{A}=180^o\left(t/c.hình.thang\right)\end{matrix}\right.\)

\(\Rightarrow\widehat{C}=\widehat{D}\)

⇒ ABCD là hình thang cân (dpcm)

Ta có : AB // CD ⇒ \(\widehat{B}\) + \(\widehat{C}\) = 180^o mà \(\widehat{B}+\widehat{D}=\) 180^o ⇒ \(\widehat{D}=\widehat{C}\)

Vì AB // CD; \(\widehat{D}=\widehat{C}\) vậy ABCD là hình thang cân