Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(D=[4;+\infty)\)
Bất phương trình tương đương:
\(\frac{\sqrt{x-4}-\sqrt{x-1}}{\sqrt{x+4}-\sqrt{x+5}}>3\)
\(\Leftrightarrow\sqrt{x-4}+3\sqrt{x+5}>3\sqrt{x+4}+\sqrt{x-1}\)
\(\Leftrightarrow10x+41+6\sqrt{x^2+x-20}>10x+35+6\sqrt{x^2+3x-4}\)(Vì VP và VT dương)
\(\Leftrightarrow1+\sqrt{x^2+x-20}>\sqrt{x^2+3x-4}\)
\(\Leftrightarrow1+2\sqrt{x^2+x-20}+x^2+x-20>x^2+3x-4\)(Vì \(VT>VP\ge0\))
\(\Leftrightarrow2\sqrt{x^2+x-20}>2x+15\)
\(\Leftrightarrow2x+15< 0\left(h\right)\hept{\begin{cases}2x+15\ge0\\4\left(x^2+x-20\right)>4x^2+60x+225\end{cases}}\)
\(\Leftrightarrow x< -\frac{15}{2}\left(h\right)\hept{\begin{cases}x\ge-\frac{15}{2}\\x< -\frac{305}{56}\end{cases}}\Leftrightarrow x< -\frac{305}{56}\)
Kết hợp TXĐ thì BPT vô nghiệm.

Bài 1: Hàm số cho xác định trên R khi và chỉ khi:
\(\Delta'\le0\Leftrightarrow m^2-22m+120\le0\Leftrightarrow10\le m\le12\)
Vậy tổng các giá trị nguyên của m là \(33\)
Bài 2: Xét \(m=4\), bất phương trình vô nghiệm
Để bất phương trình cho vô nghiệm thì:
\(\hept{\begin{cases}m-4< 0\\\Delta'< 0\end{cases}}\Leftrightarrow\hept{\begin{cases}m< 4\\m-4< 0\end{cases}}\Leftrightarrow m< 4\)
Vậy \(m\le4\), số giá trị nguyên dương của m thỏa mãn đề là 4 giá trị.
Bài 3:
TH1: \(x< -1\)thì: \(-2x-2+3-x>3\Leftrightarrow x< -\frac{2}{3}\)suy ra \(x< -1\)
TH2: \(-1\le x\le3\)thì: \(2x+2+3-x>3\Leftrightarrow x>-2\)suy ra \(-1\le x\le3\)
TH3: \(x>3\)thì: \(2x+2+x-3>3\Leftrightarrow x>\frac{4}{3}\)suy ra \(x>3\)
Vậy \(S=R.\)

We............the seedlings into peaty soil
A. take
B. plough
C. raise
D. transplant
We............the seedlings into peaty soil
A. take
B. plough
C. raise
D. transplant

\(VT\ge\frac{9}{a+b+c}+\frac{\left(a+b+c\right)^2}{3}=\left(\frac{\left(a+b+c\right)^2}{3}+\frac{1}{3\left(a+b+c\right)}+\frac{1}{3\left(a+b+c\right)}\right)+\frac{25}{3\left(a+b+c\right)}\ge\frac{28}{3}\)Đẳng thức xảy ra khi \(a=b=c=\frac{1}{3}\)
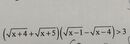
People have been.....their living from the sea for many, many years. They have found out a great number of valuable things and have shared what they learned with other people
A. earning. B.putting. C. leading. D. risking
(e k dám chắc nhé)